Why I think tension should be twice the force in a tug of war

Question

I'm going to provide my argument for why I think the tension in a rope should be twice the force exerted on either side of it.

First, let's consider a different example. Say there is a person named $\text{A}$ and a block in space. $\text{A}$ pushes on the block with a force of $100 \ \text{N}$. Then, the block will also push $\text{A}$ with a force of $100 \ \text{N}$ by Newton's third law. Now, consider the case where instead of the block, there is a person $\text{B}$ who is also pushing on $\text{A}$ with a force of $100 \ \text{N}$ while $\text{A}$ is pushing on him. $\text{A}$ will experience a force of $100 \ \text{N}$ because he pushed on $\text{B}$, AND another $100 \ \text{N}$ because he is being pushed by $\text{B}$. Hence, he will experience a force of $200 \ \text{N}$. Similarly, $\text{B}$ also experiences $200 \ \text{N}$ of force.

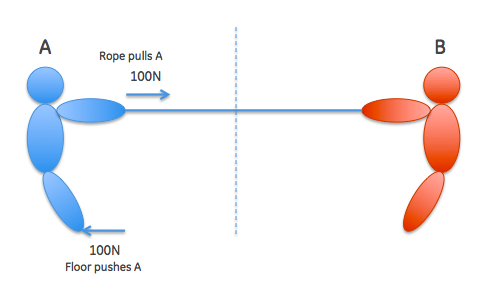
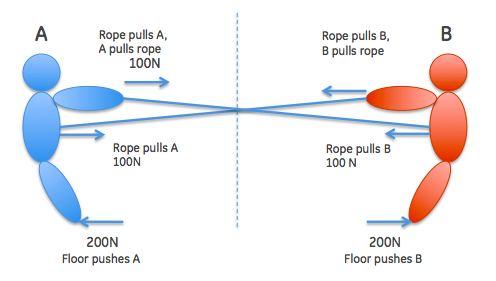
Now, back to the original problem. There are two people, $\text{A}$ and $\text{B}$, in space with a taut rope (no tension currently) in between them. If only $\text{A}$ is pulling and $\text{B}$ is not, then I agree that the tension is equal to the force $\text{A}$ exerts. This situation (in my opinion) becomes analogous to the above if $\text{B}$ is also pulling. So, say both of them pull from either side with a force of $100 \ \text{N}$. Then, the rope at the end of $\text{B}$ will pull $\text{B}$ with a force of $100 \ \text{N}$ (this pull is caused by $\text{A}$). By Newton's third law, the rope will experience a pull of $100 \ \text{N}$. But $\text{B}$ is also pulling his end of the rope with $100 \ \text{N}$. Therefore, the tension should be $200 \ \text{N}$. Similarly, the end of the rope at $\text{A}$ must pull $\text{A}$ with $100 \ \text{N}$ of force (because $\text{B}$ is pulling from the other side) and hence experience a force of $100 \ \text{N}$ itself by Newton's third law plus another $100 \ \text{N}$ because $\text{A}$ is pulling on the rope.

Apparently, the answer is not this (according to my search on the web). So, could anyone tell me why this reasoning is wrong? Thanks.

EDIT: So, apparently, people don't agree with my first example, leave alone the second. This is to the downvoters and the upvoters of the highest-rated answer: You all agree that if only $\text{A}$ pushes $\text{B}$ with a force of $100 \ \text{N}$, then $\text{A}$ and $\text{B}$ both will get pushed by a force of $100 \ \text{N}$ in opposite directions, right? Then, in the case where $\text{B}$ is also pushing with a force of $100 \ \text{N}$, it doesn't make sense that the answer would be exactly the same. It doesn't seem right that no matter what $\text{B}$ does, $\text{B}$ and $\text{A}$ will always experience the same force as they would have if $\text{B}$ hadn't applied any force.

EDIT 2: I'm going to provide here a link to a question that I posted: Two people pushing off each other. According to the answer and the comments there, the reason as to why my first example is incorrect is different to the one provided here. So maybe you should all read the answer and the comments provided by the person and reconsider what you think.

Answer 1

It is always best to draw a diagram to convince yourself of things in a case like this.

This is intended to represent a steady state situation: nobody is moving / winning. As you can see, there are two horizontal forces on A: the floor (pushing with 100N) and the rope (pulling with 100N). There will be two vertical forces (gravity pulling down on center of mass, and ground pulling up) to balance the torques - I did not show them because they are not relevant to the answer.

Now I drew a dotted line between A and B. Consider this a curtain. A cannot see whether the rope is attached to B (an opponent) or a wall. A can measure the tension in the rope by looking (for instance) at the speed at which a wave travels along the rope - or by including a spring gage.

Now ask yourself this question: if A feels a tension of 100N in the rope (this is the definition of the force on A), and can confirm (by looking at the gage) that the tension is 100 N, but he cannot see whether the rope is attached to a ring or to an opponent, then how can the tension be 200N? If I pull on a gage with a force of 100N, it will read 100N - it cannot read anything else (in a static situation, and where the gage is massless, ... )

I think I understand the source of your confusion based on the earlier q/a that you referenced - so let me draw another diagram:

In this diagram, I have move the point of attachment of the rope with which A pulls B away from B's hands, to his waist. Similarly, the rope with which B pulls on A is moved to A's waist.

What happens? Now there are two distinct points where A experiences a force of 100 N: one, his hands (where he is pulling on the rope attached to B's waits); and another where the rope that B is pulling on is tied around his waist.

The results is that there are two ropes with a tension of 100N each, that together result in a force of 200N on A (two ropes) offset by a force of 200N from the floor, etc.

This is NOT the same thing as the first diagram, where the point on which B's rope is attached is the hands of A - there is only a single line connecting A and B with a tension of 100 N in that case.

As was pointed out in comments, you can put a spring gauge in series with your rope to measure the tension in it; and now the difference between "a single person pulling on a rope attached to a ring at the wall (taken to be the dotted line) and two people pulling across a curtain (so they cannot see what they are doing) is that in one case, a single spring (with spring constant $k$) expands by a length $l$, while in the second case you find a spring that's twice as long, with constant $k/2$), expanding by $2l$.

These are all different ways to look at the same thing.

Answer 2

Your first example is facetious. If each is providing 100N then each is feeling 100N, period. In order to feel 200N, each would have to provide 200N. This is what Newton's Laws of Motion are all about; one does not feel their own force, only external forces, or when their own force comes into contact with an external body.

Answer 3

I think the greatest confusion here is forgetting that there are no lone forces in the world, there are only third law pairs (as in Newton's third law). Yes the person pulls on the rope with 100N, but the string is pulling back with 100N. The floor pushes on the foot with 100N, and the foot pushes on the floor with 100N. In reality, the way to measure force is to insert a scale. This measures the force between two objects. Now just eliminate the rope altogether. First let person A and person B push on the same scale from opposite sides. When the scale reads 100N, they are each pushing with 100N. Now let them pull on the same spring scale (from opposite sides). When the spring reads 100N, they are each pulling with 100N. As @floris pointed out, as far as either of them are concerned, there could be another person pulling on the other end of the spring scale, or it could be attached to a wall. It matters not. And, we can always replace the spring scale with a rope. The spring scale just measures the tension

Maybe it's even more intuitive to make the scenario vertical. Person A holds onto a spring scale that hangs down. The scale has a 100N weight hanging from it, so the scale reads 100N. Clearly, person A is pulling up with 100N (of course person A and the spring are pulling on each other with 100N). Person A now closes her eyes. She can no longer tell if the weight is still there, or if person B is now pulling down on the spring with 100N of force. See? Persons A and B can each be pulling on the spring with 100N of force, and the scale just reads 100N. Take away the scale and insert a rope. The physics does not change.

Answer 4

No.

Your mistake is that the block also pushes back on A, with the same 100 N that A is pushing with, by Newton's third law. Assuming both weigh 100 kg, both the block and the person will accelerate at 1 m/s$^2$ for the duration of the push. If there's two people pushing on each other, then clearly they will also accelerate at 1 m/s$^2$ so the forces must be the same. (If this isn't obvious, consider placing a very massive, very thin, very strong wall between them, which can't alter the physics. Then it's just two 100 kg people pushing off a stiff wall with 100 N.)

The difference between the two scenarios is that in the first one the reaction force is provided by the block's structure, while in the second one by a work-performing human. In the first one the block goes away while in the second one A and B's palms stay stationary. Thus A is able to push for twice as long and therefore do ~twice the work, so they will - as intuition says - end up going faster.

This translates directly to the rope-pulling scenario, simply substituting tension for the compression force at the guys' palms.

Answer 5

After some research, I decided to edit this answer and I hope to finally clarify why tension shouldn't be 0N or 200N when an external force of 100N is applied to the cable at each end.

I would say that historically Hooke decided to use only the force at one end of the spring (e.g. 100N) when establishing his famous law probably for simplicity and considering we are more interested in the force that this element (spring, cable, etc.) is exerting on each body.

He essentially would attach successively increasing weights to a spring (for example fixed to the ceiling). For each weight, he would measure the displacement of the spring and he noticed a pattern: the displacement would increase proportionally with the increase of force generated by the weight. Since he was dealing with a static scenario (he would let the spring reach its new balanced position after increasing the weight), he knew that a reaction force, equal in magnitude to the weight force, was being applied at the other end of the spring (this is the reaction from the ceiling). He could say the total force on the spring was zero, however, we can clearly see that even though the spring is in static equilibrium, it is stretched, meaning there is some internal action going on. Considering $F=0$ isn't useful to describe what's happening to the system internally. Now let's suppose that he expressed the proportionality law in terms of the two forces, i.e., instead of saying:

$$F=kx$$

(Where F is the force applied at one end of the spring, i.e. 100N), he could have assumed that the internal force was actually the double ($F^*=2F$) since at the other end of the spring there is always a reaction force of the same magnitude:

$$F^*=k^*x$$

This would simply affect the spring constant (and consequently Young's modulus by a factor of 2). Seemingly one would think that this would just change the Force vs Displacement or Stress vs Strain plots by a factor of two. This way we would interpret this plots as showing a failure strength when two forces of equal magnitude are applied at each side of the cable for example.

Note that the stiffness using this definition can be interpreted as the double of the old stiffness $k$ (for a given displacement). $$\frac{F^*}{F}=\frac{k^*x}{kx}=\frac{k^*}{k}=2 $$ However, as one would expect, since a lot of mathematics was built upon Hooke's law there are probably a lot of reasons why to say that the material "feels", internally, a force of 100N and not 200N.

One example where defining $T = 200N$ would be inconsistent is in the case of a dynamically loaded cable. Let's say the cable has mass. The argument is based on this great answer regarding beam displacement: https://physics.stackexchange.com/a/222318/46464 Let's start with the following schematic ($F=100N$ is the externally applied force at the end of the cable):

Experimentally you would see that due to elasticity, the cable at the tip you are pulling would stretch more than on the other side. The logic here is that if you make an imaginary cut on the cable, infinitesimally close to the tip, the molecules from the right side would exert a force $T(x)$ equal to the external force, as a reaction. However as $x$ increases, it will reach a point where the molecules don't feel a force anymore, so they don't react on the molecules from the left block $T(x=L)=0$

$$T = F\left(1-\frac{x}{L}\right)$$

As you can see it would be incoherent to use tension as 200N at the tip, and indeed further complications would arise on displacement calculations because now you are dealing with a stiffness $k^*$.

Answer 6

simple easy answer, if you are pulling the rope with your maximum effort of 100 and the rope is pulling you with 101 then you will be pulled across the line and lose, so did your opponent win by applying a force of 1 ?

Answer 7

This is a lovely question, as it poses an obvious common sense interpretation that is at odds with our physical models, specifically do to with the difference between a passive reaction to a force and an active one; intuitively one expects there to be a quantitative difference between their magnitudes, when in fact they are the same.

Consider three situations. In one a weight of 10kg sits on a set of bathroom scales of unsprung mass 1kg which is on the floor. In the second the same weight sits on the same scales but the scales are held in the air by a human maintaining a constant upward force. In the third the scales alone are in outer space and are accelerated by a force of 100N pressing on the plate. What reading is shown on the scale in each of these scenarios?

The answer is that they all show 10kg, even though two of them involve something else pushing back on the scales and one doesn't.

Answer 8

To keep things simple lets say the tug of war rope is stationary, both sides are pulling with equal force.

A and B are both people, A pulls left with 100N, B pulls right with 100N.

Now in your second example we replace B with C, your immovable weight.

A pulls left with 100N. Newtons law says that the block must be pulling right with 100N, otherwise the system would move.

The total strain on the rope is exactly the same in both cases.

To make it more complicated lets have A, B and C all in play:

A
         C
B

The rope runs from A, to C, through a pulley and then on to B.

A and B both pull with 100N. C experiences a force similar to 200N (reduced a bit as A and B are in slightly different directions, however each length of the rope still experiences a force of 100N.

Now if you have a single length of rope, and both A and B pull on it now you finally have your 200N of force in the rope, because now A and B are pulling for a combined force of 200N so the counter-force from C increases to match it, otherwise the whole system will start moving.

Answer 9

Let's try one more! Let's attach a rope to the ceiling and suspend a $10 \ \text{kg}$ weight.

The tension force on the rope is $$\text{Tension force} = ma = 10 \ \text{kg} \times 10 \ \text{m/s}^2 = 100 \ \text{Newtons}.$$ With a $20 \ \text{kg}$ weight:
$$\text{Tension force} = 20 \ \text{kg} \times 10 \ \text{m/s}^2 = 200 \ \text{Newtons}.$$

Now, attach a simple pulley to the ceiling and put $10 \ \text{kg}$ on either end:

$$\text{Tension force on the rope} = (10 \ \text{kg} + 10 \ \text{kg}) \times 10 \ \text{m/s}^2 = 200 \ \text{Newtons}.$$

We've created a "dumb waiter" apparatus. Now, to "win" the tug of war, put one more pound on either end.

And another!

Attach a $10 \ \text{kg}$ weight to the ceiling of a rocket on the launch pad.

$$\text{Tension force on the rope} = 10 \ \text{kg} \times 10 \ \text{m/s}^2 = 100 \ \text{Newtons}.$$

Now accelerate to rocket upwards at $10 \ \text{m/s}^2$.

$$\text{Tension force on the rope} = 10 \ \text{kg} \times (10 + 10) \ \text{m/s}^2 = 200 \ \text{Newtons} \quad \text{or} \quad 2 G.$$

Answer 10

tension will be equal to force of one side. because wen a string is attach with a support, and other end is attached wit mass we take tension is equal to weight. do you think there are only two forces tension and weight? no, there are 3 forces, 1. weight downward tension upwards, another force of support upward, this is same as rope is pulling from either side by same forces. so tension is equal to 1 forces, if forces are same.