1
$\begingroup$

This is a long post, but only because I have explained the scenario very very clearly,90 percent of this post is explaining you a simple situation,my doubt is only 5-6 lines at the very end of this post,so please bear the patience and please help me

Imagine a scenario where 2 people are pulling with equal forces of 100N on a rope of some mass M enter image description here

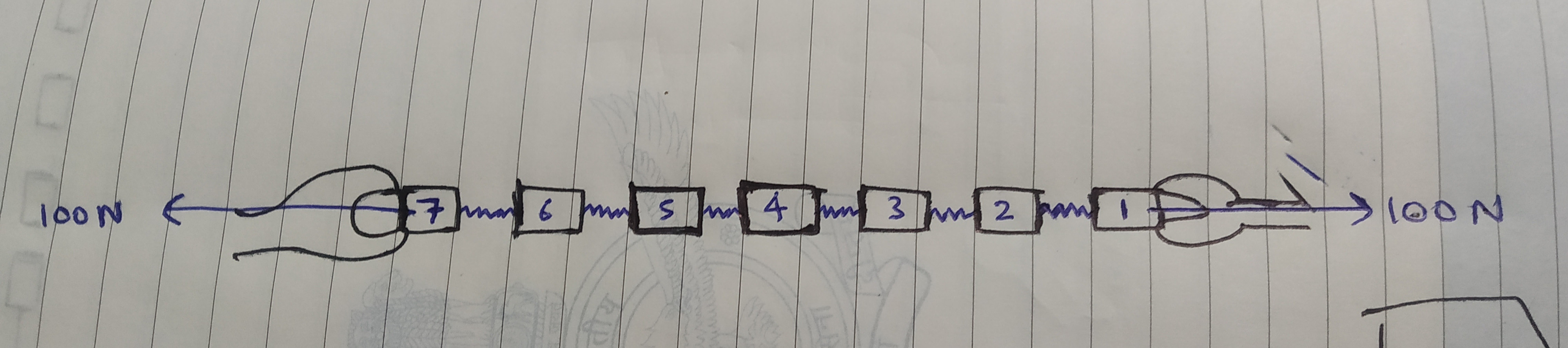
Now,for better understanding of tension,let us view the rope as a connection of molecules through which the rope is made of,something like this

1.the boxes represent molecules

2.The blue lines represent intermolecular forces

3.The boxes are numbered for a purpose you'll soon find out)

Now,the man pulls on molecule 1 with 100N, By Newton's 3rd law,the molecule 1 SHOULD pull the man with a force of 100N as well(am I right? Does the molecule 1 actually produce a reaction force of 100N which pulls the man?) 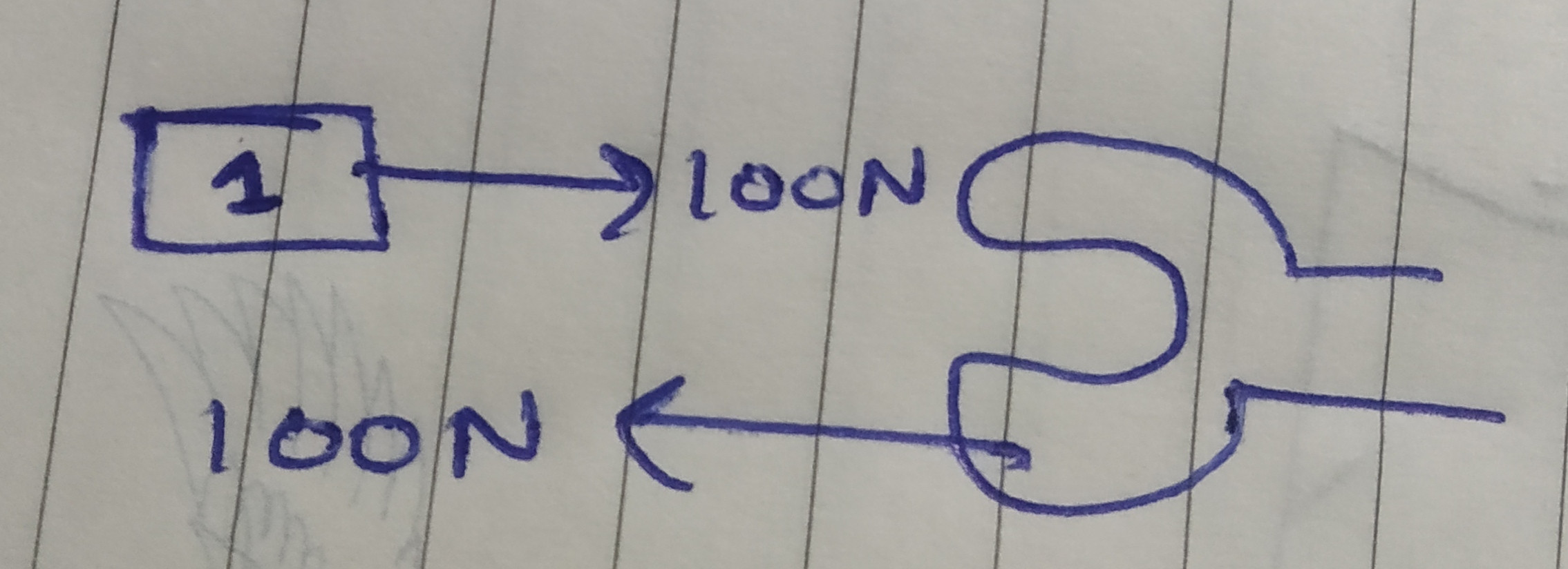enter image description here

Now,since molecule 1 is being pulled towards man,it will have some motion towards the right,as a result repulsive and attractive forces between molecule 1 and 2 would reduce,but there would be a net attractive force between molecule 1 and 2 due to Coulomb's force

enter image description here

The same thing follows at the other end, man pulls with 100 N on molecule 7,molecule 7 SHOULD produce reaction pulling force of 100N, Molecule 7 moves towards man,Coulomb's force develops between molecule 7 and 6enter image description here

Now,2 moves to right due to force F,so again repulsive and attractive forces between molecule 2 and 3 reduce but there is net attractive electrostatic attractive forces between molecule 2 and 3(Coulomb's force I think)

enter image description here

Same thing in molecule 6 and 5

And it goes on and on

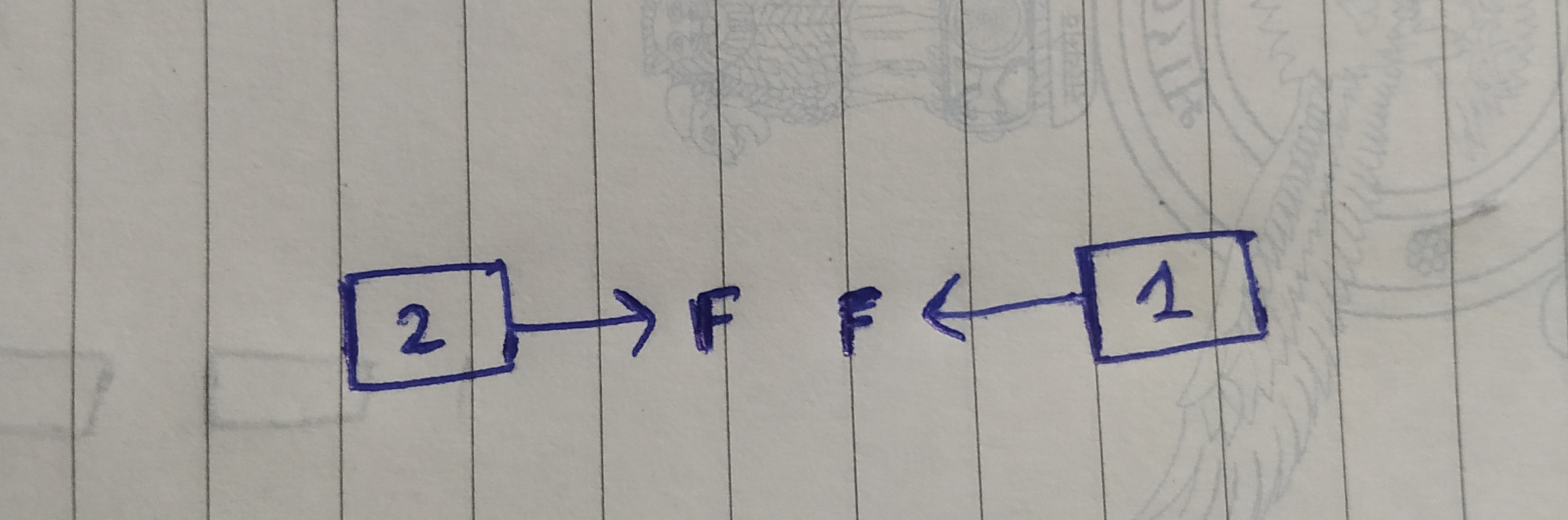
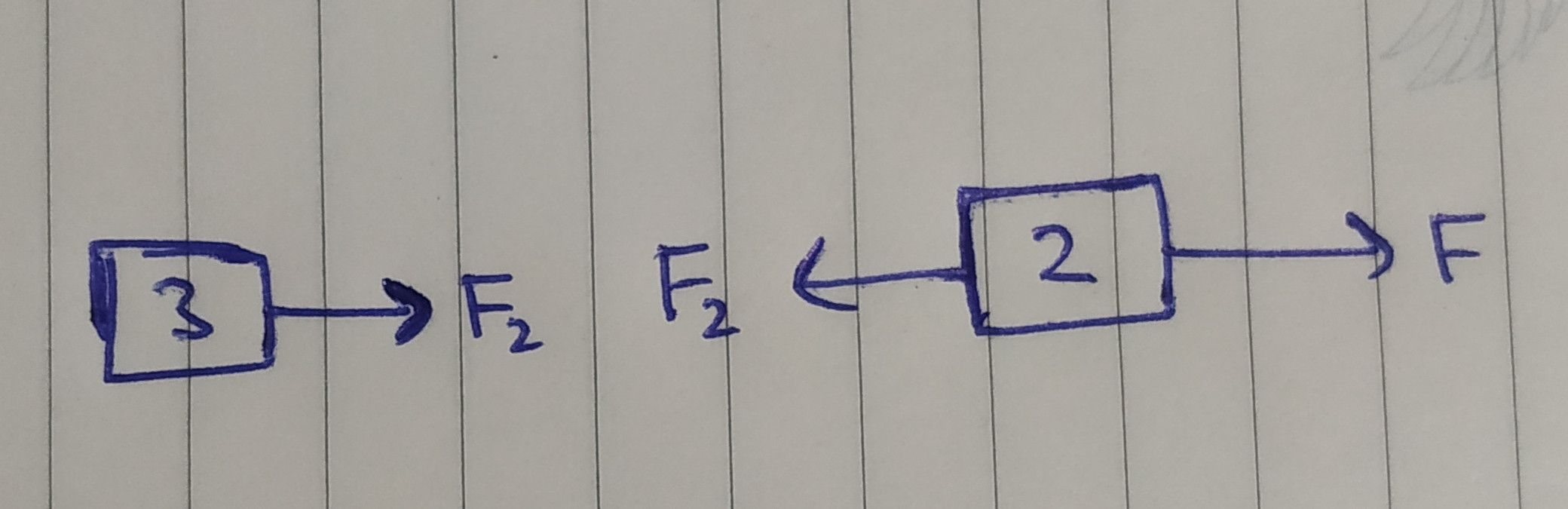
In short, free body diagrams of all the objects are as follows

enter image description here

Now,we know,net force on rope is 0,so by Newton's 2nd law(F=ma), We get the following values

Action pull force by man on molecule 1=100N(given in the situation)=Reaction pull force by man on molecule 1=100N(newtons 3rd law)=F=F2=F4=F5=F3=F1=action pull force by man on molecule 7(given)=reaction pull force on man by molecule 7(newton 3rd law)

Question

Which force,in this holy world,is tension out of all these forces? Many people say it's the reaction force due to pulling force,so is it the reaction force applied by molecule 1 and 7 on the men?but some people say it's the force by which one part of rope pull the other part,so is it force F,F1,F2,F3,F4,F5???some people say tension pulls the man,while others say tension is developed in the rope,WHICH ONE IS IT??????I know by 2nd law all these forces came equal in magnitude so it won't make any difference in values whichever I consider BUT I precisely wish to know which one of these is actually the holy "TENSION" Force??

Improve this question
$\endgroup$
4
  • 3
    $\begingroup$ I don't see why the definition matters. It's whichever one you care about. Usually, you care about the force exerted on an object by a rope, in which case we call this a tension force. But, in the case where the rope can be treated as massless and inextensible, the magnitude of this force is the same as the magnitude of the forces exerted by different parts of the string on each other and8 on any *other object the rope exerts a force on. Note that if any of your little links in the chain have mass, then this is not true: the forces will be different along the chain. $\endgroup$
    – march
    Commented Apr 27, 2023 at 16:05
  • 1
    $\begingroup$ As said in your previous question. Try to add spaces after punctuation (commas, periods, question marks). $\endgroup$
    – Mauricio
    Commented Apr 27, 2023 at 16:38
  • 1
    $\begingroup$ Also add dollar signs $ between mathematical variables for better readibility. For example $F$ reads $F$. $\endgroup$
    – Mauricio
    Commented Apr 27, 2023 at 16:41
  • $\begingroup$ If you like this question you may also enjoy reading this and this Phys.SE posts. $\endgroup$
    – Qmechanic ♦
    Commented Apr 27, 2023 at 16:51

2 Answers 2

Reset to default
12
$\begingroup$

Tension forces are the internal forces keeping the rope together. This means all of the $F$'s are tension forces, at different positions along the rope. In general they don't have to be all equal, e.g. in the case of an accelerating rope. But in general I would advise not getting too hung up on what a force is called. See also stress.

Improve this answer
$\endgroup$
5
$\begingroup$

Your mistake is thinking that there is only one tension force involved here. In fact, every point along the rope has its own tension, and in addition there is there is the tension that the rope exerts on its supports at either end. In the case of a light inextensible rope, the values of all of these tension forces are equal, so it is tempting but misleading to think of them as being one force, but in actual fact they are many forces which may or may not have the same value.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.