3
$\begingroup$

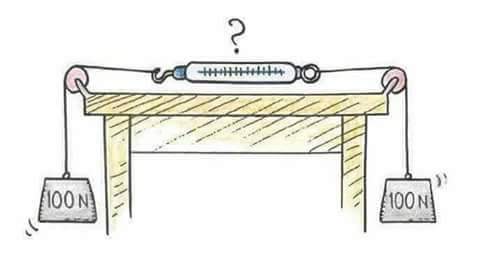
enter image description here

If $g=10 N/kg$ , what will show the Scales?

Yes, I know this is a very simple problem. But, I am stuck. $$P=mg \Rightarrow m=\frac{100N+100N}{10N/kg}=20 kg .$$

But, I'm worried about this answer. What's the difference between hand holding the scales and putting them on the table? I think, if the Scales is captured manually, the force applied from above should be meaningless. And answer must be $m=10 kg.$ Is it correct?

Improve this question
$\endgroup$
6
  • $\begingroup$ This is not a question about mathematics, but a question about physics. $\endgroup$
    – Arthur
    Commented Feb 1, 2018 at 7:57
  • $\begingroup$ Should I delete question? $\endgroup$
    – Newuser
    Commented Feb 1, 2018 at 7:58
  • $\begingroup$ I actually don't know. I have voted to have it moved to Physics, but for that to take effect, more people need to agree with me and actively vote to have it moved. $\endgroup$
    – Arthur
    Commented Feb 1, 2018 at 7:59
  • $\begingroup$ I understood. Sorry for this. I hope the question is not downvoted. I made mistake.. $\endgroup$
    – Newuser
    Commented Feb 1, 2018 at 8:05
  • $\begingroup$ Hold the scales vertical, with one weight pulling straight down, and the other pulling down via a pulley (i.e. pulling straight up, as far as the scales are concerned). Then what do you think? $\endgroup$
    – hdhondt
    Commented Feb 1, 2018 at 9:00

2 Answers 2

Reset to default
3
$\begingroup$

The answer is $10 kg$. When you apply Hooke's law to one end of a spring: $F=-kx$, it is implicit that the other end is fixed in place by a force $-F$. This force may be applied by the wall, hand, another mass, etc...

Improve this answer
$\endgroup$
-1
$\begingroup$

The idea is that when you would hang $1\mathrm{kg}$ in a massless basket under a scale, the scale would show that $1\mathrm{kg}$ in the basket. We do know Newtons second law that force equals mass times acceleration: $F=m a$. We know the gravitational acceleration on earth at sea level is approximately $g=10\mathrm{m/s^2}$ this tells us that the force being exerted for $1\mathrm{kg}$ is $10\mathrm{N}$. Now you have to imagine the other way around, when I have a force of $100\mathrm{N}$ pulling on both sides, what would the scale show now? It would show the same conversion but now the other way around so: $m=\frac{F}{a}$ with in this case $a=g$. So $F=200\mathrm{N}$ and $a=10\mathrm{m/s^2}$ gives $m=20\mathrm{kg}$.

Improve this answer
$\endgroup$
1
  • $\begingroup$ I think your answer is wrong. Answer must be $10 kg$ $\endgroup$
    – Newuser
    Commented Feb 1, 2018 at 10:01

Not the answer you're looking for? Browse other questions tagged or ask your own question.