Liquid in a container on a scale with internal and external forces

Question

In this question:

Two similar containers containing the same liquid are placed on two scales. We put a 5 kg object in the first container and it settles. In the second container, the same 5 kg object floats on the surface of the liquid. What is the numerical difference between the first and second scales?"

the answer is zero. and the normal force of the surface of the container when the object is settled does not cause the downward forces of the container (weight) to decrease.

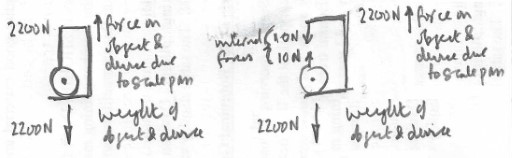
The problem is that in the original question I asked, the discussion is why the normal force does not affect the scale number because it is an internal force. This internality of power is the basis on which we choose our systems. And the choice is yours. So, if in the original question, only the liquid and the body were of the system, and the body of the container was of another system, the normal force would neutralize the body's weight and the scale would show another number.

Now the question is why weight is not neutral in the real world. The scale indeed shows the result of a downward force. Still, the problem is that, for example, when we pull a 20 kg object up with an external force of 10 newtons, we have caused the weight of the effective object on the scale to decrease. Because of that, The number of the scale is reduced, but when an internal force such as the normal force of the surface of the container is applied to the settled object and neutralizes the effect of its weight, we do not consider it!

The key thing I don't understand is how we say the normal force is internal and the F is external. if it is contractual, in the real world, everything should be considered a different system and then every force can affect the number of the scale!

Answer 1

The scale reading is the upward force that the scale pan exerts on the object(s) on the scale pan.

It is all about the system you choose and the initial and final conditions.

Still, the problem is that, for example, when we pull a 20 kg object up with an external force of 10 newtons, we have caused the weight of the effective object on the scale to decrease.

System - object alone

Initial condition - no external upward force on object - scale reading $200\,\rm N$ - net force on object $= 200-200 = 0\,\rm N$
Final condition - external upward force on object of $10\,\rm N$ - scale reading $200-10=190 \,\rm N$ - net force on object $= 190-190 = 0\,\rm N$

System - object and device, of total weight $2000\,\rm N$ which sits on the scale pan, for exerting an upward force on the object of $10\,\rm N$ when required

Initial condition - no upward force on object - scale reading $200+2000=2200\,\rm N$
Final condition - internal upward force on object - scale reading $200+2000=2200\,\rm N$ as the scale pan has to exert an upward force on the object and device of $2200\,\rm N$

Lets consider to two individual parts of the system.

System - object alone
Initial condition - no external force on object - part scale reading $200\,\rm N$ - net force on object $= 200-200 = 0\,\rm N$ Final condition - external upward force of $10\,\rm N$ due to device and part scale reading force of $190\,\rm N$ - net force on object $= 190 + 10 -200 = 0\,\rm N$

System device alone
Initial condition - no external force on object - part scale reading $2000\,\rm N$ - net force on object $= 2000-2000 = 0\,\rm N$
Final condition - external downward force of $10\,\rm N$ due to object and part scale reading force of $2210\,\rm N$ - net force on object $= 2000+10 -2010 = 0\,\rm N$

For the two individual systems the net upward force due to the scales was $190$ (due to object)$+2010$ (due to device) $= 2200\,\rm N$ - an unchanged scale reading.

And what might be my device for applying an upward force on the object?
The liquid in a container creating an upthrust.