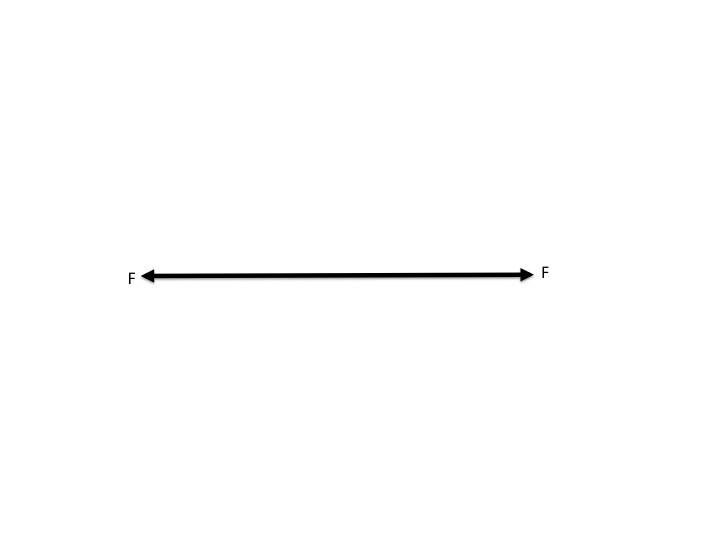
If you attach a ideal string to a wall and pull it with force F, there should be a tension T=F in the string. And the string will apply force F on the wall too.
But what if there are two persons pulling the same string in opposite directions with same force F? Shouldn't the tension in the string be 2F since both persons are applying force in opposite directions. Or the another person which is pulling the string is acting as wall just like in first case?
It's the latter; the second person is just acting like a wall.
In both the case of a second person, and in the case of the wall providing the tension, the equal and opposite forces make sure the system stays in equilibrium. As far as the rope is concerned, a person pulling in the opposite direction with a force $F$ and a wall pulling in the opposite direction with a force $F$ are the same thing.
To consider it another way, if you apply a force $F$ to the rope, and the other person holding it applies less than $F$, there will be a net force acting on the them + you + rope system, causing a net acceleration of the whole system. When forces are equal, the tension of any point in the rope can be said to be equal to the balanced tension forces acting on each side of the rope.
The other man indeed acts like the wall. As both the men apply the same force in opposite direction, our whole system is in equilibrium. Now, consider the free body diagram of the leftmost end of the string. That point is being acted upon by F on left and tension T on the right (we will not consider force F by the other man as in a FBD we always draw the forces directly acting on the particle). As acceleration is zero, F and T balance each other.
The wall, being a wall, is not moving.
You are correct that:
the string will apply force F on the wall too.
So it must counteract the tug of the rope by the same amount, F, to not move.
This is the whole principle of equilibrium.
In conclusion the free-body diagrams of the two situations look identical:
person1 wall/person2
<---█--->------------<---█--->
F T T F
Shouldn't the tension in the string be 2F since both persons are applying force in opposite directions?
No.
If you have two, equal but opposite forces at each end of the string, there will be no acceleration. Think about the game of tug of war. You have persons at each end of the rope pulling with equal but opposite forces $F$ so there is no acceleration of the rope. The sum of the external forces on the rope is zero. But tension is an internal force. You know its there because if you cut the rope, the contestants will go flying.
So do a free body diagram. Cut the rope at one end eliminating one group of contestants. You need to replace the removed contestants with the force $F$ that they applied in order to keep the other contestants from flying off. That force $F$ is the tension in the rope. To see this more clearly, then cut the other end of the rope and you will need to replace the other contestants with a force $F$ as well. The diagram below shows the rope as a free body with both ends cut. $F$ is the tension in the rope.
Hope this helps
