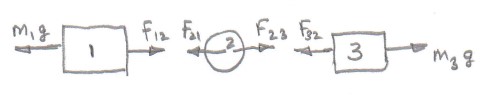13
$\begingroup$

According to Newton's third law, whenever objects A and B interact with each other, they exert equal and opposite forces upon each other. I have always struggled with how to apply this law to problems and real life.

Suppose I get a pull from my friend with some force, then I get pulled forward by a large distance as compared to the distance covered by my friend. Isn't this a case where Newton's third law of motion fails? Or, does this happen because of the difference in our masses?

My second question is about the tension in the rope in Atwood's Machine (two unequal masses connected by a rope on either side of a friction-less pulley). http://hyperphysics.phy-astr.gsu.edu/hbase/atwd.html I have solved various pulley mass problems but I have not thought about applying Newton's Third Law of Motion to it.

Is tension the counterforce to weight? Consider a segment of rope from which a mass is suspended in Atwood's Machine. That segment will experience a force $m1g$ from the weight. People say this weight is the reason for the counterforce in that segment, and that this force will travel all the way through the rope to the other segment resulting in a uniform tension. If this is correct then I can see the uniform tension around the rope as an application of newton's third law but the fact that this resulted from the weight negates the third law and also this will mean that m1=m2 where m1 and m2 are the masses at each segment ...can somebody explain this to me

Improve this question
$\endgroup$
4
  • 5
    $\begingroup$ A more vivid example of the first case is a horse pulling a sleigh. By Newton's third law, the force that the horse exerts on the sleigh is equal to the force that the sleigh exerts on the horse. It's only when we account the friction and the ground that we are able to understand that the duo moves horse-ward. $\endgroup$
    – svavil
    Commented Jan 21, 2018 at 18:13
  • $\begingroup$ @svavil Ah but real life is so complicated ;-). Are you suggesting that the friction force on the sleigh is smaller than the force with which the horse pulls? I don't thinks so (for constant velocity). If we assume, for the sake of argument, that the frictional force does not depend on the sleigh's velocity (and mind you, in reality the friction is probably weaker once the sleigh is moving!); then the force needed to keep the sleigh moving is not larger than the force needed to get it going from still-stand. The two are in equilibrium at constant velocity, even going backwards! $\endgroup$
    – Peter - Reinstate Monica
    Commented Jan 22, 2018 at 9:40
  • $\begingroup$ @svavil (ctd. ...) Big difference to the accelerating masses in Atwood's machine, where there is no equilibrium. $\endgroup$
    – Peter - Reinstate Monica
    Commented Jan 22, 2018 at 9:42
  • $\begingroup$ If it wasn't like this, then hitting a hammer with an egg would not produce the same results as hitting the egg with the hammer. $\endgroup$
    – Stian
    Commented Jan 22, 2018 at 10:37

5 Answers 5

Reset to default
14
$\begingroup$

If you and your friend are interacting, when he pulls you, he will feel that you apply to him the same force as he applies to you. If both of you are in vacuum and no further forces are present, the change in momentum will be equal for both of you. If further forces like friction are present, then the total forces acting on each of you might differ. But the parts which come due to you two interacting with each other will still be equal.

In the rope example there are two things playing a role and they should be kept apart:

  1. Each segment of the rope acts on any of the adjacent segments with the same force as the adjacent segment acts on it. Here we are looking at forces acting on different objects interacting with each other. This is Newton's 3rd law.
  2. The weight and the rope (and thus each segment of the rope) are in a force equilibrium, so the total force on each segment exercised by both adjacent segments totals to zero. Here we are looking at the forces on one object exercised by all other objects it interacts with.

While the first point is always true, the second one doesn't have to be true, say, if the rope and the weight are in a free fall accelerating towards the earth, rather than in an equilibrium situation. Obviously, there will be no tension force in case that the second point is not given.

Improve this answer
$\endgroup$
34
  • $\begingroup$ I understood the role of newton's third law in the first case but can you explain the second case some more as i have edited some parts in it.Sry for disturbing you $\endgroup$
    – Hydrous Caperilla
    Commented Jan 21, 2018 at 10:51
  • 1
    $\begingroup$ Say, you enumerate your segments from 1 to n. The weight is in equilibrium, so it feels two equal forces: $F_w=mg$ pulling it down and $F_1=F_w$ by the first segment of the rope pulling it up (this is point 2, equilibrium). The force pulling down the first segment from this interaction is again $F_1$ (this is point 1, Newtons law). Now, the first segment is in equilibrium as well, so it has to be pulled up with the same force (it is acted on by the second segment) as it is pulled down by the weight, so we have $F_2=F_1$. But now we know that the same force $F_2$ pulls the second segment down.. $\endgroup$
    – Photon
    Commented Jan 21, 2018 at 11:04
  • $\begingroup$ So you see, that in each step we have to apply both points: equilibrium condition and Newton's 3rd law. One of them ensures that the forces on each segment by its neighbours are equal (and total to zero due to different signs), the other one ensures that forces with which each pair of segments act on each other are equal as well. In total we have the same force throughout the whole rope. $\endgroup$
    – Photon
    Commented Jan 21, 2018 at 11:07
  • $\begingroup$ So each segment will remain at equilibrium as this goes on.......what will tension force be here...F1,F2...etc? $\endgroup$
    – Hydrous Caperilla
    Commented Jan 21, 2018 at 11:09
  • $\begingroup$ Now thinking this I got the idea about what is happening in equilibrium condition but I am now confused for the non-equilibrium part.How will tension vary for that case when both masses are accelerating $\endgroup$
    – Hydrous Caperilla
    Commented Jan 21, 2018 at 11:25
3
$\begingroup$

The motion of you and your friend depends on all the forces which are acting on you you and your masses.

When you pull your friend forward although the forces between you and your friend are equal in magnitude what about the force that you bother experience due to the Earth?

Assuming that you had equal masses if net force on your friend is greater than the net force on you then your friend will undergo a greater acceleration.

Mass will a play a part and so when you start walking towards the front of a moving train the change is speed is not noticeable although it possibly would be if you were in a small boat whose mass is much less than that of the train and thus closer to your mass.

The Atwood machine with one pulley can be thought of being equivalent to the following example.

enter image description here

Where the two masses at the ends of the rope are called $1$ and $3$ and the rope $2$ but you could extend it to being a whole string of as many masses as you wish.

The masses at the ends of the rope are subjected to gravitational attractive forces and the Newton third law force are labelled $F_{12}$ etc where $F_{12}$ is the force on mass $1$ due to mass $2$, the rope.

Taking towards the right as positive and applying Newton's second law gives.

$$m_3g-F_{32} = m_3a \;\;\; F_{23}-F_{21} = m_2a \;\;\; F_{12}-m_1g = m_1a $$
where is the acceleration of all the masses as the rope is assumed to be inextensible.

It is often the case that the rope is assumed to be massless which means that $F_{23}-F_{21} = 0 \Rightarrow F_{23} = F_{21}$ and these forces are called the tension in the rope.

Improve this answer
$\endgroup$
0
3
$\begingroup$

In the case of unequal masses attached to a massless rope supported by a frictionless pulley, the tension in the rope is the same everywhere within the rope. Using m1 to represent the smaller mass and m2 to represent the larger mass, and a to represent the rate of acceleration, then

tension = m1 (g + a) = m2 (g - a)

where

a = (m2-m1) g / (m1+m2)

The same formulas apply for m1 >= m2, corresponding to a <= 0.

Improve this answer
$\endgroup$
2
$\begingroup$

When you pull your friend both experience difference forces, you travel different distances because of several other factors (friction, mass, etc.)

Yes tension is created as a reaction to the weight but only when the mass attached to the string is not accelerating, in accelerating system the tension is the counter force to the force exerted by the particle on the string (not always equal to the weight).

Improve this answer
$\endgroup$
-1
$\begingroup$

When you are being pulled by your friend, the action is your friend pulling and the reaction is you moving forwards. That's the Third law. Now you move a bit ahead after he stops : because

  1. Probably the force was increased momentarily before he stopped.
  2. He kept pulling even after stopping.
  3. Quoting First Law of motion, an object in motion tends to remain in motion unless an external force acts on it. So you moved an extra distance until the external force of friction made you finally stop.

For simplicity, I consider tension as the opposite of compression. It is like friction. There is a limiting value of tension exceeding which the rope snaps. Tension is indeed the counter force of weight. It acts only when weight is present and that too in the direction of the string, opposite to the weight. Just as a reaction force. As per Third Law, the weight when attached to string must exert a force on the string (action) the string initiated a pulling force in the opposite direction to keep it attached (reaction) which is tension. So there.

It is indeed uniformly distributed in Atwoods machine. Only a massless string would have that. Even if 2kg and 5kg were attached to both ends the tension would have been uniformly distributed. How else would you explain the resultant acceleration if tension were localised? That's why the string moves because of residual force of 30N in my case. Tension balanced 2kg for 20N and 5kg for 50N and the rest caused acceleration. Here too the residual force 30N exerted on the string (action) caused a net acceleration (reaction). I hope this theory helps.

Improve this answer
$\endgroup$
1
  • $\begingroup$ -1 When the action is your friend pulling you, the reaction is you pulling your friend. This is the 3rd Law. Whether or not you move and in which direction depends on the resultant of all forces acting on you, not only the force applied by your friend. $\endgroup$
    – sammy gerbil
    Commented Apr 9, 2018 at 11:06

Not the answer you're looking for? Browse other questions tagged or ask your own question.