2
$\begingroup$

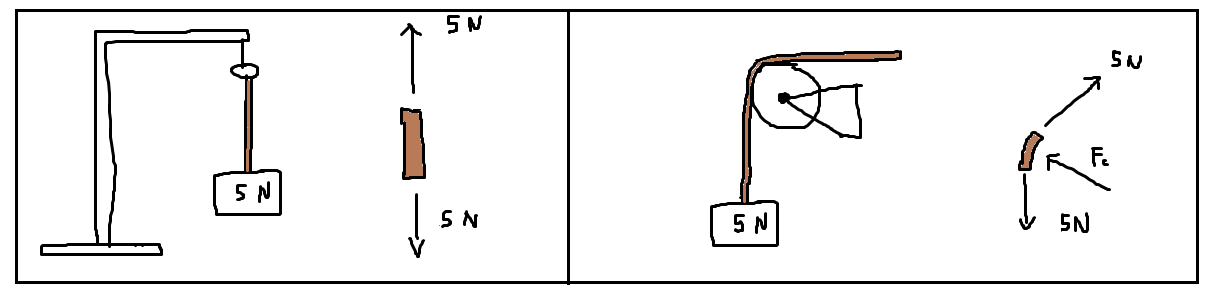
I am trying to understand if the "internal forces" in a rope around a pulley is the same as the "internal forces" in the rope from a weight without a pulley.

I know the tension before and after the pulley is the same (assume massless rope and smooth pulley), very good demonstration here, but what about part incontact with the pulley?

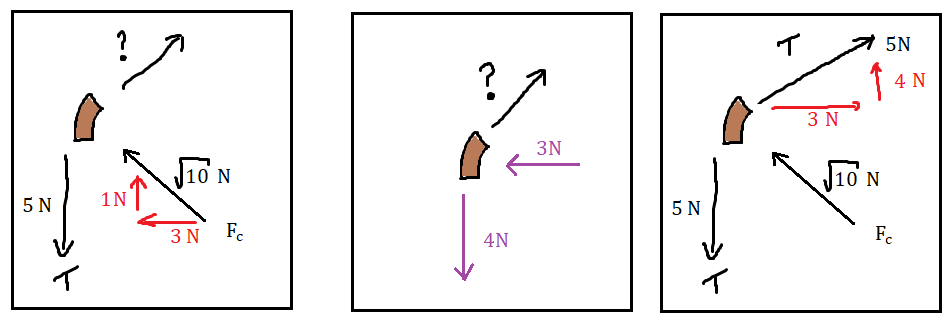
Here is my diagram: (the force diagram is next to each experiment)

enter image description here

The first picture makes shows clearly that the internal forces, tension is $10N$ throughout the rope, but for the second picture, there are $3$ forces on it (the third, $F_c$ is the contact force from the pulley).

If the system is in equilibrium, then the tension matches the force pulling from the bottom.

enter image description here

We know the tension in the middle of the rope is also $5N$ as if we were to cut the rope in half, then in order to keep the system in equilibrium, the end needs to have $5N$ of tension in it.

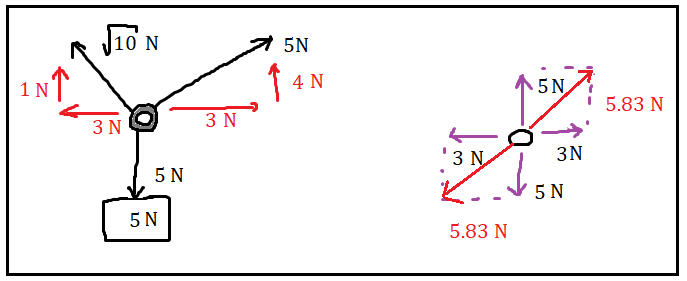
But if we look at the next experiment of 2 angled wires supporting a weight:

enter image description here

Here even when the tension top right is $5N$, the resultant "tension" (not sure if this is the right word) is actually $5.83N$ inside the ring.

My question: shouldn't the forces in each of the rope segments around the pulley be similar to that of the ring, where its slightly higher than the tension (or the force required to make the tension) at the end?

Improve this question
$\endgroup$
5
  • $\begingroup$ Something stands out to me. In the case of the pulley $F_c$ is radial from the axis of the pulley, so always perpendicular to the rope. The situation is different in the case of the ring. Does that have a bearing on your argument? Is the analogy between the two situaitons not close enough for comparison? $\endgroup$
    – garyp
    Commented Sep 19 at 13:26
  • $\begingroup$ A simpler example is pulling at something by 1 N in all directions. You can find various vector combinations that add up to more than 1 N, but it doesn’t seem particularly relevant, and it wouldn’t be correct to call that a “tension.” No single pulling force exceeds 1 N. $\endgroup$
    – Chemomechanics
    Commented Sep 19 at 14:46
  • $\begingroup$ I think you double-accounted the horizontal 3N. It is a component of the 5N tension, not a separate force. Also, the load on the rope segment would be distributed somehow, not a point load. But the net force would probably be as you have drawn. $\endgroup$
    – Mariano G
    Commented Sep 19 at 15:41
  • $\begingroup$ I suppose If I used a tubed cord like a hosepipe instead of a rope for the pulley, then the tension in the hosepipe parralel along it will be 5N. But we know the hosepipe will flatten against the pulley. Because tension is parralel to the hosepipe, it can't have contributed to the flattening, thus there must be extra strain on it in addition to 5N tension? $\endgroup$
    – Reuben
    Commented Sep 19 at 17:23
  • $\begingroup$ It just 'feels' like the rope over the pulley will more likely snap(suggesting more internal forces) than the one where the rope is just hanging down. But compressive force should not cause the rope to snap as I can increase the compressive force for the hanging rope purely by squeezing it, but this will not incresae the chance of snapping. So it must be something else. $\endgroup$
    – Reuben
    Commented Sep 19 at 17:44

1 Answer 1

Reset to default
3
$\begingroup$

the resultant "tension"(not sure if this is the right word)

You've identified the issue: "Tension" is not the right word. The tension refers to the axial load applied to the ends of a rope segment.

You've also correctly shown that an additional load (a lateral load) acts on the rope where it's curving around the pulley that's absent elsewhere, where the rope is pulled taut. This induces a compressive stress perpendicular to the axial tensile stress, and we'd expect a real material to deform accordingly. It doesn't alter the tension, though.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.