Order of Feynman diagrams for electroweak processes?

Question

I want to compare two Feynman diagrams and be able to say which one describes a process that is more likely to happen.

As far as I understand, this is done by considering the order of the diagram.

In the case of, for example, $e^+ e^- \rightarrow \mu^+ \mu^-$ (tree level) I have the two electrons coupling to the photon - introducing a factor of $\alpha_{EM}$, the coupling constant - and the two muons coupling to the same photon, introducing another factor of $\alpha_{EM}$.

At tree level, therefore, $$\Gamma_{tree} (e^+ e^- \rightarrow \mu^+ \mu^-) \propto \alpha_{EM}^2,$$ but if I were to include an electron-positron loop I'd have another $\alpha_{EM}^2$ therefore giving $$\Gamma_{1 loop} (e^+ e^- \rightarrow \mu^+ \mu^-) \propto \alpha_{EM}^4$$ and so on. In this case I understand that the power of $\alpha_{EM}$ is the order of the diagram.

ANYWAY, what I really want to quantify is this kind of processes:

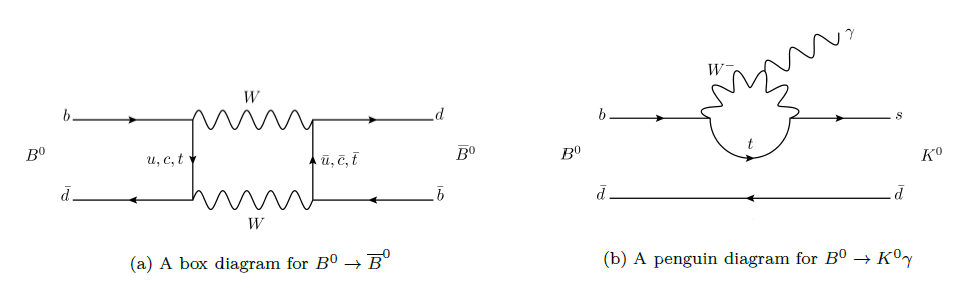
(a) I have 4 vertices of the $W$ bosons... so what, is this $\propto \alpha_{weak}^4$?

(b) By the same logic, $\propto \alpha_{EM}*\alpha_{weak}^2$?

Is (a) less probable than (b) then?

Answer 1

Your reasoning is essentially correct. Assuming that $\alpha_\text{weak}^4 \ll \alpha_\text{EM}\alpha_\text{weak}^2$, we can conclude that (a) is less probable than (b). As ACuriousMind notes, to be certain one should actually compute the full diagram, but the integrations from the loops usually give quite reasonable numbers that don't change the picture much, except maybe for specific values of external paramters. For example, a diagram like (a) may dominate if the virtual quarks are on-shell (although I don't think it'll matter in this particular case).