1
$\begingroup$

Consider the interaction $$e^-+e^- \rightarrow e^-+e^-$$ The following is a tree level Feynman diagram for this: 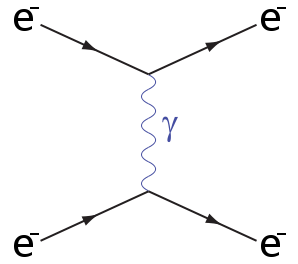enter image description here We can also make the paths of the two electrons on the right hand side cross over and this is a distinct diagram.

However lets consider the interaction $$e^-+e^+ \rightarrow e^-+e^+$$ The above diagram would be a tree level diagram in this situation (swap the top electron for a positron). However I am led to believe that in this case making the path of the electron and positron on the right hand side cross would not give a distinct diagram.

Why is this the case and generally when does a 'cross-over' give rise to a distinct diagram? Thanks :)

Improve this question
$\endgroup$

1 Answer 1

Reset to default
2
$\begingroup$

'Crossover diagrams' exist when the final products are indistinguishable. When using Feynman diagrams for computations, you need to add all distinct diagrams that depict the same process, i.e., have the same external lines. Both the crossed and uncrossed diagrams for the electron-electron process have the same final products, since you cannot tell the electrons apart. You can have one electron with momentum $p_1$ and another with $p_2$, or vice versa, and the process would look exactly the same to the experimenter. But the diagrams themselves are distinct, and need to be added.

However, for the electron-positron diagram, the electron and positron are distinguishable objects, and the crossed diagram is a different process altogether. $e^-(p_1)+e^+(p_2)$ is different from $e^-(p_2)+e^+(p_1)$ and the crossed diagram should not be added.

Improve this answer
$\endgroup$
4
  • $\begingroup$ Does this mean that crossing the electron and the positron is equivalent to swapping their momenta? Is this because a Feynman diagram is drawn in momentum space? $\endgroup$
    – Watw
    Commented Apr 9, 2016 at 19:18
  • $\begingroup$ Yes, crossing is equivalent to swapping momenta. Feynman diagrams aren't exactly drawn in momentum space (remember there is a time axis), but computation of the matrix element is done using particle momenta. Feynman diagrams are graphs used to depict successive terms in a perturbation series. They aren't exactly 'real' spacetime or momentum space depictions of processes. It is more a mathematical tool that simplifies calculations immensely. $\endgroup$
    – Gowri
    Commented Apr 9, 2016 at 19:29
  • $\begingroup$ Also, why doesn't crossing the two incident electrons give a new diagram? $\endgroup$
    – Watw
    Commented Apr 9, 2016 at 19:35
  • $\begingroup$ If you write down the matrix element for the crossed incident particles diagram, you will get the same term as for the crossed final particles diagram. Therefore, they are not distinct diagrams. Essentially, in both cases, the same pairs of particles are connected via the two vertices. In the uncrossed case, you get the other set of pairs (from performing one exchange). eg:- Uncrossed: (1->$\mu$->3)(2->$\nu$->4)(photon propagator), while, Crossed: (1->$\mu$->4)(2->$\nu$->3)(photon propagator) $\endgroup$
    – Gowri
    Commented Apr 9, 2016 at 20:19

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.