2
$\begingroup$

In QED, we are taught about the one-loop corrections and the counter-terms to the photon propagator, as well as the one loop corrections and the counter-terms to the fermion propagator. We are also taught about the one-loop correction to the photon-fermion-fermion vertex.

This is all nice and well, but I want to make sure that I understand how to apply these notions to a real interaction. Consider, for instance a $e^-\mu^-\rightarrow e^-\mu^-$ collision. I consider this interaction because one needs only one tree-level diagram in order to write down the Feynman amplitude.

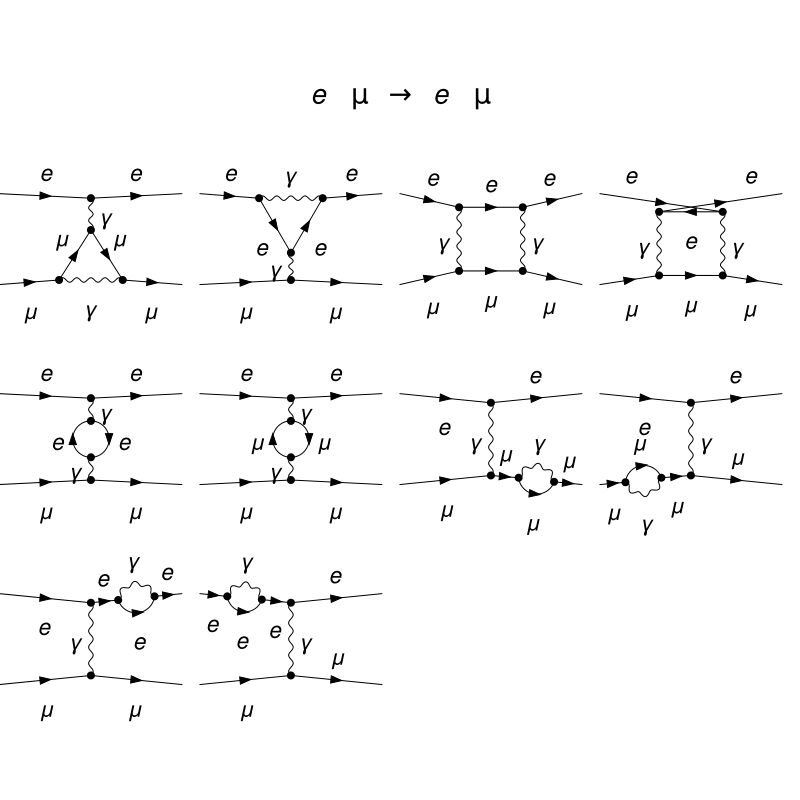
We wish to draw all the diagrams contributing to the Feynman amplitude at the loop-level. According to my understanding, I will modify the tree-level diagram such that my loop-level diagrams include

  1. Four fermion self-energy diagrams: in those diagrams an additional virtual photon will be added on the tree-level diagram, whose (virtual photon's) beginning and end will lie on the same external electron/muon.

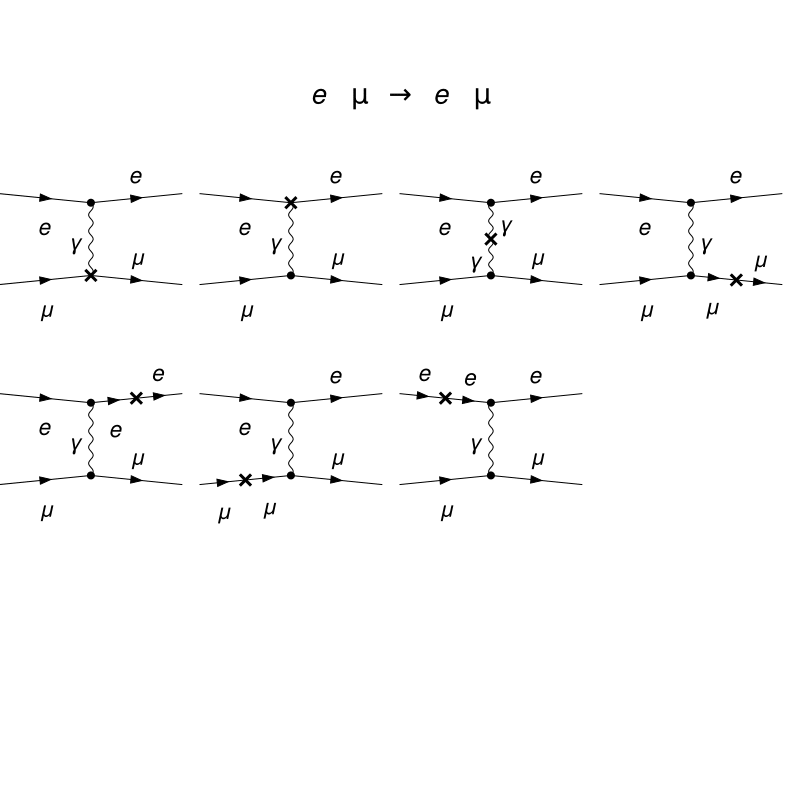
  2. Four counter-term diagrams for the fermion propagator

  3. Two vertex diagrams, one for the electron and one for the muon.

  4. Two photon self energy diagrams

  5. Photon self-energy counter-term diagram

  6. Box diagrams: these are obtained by the tree-level diagram by adding one virtual photon that connects (a) the initial electron with the initial muon and (b) the initial electron with the final muon.

So, in total, in the Feynman amplitude I need to consider $4+4+2+2+1+2=15$ diagrams of which I only need to calculate the two vertex diagrams and the two box diagrams, as well as the photon self-energy diagrams and its respective counter term diagram, because the remaining diagrams (electron self-energy) are negated by the respective counter-term diagrams.

Are my considerations correct? Any comment/suggestion will be helful.

Improve this question
$\endgroup$
9
  • 3
    $\begingroup$ Remember that the counter-terms are included to - yes - cancel UV divergences as you described but that the result of adding them is not null: the finite part of the self energy + counter term (or vacuum polarisation plus counter-term) is non-zero and momentum dependent (e.g. leading to running of coupling). $\endgroup$
    – nox
    Commented May 19, 2023 at 17:09
  • $\begingroup$ Yes, I guess I should edit :) $\endgroup$
    – schris38
    Commented May 19, 2023 at 17:11
  • 1
    $\begingroup$ Tip: 1. Consider to only ask 1 question per post. 2. Let's not have posts look like revision histories. $\endgroup$
    – Qmechanic ♦
    Commented Jun 6, 2023 at 9:07
  • $\begingroup$ IMHO drawing all the one-loop diagrams and evaluating them is not the best approach. Instead, recall that when we do renormalization we guarantee that the 1PI insertions become finite. The reason is simply that they are the building blocks of Feynman diagrams. So IMO a better approach is: (1) correct the propagators and vertices to one-loop order, (2) write down the tree-level diagrams for the process you want, (3) use the corrected vertices and propagators instead of the tree level ones. Just beware that you want to use only one corrected object per graph, otherwise you get two loops. $\endgroup$
    – Gold
    Commented Jun 7, 2023 at 12:45
  • 1
    $\begingroup$ The issue is the following. Any diagram can be written as a string of 1PI graphs connected to one another by tree level propagators. So the standard lore is that you correct the propagators and 1PI graphs and then you are basically done, because to evaluate an arbitrary diagram you use this decomposition. The issue is that I thought that the 1PI graphs were essentially all described by the corrections to the propagators and vertices. The box diagram looks like a counterexample, it seems like a 1PI graph that is not a correction to the vertices/propagators. I also must think about it. $\endgroup$
    – Gold
    Commented Jun 7, 2023 at 14:01

1 Answer 1

Reset to default
2
$\begingroup$

Using FeynArts to generate all diagrams for the given process, excluding Tadpole contributions, results in: enter image description here While the generation of counterterm diagrams yieldsenter image description here So basically you missed the vertex counter terms when you count all the diagrams. Furthermore, as also mentioned in the comments, the loop correction to the outer legs only add up to zero with the counter term insertions on the outer legs in the on-schell scheme. Then these corrections are "pushed into" the vertex counter terms. To calculate them you also need to compute the lepton self energies and the photon self energy! For a nice discussion on the one-loop renormalization of QED in the on-shell scheme see for example "Gauge Theories of the Strong and Electroweak Interaction" by Böhm, Denner and Joos.

Furthermore, since the photon is massless there probably are IR-Divergencies so you need to include real radiation diagrams.

Improve this answer
$\endgroup$
4
  • $\begingroup$ Hi @AlmostClueless and thank you so much for the reply. Some authors (see Srednicki) do not discuss anything about including -among the diagrams- the vertex counter term diagram. However, I think they include in their calculations the effects of adding the relevant counter terms. Am I right in assuming that? If yes, then is it a matter of taste whether or not one should include the vertex counter-term diagram in drawing the loop order diagrams for the interaction at hand? $\endgroup$
    – schris38
    Commented Jun 7, 2023 at 13:39
  • $\begingroup$ Also, thank you so much for referring me to a source and of course, I shall take great care in evaluating the IR-divergences and hence, include real radiation diagrams as well. Thanks! $\endgroup$
    – schris38
    Commented Jun 7, 2023 at 13:41
  • 1
    $\begingroup$ @schris38 Well, the whole renormalization framework is "a matter of taste" of some sort. I took a quick look at the book by Srednicki and I got the impression that the way they describe perturbative calculations is rather "old-school". I think of renormalization really as an effective field theory procedure, where you dress the couplings order by order with quantum corrections and get back an effective theory which is closer to the full theory. So when performing the so called "multiplicative renormalization transformation" it is natural to always include vertex counter terms. $\endgroup$
    – AlmostClueless
    Commented Jun 8, 2023 at 9:10
  • $\begingroup$ Okay, I think I see. Thanks a lot @AlmostClueless. So in my case (since I follow Srednicki for now) I do not draw any vertex counter term diagrams, but since I include $Z_1$, which is the counter-term appearing in the interaction term, in the Lagrangian I think I am good to go... The remaining diagrams are identical and I will be following the procedure in Srednicki to get the (renormalized) amplitudes $\endgroup$
    – schris38
    Commented Jun 8, 2023 at 11:26

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.