4
$\begingroup$

Setup: A rope is fixed at one end to a wall. You swing the other end up and down once. A wave starts travelling. It moves, hits the wall, then flattens, then is created again underneath (inversed), and then starts travelling back.

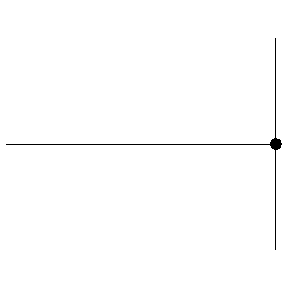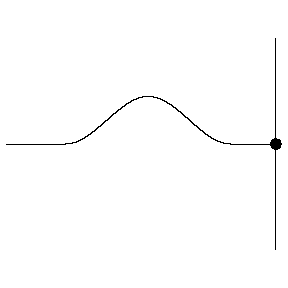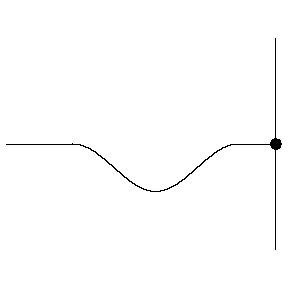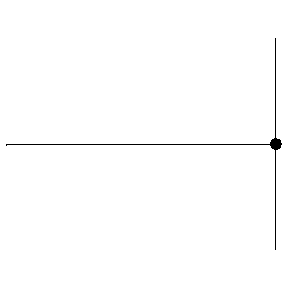
enter image description here

Question: The inversion itself makes sense. The wall responds with a force downwards on the rope when the wave in the rope tries to pull the wall upwards to continue (Newtons 3rd law). But why is there a delay?

At the point where the wave is perfectly flat, what is in that exact moment pulling the rope downwards? In that moment the wave is not pushing the wall upwards anymore, so the wall will also not give any force. Or am I wrong? Where to has the energy in the travelling wave been converted which gives it back to the rope in the flattened moment?

Improve this question
$\endgroup$
2
  • $\begingroup$ Nothing is pulling the flat rope, but remember the 1st Newton's law. $\endgroup$
    – Ruslan
    Commented Nov 18, 2014 at 14:58
  • $\begingroup$ @Ruslan, your comment was all I needed. Of course if the rope "particles" have a speed at the flattened moment they continue downward. Newton's 1st law. Simple, though this has draught me for weeks. If you give it as an answer I'll accept it. $\endgroup$
    – Steeven
    Commented Nov 19, 2014 at 11:40

3 Answers 3

Reset to default
5
$\begingroup$

At the point where the wave is perfectly flat, what is in that exact moment pulling the rope downwards?

Nothing. The force of the wall on the rope vanishes at that instant.

In that moment the wave is not pushing the wall upwards anymore, so the wall will also not give any force.

Right.

Where to has the energy in the travelling wave been converted which gives it back to the rope in the flattened moment?

It's in the kinetic energy of the rope.

Improve this answer
$\endgroup$
3
$\begingroup$

Putting comment into answer, as requested:

At the point where the wave is perfectly flat, nothing pulls it downwards. But to continue moving it doesn't need any force - it just obeys Newton's first law and moves with nonzero velocity.

Improve this answer
$\endgroup$
1
$\begingroup$

Think of it like this: when is the kinetic energy a maximum in this oscillation? At the point when $y=0$, or at the point at which the rope is flat. If there were a force pulling it, it would violate conservation of energy as from that point thereafter energy starts to transfer to potential energy of oscillation.

In short, the energy is transferring between kinetic and potential energies in the system. It's worth noting also that the transverse movement is the only real "movement" in the wave so to speak; that is, no point on the string is actually moving horizontally if you think about it.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.