Transverse waves in a rope: Why does tension not increase?

Question

So I've gone down the "waves" rabbit hole...

I found out that the propagation speed of transverse waves in ropes depends on the tension in the rope. At a first glance, this intuitively makes sense: If there is more tension in the rope, the internal forces pulling up and down the crests are stronger, which therefore makes them "move" faster.

Now on to my question:
Say we have a rope of length $r$ with tension $T$ and make it wave. To do so, one must lift up one end (by the distance $d$) and then quickly lower it again, while the other is fastened to a wall or something.

I know, I'm a talented artist...
I am aware this point where the rope bends upwards moves towards the wall untill it is completely straight, yet now inclined, again, but my point still holds. These sketches just show a moment in time.

This is the point where I get confused: If I lift the rope, but remain in the same horizontal position, that means I have to stretch the rope, because the distance is longer.

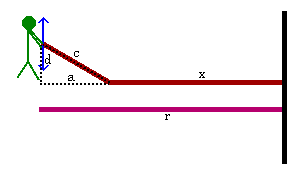
The rope's length now should be: $$r'=x+\sqrt{a^2+d^2}$$ As the rope increases in length, it must have some kind of elasticity, otherwise this action would immediately tear it apart.
Now then: The way I know elasticity, the forces (in this case the tension) increase when stretching something elastic. A rope should be no different. This increase depends on a material constant (let's name it $E$ for "elasticity" here). This should give us the new force $F$ acting inside the rope of: $$F=T+E*Strech$$ where the stretch is the length by witch the entire rope's length increased from lifting.
This should also happen along the crests of waves, so my question finally is:
Why does a rope's elasticity not affect wave speed? If a wave stretches and contracts a rope, how is it that the elasticity factors in different materials don't change how fast the waves travel?

Thanks for sitting through this mess!

Answer 1

The short answer is that the elasticity does affect the wave speed. However, when people typically talk about the wave speed on a taut string they are referring to very small disturbances. In the limit that the disturbance is infinitesimal, these phenomena you are referring to become negligible, and it is in this limit that the wave speed is defined.

I found a dissertation on nonlinear waves on a string with inhomogeneous properties that provides plenty of mathematical and physical detail on how to account for the elasticity of the string. From this dissertation we find that the first set of equations that account for elasticity (you need two because there is both vertical and horizontal displacement of the string) may be written as \begin{gather} u_{tt} - c_\lambda^2u_{XX} = 0, \\ v_{tt} - c_\tau^2v_{XX} = (c_\lambda^2-c_\tau^2)\left[ v_Xu_{XX} + v_{XX}u_X \right], \end{gather} where $u$ is the horizontal displacement of the string, $v$ is the vertical displacement, $X$ is the horizontal position of the string at rest, $t$ is time, subscripts denote partial differentiation with respect to the subscripted quantity, $c_\lambda^2=EA/\rho$ is the square of the longitudinal wave speed, $c_\tau^2=T_0/\rho$ is the square of the transverse wave speed, $E$ is the elastic modulus, $\rho$ is the mass density per unit length, and $T_0$ is the initial tension. The terms on the right-hand sides are all proportional to the product of two displacements. If we assume the displacements are small, then these nonlinear terms become very small. If we were to neglect them, returning to the linear equations, the two equations would decouple, and the vertical displacement equation would become the standard wave equation on a string people refer to.

Answer 2

Due to the disturbance the tension in the rope will increase from $T$ to $T+\delta T$ where $\delta T \ll T$ due to the elastic properties of the rope.

If the rope makes some small angle $\delta \theta$ from its equilibrium position due to a disturbance then the component of the tension perpendicular to the equilibrium position of the rope (the restoring force) will be $(T+\delta T) \sin \delta \theta\approx (T+\delta T) \delta \theta \approx T \delta\theta$ as the second order term can be neglected.

Since the $\delta T\delta \theta$ term can be neglected and $\delta T$ in some way depends on the elasticity of the rope, one does not need to know about the elastic properties of the rope when considering the propagation of a disturbance along the rope.

So as long as the disturbance is small enough to justify the approximation made above one does not need to consider the actual elastic properties of the string.

Answer 3

First of all, you cannot increase tension on a rope by stretching it without this having any elasticity. So elasticity of the material is necessary to increase tension. Increase in one dimensional tension translates to a reduction in the mass density of the material $ρ$ (mass per unit volume per unit of length since tension is related to longitudinal applied pulling forces).

Tension is a result of the elasticity of the material and of paramount importance. No elasticity, no tension. The higher the elasticity of the material the higher its ability to deform longitudinally and therefore the higher the possible applied tension on the rope (i.e. mass density reduction per unit of length).