1
$\begingroup$

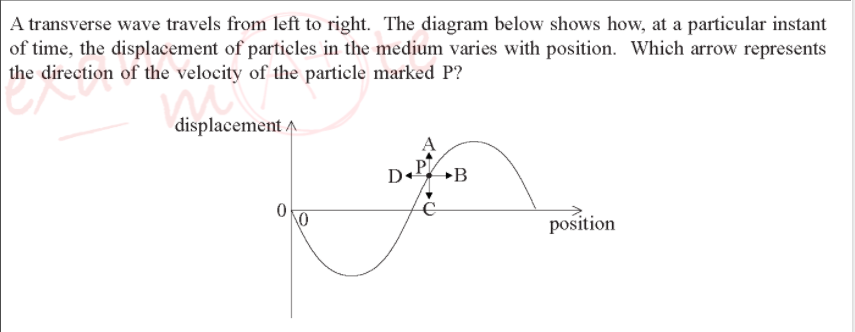
I have a good understanding of Displacement-time graphs and position-time graphs and how to interpret them. I take the IB diploma and came across this question from a 2009 paper with a displacement-position graph but our textbook does not help interpret it and neither can I find an internet article with explanations

Here is the question. So the answer is C meaning the velocity is downwards. But I thought the particle starts at its mean position, travels to the left and back to the mean position, and then begins travelling to the right. So at point P the particle should be moving in the right direction. The question says at the wave travels left to right so how can the velocity really be downwards?

enter image description here

Improve this question
$\endgroup$

3 Answers 3

Reset to default
2
$\begingroup$

The overall wave shown is traveling to the right. The individual particles in a transverse wave do not move to the left and right (parallel to the direction of the wave). They move up and down (transverse to the wave direction). What you see as a "wave" is really just the procession of particles moving up and down in sequence as if they were on little springs. They are moving in a direction transverse to the direction of the wave!

You can simulate the effect with a bedsheet that's tucked in on both sides. Place a small object on the sheet and then slide your hand underneath it. You can consider the direction you slide your hand to be the wave's direction. You'll notice as your hand approaches it, the particle itself doesn't move in the same direction as your hand, it moves up. As your hand passes underneath it, the particle begins moving back down (this is where the particle in your diagram is).

If the wave were a longitudinal wave (a compression wave like sound waves and waves down a slinky when you give it a push along the length of the spring) then the individual particles would move parallel with the wave direction (the individual particles still stay close to their original position, only the wave travels).

In fact, whether a particle moves perpendicularly or parallel to the wave's direction is what defines whether a wave is transverse or longitudinal.

Improve this answer
$\endgroup$
3
  • $\begingroup$ Wonderful! Thank you so much, I tried the bedsheet thing and I understood how to solve it. Rlly appreciated $\endgroup$
    – Snehal Bhadani
    Commented Apr 11, 2022 at 9:46
  • $\begingroup$ Glad it helped you. A nice way to think of how waves work is if you consider a line of beads (particles) all connected by little springs (restoring forces) in a line. If you tug on one particle, it tugs on the next one down the line a bit, which tugs on the next, and so on. Remember, the wave itself is an emergent behavior, the particles moving back and forth in sequence are what makes it a wave. Depending on what restoring forces are present, you can get transverse, longitudinal, or both types present (simultaneously even!). It all depends on the media (material). $\endgroup$
    – March Hare
    Commented Apr 12, 2022 at 3:45
  • $\begingroup$ Thank you for that lovely explanation. Your comment is inspiring me to find more joy in physics, because usually with physics I never tried to visualise anything and have been memorising my textbook and trying to get away with it. Loved your explanation of waves $\endgroup$
    – Snehal Bhadani
    Commented Apr 24, 2022 at 9:22
1
$\begingroup$

The particle does not travel left-right. It is a transversal wave traveling left-right. This means that the particles are moving either up-down or forward-backward (perpendicular to the page). None of the particles in the wave are mving-left-right. Here they don't consider the direction perpendicular to the page so the only direction to consider is up-down To see if it's up or down just draw the same graph for a little later time. You know that it propagates to the right so it will be shifted to the right. Then look at the displacement of the same particles on the two graphs.

Improve this answer
$\endgroup$
1
  • $\begingroup$ Thank you so much. $\endgroup$
    – Snehal Bhadani
    Commented Apr 11, 2022 at 9:46
1
$\begingroup$

Since the wave is an transverse wave the particles will move perpendicular to the direction of propagation so we can eliminate B,D

Now we know that the velocity of any particle is given by $v_{Particle}=-v_{wave}*\text{(Slope of the graph at particle)}$

Proof: Let equation of wave be $y=A\;sin(wt-kx+a)$ then velocity of particle is: $\frac{dy}{dt} = v_{particle}=A \omega \; cos(wt-kx)$ ①

Slope of graph is: $dy/dx= m =- Ak \; cos(wt-kx+a)$ ②

From equation ①,②: $v_{particle}=-\omega/k*m=-v_{wave}*m$ (Since velocity of wave is given by $\frac{w}{k}$)

As per the given question wave travels from left to right ie v(w)= positive and the slope of graph at given particle location is also positive So if we apply this, $-(\text{positive }v_{wave})(\text{positive slope})=\text{negative }v_{particle}$, so it moves downward (c is your answer)

Improve this answer
$\endgroup$
1
  • $\begingroup$ Thank you for the reply. Helped me a lot in understanding, rlly appreciate $\endgroup$
    – Snehal Bhadani
    Commented Apr 11, 2022 at 9:44

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.