In a non-interacting Fermi gas at zero temperature ($T=0$), the momentum distribution function, denoted by $n(\mathbf{k})$, is given by the Heaviside step function: $n(\mathbf{k}) = \theta(k_F - |\mathbf{k}|)$, which equals 1 for $|\mathbf{k}| < k_F$ and 0 for $|\mathbf{k}| > k_F$, where $k_F$ is the Fermi momentum and $\theta(x)$ is the Heaviside step function. This sharp discontinuity at $|\mathbf{k}| = k_F$ arises directly from the Pauli exclusion principle, which dictates that at $T=0$, all single-particle states with momenta $|\mathbf{k}| < k_F$ are occupied while all states with $|\mathbf{k}| > k_F$ are unoccupied.
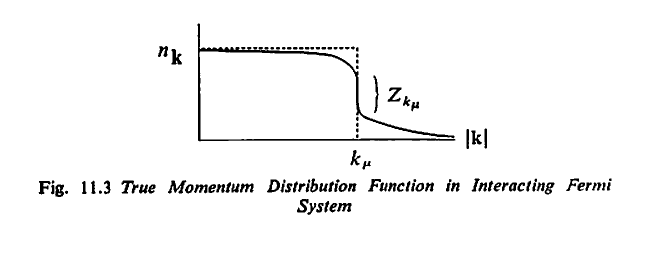
When interactions are introduced, Landau's Fermi liquid theory describes the low-energy excitations as quasiparticles – long-lived, fermionic excitations with renormalized properties. The momentum distribution function $n(\mathbf{k})$ deviates from the ideal step function; however, a crucial feature persists: a finite discontinuity at $|\mathbf{k}|=k_F$, quantified by the quasiparticle residue, $Z$. This discontinuity is defined as $\Delta n(k_F) = n(k_F^-) - n(k_F^+) = \lim_{\epsilon \to 0} \left[ n(k_F - \epsilon) - n(k_F + \epsilon) \right] = Z$, where $n(k_F^-)$ and $n(k_F^+)$ denote the limits of $n(\mathbf{k})$ as $|\mathbf{k}|$ approaches $k_F$ from below and above, respectively. The quasiparticle residue $Z$, where $0 < Z \leq 1$, is a measure of the overlap between the quasiparticle state and the bare fermion state, and can be expressed in terms of the Green's function $G(k,\omega)$ through the relation $$Z = \left. \frac{\partial}{\partial \omega} G(k_F,\omega)^{-1}\right|_{\omega=0}^{-1}$$.
The persistence of this discontinuity is fundamental. The Fermi surface is defined as the locus of points in k-space where this discontinuity in $n(\mathbf{k})$ occurs, specifically at $|\mathbf{k}|=k_F$. Luttinger's theorem states that the volume enclosed by the Fermi surface, $V_{FS}$, (related to $k_F$ through $V_{FS} \propto k_F^d$, where d is the spatial dimension) is invariant under interactions, provided the system remains in the Fermi liquid phase. This can be formulated in terms of the electron density $n$ as $$\sum_{k,\sigma} n(k, \sigma) = n$$, and $V_{FS}$ can be related to this quantity. This invariance is topologically protected and intimately connected to the conservation of particle number. The volume enclosed by the Fermi surface can also be expressed through the Green's function in terms of a topological invariant: $$V_{FS} = \frac{1}{2}\int \frac{d^dk}{(2\pi)^d} \operatorname{tr} [G(\mathbf{k}, \omega\rightarrow 0^+) - G(\mathbf{k}, \omega\rightarrow 0^-)]$$. The finite quasiparticle residue $Z$ is crucial for the existence of well-defined quasiparticles with long, but finite, lifetimes ($\tau \propto \epsilon^{-2}$, where $\epsilon$ is the energy above the Fermi level) near the Fermi surface ($|\mathbf{k}|\approx k_F$). The presence of $\Delta n(k_F)$ signifies that the interacting system is adiabatically connected to the non-interacting Fermi gas, as long as $Z \neq 0$. The mathematical foundation for these concepts is the single-particle Green's function, $G(\mathbf{k}, \omega)$, which can be expressed as $$G(\mathbf{k}, \omega) = \frac{Z}{\omega - \epsilon_k + i\delta} + G_{inc}(\mathbf{k}, \omega)$$, where $\epsilon_k$ is the quasiparticle dispersion and $G_{inc}$ is an incoherent part. Luttinger's theorem can be derived by analyzing the pole structure of $G(\mathbf{k}, \omega)$ as $\omega \rightarrow 0$, specifically showing that the volume enclosed by the singularity in $G(\mathbf{k}, \omega)$ does not change with interactions. The Green's function near the Fermi surface also has a direct relation to the momentum distribution function:
$$n(\mathbf{k}) = -i\int_{-\infty}^{0} \frac{d\omega}{2\pi} G(\mathbf{k},\omega)$$.
In systems where the discontinuity vanishes ($Z \rightarrow 0$), such as one-dimensional Luttinger liquids or certain strongly correlated materials, we are no longer in the Fermi liquid regime. In these non-Fermi liquids, $n(\mathbf{k})$ becomes a smooth function with no discontinuity at $|\mathbf{k}| = k_F$. In this case, defining the Fermi surface can become more abstract, and may require other approaches based on the single-particle Green's function or spectral functions, like the spectral function $$A(k,\omega) = -1/\pi Im[G(k,\omega)]$$, and, for example, the location of the maximum in $A(k, \omega=0)$.
In multi-band systems, each band crossing the Fermi level can give rise to distinct Fermi surfaces, each characterized by its discontinuity $\Delta n(\mathbf{k}_F)$ associated with the specific band's Fermi momentum. This is particularly relevant in strongly correlated systems where Fermi surface reconstructions occur due to complex electronic correlations. The experimental observation of this discontinuity is typically achieved using Angle-Resolved Photoemission Spectroscopy (ARPES), which measures the spectral function which provides information on $n(\mathbf{k})$, allowing for the determination of the existence or absence of the discontinuity, therefore, providing crucial experimental evidence for Fermi or non-Fermi liquid behaviors. Near quantum critical points (QCPs), strong fluctuations and diverging effective masses can suppress $Z$ significantly, leading to non-Fermi liquid behavior where $Z \rightarrow 0$ and the quasiparticle description breaks down.