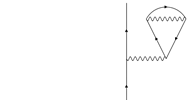
I am studyng how to construct Feynman diagams for the perturbative expansion of the one-particle Green function (or propagator) using the book "A Guide to Feynman Diagrams in the Many-Body Problem". One of the second-order diagrams for the Green function is shown below.
According to the book, when we have a system of identical fermions interacting through a momentum-conserving potential (like the Coulomb potential), this diagram can't contribute. To do this, the book use only physical arguments, but I would like to show this mathematically. The problem is that I can't show that it is equal to zero. I will explain what I am doing. The perturbative expression for the Green function is
$$G(k_{1},k_{2},t,t') = \sum _{n=0}^{\infty} \frac{(-i)^{n}}{n!} \int dt_{1} \cdots \int dt_{n}\ \langle \Phi_{0}| T[H_{I}(t_{1}) \cdots H_{I}(t_{n})a_{k_{1}}(t)a_{k_{2}}^{\dagger}(t')] |\Phi_{0} \rangle$$
devided by the vacuum amplitude (I am using standard notation, so I won't spend time defining everything that appears above). We can write every interaction $H_{I}$ using creation and annihilation operators
$$H_{I}(t_{1}) = \frac{1}{2} \sum_{l} \langle l_{1}l_{2}|V|l_{3}l_{4} \rangle a_{l_{2}}^{\dagger}(t_{1})a_{l_{1}}^{\dagger}(t_{1})a_{l_{3}}(t_{1})a_{l_{4}}(t_{1}),$$
$$H_{I}(t_{2}) = \frac{1}{2} \sum_{m} \langle m_{1}m_{2}|V|m_{3}m_{4} \rangle a_{m_{2}}^{\dagger}(t_{2})a_{m_{2}}^{\dagger}(t_{2})a_{m_{3}}(t_{2})a_{m_{4}}(t_{1}),$$
and apply the Wick's theorem to express the time-ordered product using contractions of these operators. The diagram above represent the following contractions
$$ \overbrace{a_{l_{2}}^{\dagger}(t_{1})a_{m_{4}}(t_{2})} \overbrace{a_{l_{1}}^{\dagger}(t_{1})a_{k_{1}}(t)} \overbrace{a_{l_{3}}(t_{1})a_{k_{2}}^{\dagger}(t')} \overbrace{a_{l_{4}}(t_{1})a_{m_{1}}^{\dagger}(t_{2})} \overbrace{a_{m_{2}}^{\dagger}(t_{2})a_{m_{3}}(t_{2})}$$
Now, using the particle-hole notation, where $a_{k} = b_{k}$ if $k > k_{F} =$ Fermi momentum and $a_{k} = c^{\dagger}_{k}$ if $k < k_{F}$, where $b$ is the annihilation operator for a particle and $c^{\dagger}$ the creation operator for a hole, the contractions are
$$\overbrace{b_{k}(t_{1})b_{l}^{\dagger}(t_{2})} = \begin{cases} \delta_{k,l} e^{-i\varepsilon_{k}(t_{1} - t_{2})} & \text{ , } t_{1}>t_{2} \\ 0 & \text{ , } t_{1} \leq t_{2} \end{cases}$$
and
$$\overbrace{c_{k}^{\dagger}(t_{1})c_{l}(t_{2})} = \begin{cases} 0 & \text{ , } t_{1}>t_{2} \\ -\delta_{k,l} e^{-i\varepsilon_{k}(t_{1} - t_{2})} & \text{ , } t_{1} \leq t_{2} \end{cases}$$
However, by my calculations, if I use this expressions and integrate, the result is not zero. Does anyone have any ideas? I'm sorry if my question is confused...