Why doesn't this diagram appear in the partition function in zero-dimensional QFT?

Question

For the zero-dimensional QFT with action $$S(\phi)=\frac{\alpha}{2}\phi^2+\frac{\lambda}{4!}\phi^4-J\phi,\tag{1}$$ we can perturbatively expand the partition function as $$Z_\lambda(J)=\int_{-\infty}^{\infty} d\phi~e^{-S}=\sum_{r=0}^{\infty}\frac{1}{r!}\left(\frac{-\lambda}{4!}\right)^r\left(\frac{\partial^4}{\partial J^4}\right)^r\exp\left(\frac{J^2}{2\alpha}\right),\tag{2}$$ which at first order in $\lambda$ gives $$-\frac{\lambda}{4!}\left(\frac{J^4}{\alpha^4}+\frac{6J^2}{\alpha^3}+\frac{3}{\alpha^2}\right).\tag{3}$$

This can be expressed via the diagrams

since we know we need one vertex and to contract in line with Wick's theorem. What I'm unsure about is why we can't also have the disconnected diagram

since it has the same number of sources, propagators and vertices as the second diagram above. This diagram does show up when calculating the second moment $\langle\phi^2\rangle$ using the partition function.

Answer 1

Well, OP partition function $Z[J]$ does in fact contain disconnected diagrams, such as, e.g. OP's last diagram $8|$, cf. the linked cluster theorem $Z[J]=\exp(\frac{i}{\hbar}W_c[J])$.

Concretely, the propagator $|$ in OP's last diagram $8|$ comes from the bag $\exp\left(\frac{J^2}{2\alpha}\right)$ of propagators in OP's eq. (2).

Answer 2

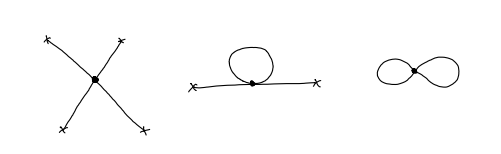
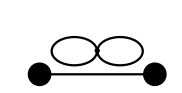
Starting from $S(\phi,J) = S_0(\phi) + S_{\rm int}(\phi) - J \phi$ with $S_0(\phi) = \alpha \phi^2/2$ and $S_{\rm int}(\phi) = \lambda \phi^4\!/4!$, the generating function(al) of the full model (including the interaction term) is given by $$ Z(J) = \frac{\int \! d \phi \, e^{-S_0(\phi)} e ^{-S_{\rm int}(\phi)} e^{J \phi}}{\int \! d\phi \, e^{-S_0(\phi)}e^{-S_{\rm int}(\phi)}} =: \left\langle e^{J \phi} \right\rangle,$$ which can be rewritten as $$Z(J) = \frac{\int \! d\phi \, e^{-S_0(\phi)} e^{- S_{\rm int}(\phi)} e^{J \phi}}{\int \! d\phi \, e^{-S_0(\phi)} } \cdot \frac{\int \! d\phi \, e^{-S_0(\phi)} }{\int \! d\phi \, e^{-S_0(\phi)}e^{-S_{\rm int}(\phi)} } = \frac{\left\langle e^{-S_{\rm int}(\phi)} e^{J\phi} \right\rangle_0 }{ \left\langle e^{-S_{\rm int}(\phi)} \right\rangle_0}, $$ where $\left\langle f(\phi) \right\rangle_0$ denotes the Gaussian mean value defined by $$\left\langle f(\phi) \right\rangle_0 := \frac{\int \! d\phi \, e^{-S_0(\phi)} f(\phi)}{\int \! d\phi \, e^{-S_0(\phi)}}. $$ The perturbative expansion of the term $\left\langle e^{-S_{\rm int}(\phi)} e^{J \phi}\right\rangle_0$ is given by $$ \left\langle e^{-S_{\rm int}(\phi)} e^{J\phi} \right\rangle_0 = e^{-S_{\rm int}(\partial_J)} \left\langle e^{J\phi}\right\rangle_0 = e^{-S_{\rm int}(\partial_J)} Z_0(J), $$ with the generating function of the free theory $Z_0(J)= \left\langle e^{J \phi} \right\rangle_0 = e^{J^2/2 \alpha}$, leading to the compact expression $$ Z(J) = \frac{e^{-S_{\rm int} (\partial_J)}Z_0(J)}{e^{-S_{\rm int}(\partial_J)}Z_0(J) \Large|_{J=0}}. $$ Expanding up to terms linear in $\lambda$, one finds $$e^{-S_{\rm int}(\partial_J)}Z_0(J) = e^{J^2/2\alpha} \left[1-\frac{\lambda}{4!}\left(\frac{J^4}{\alpha^4} +\frac{6 J^2}{\alpha^3}+\frac{3}{\alpha^2}\right) +\mathcal{O}(\lambda^2) \right].$$ With the help of the comments, you had realized yourself that you had simply forgotten the exponential term $e^{J^2/2 \alpha}$ in this expression. Note that the term in the denominator, $$e^{-S_{\rm int}(\partial_J)} Z_0(J) {\large|}_{J=0} = 1 -\frac{\lambda}{4!} \frac{3}{\alpha^2}+ \mathcal{O}(\lambda^2),$$ cancels all graphs with disconnected vacuum bubbles (like your last diagram), arriving at $$Z(J) = e^{J^2/2 \alpha} \left[ 1 - \frac{\lambda}{4!} \left( \frac{J^4}{\alpha^4} + \frac{6 J^2}{\alpha^3} \right)+ \mathcal{O}(\lambda^2) \right]$$ and $\left\langle \phi^2 \right\rangle =1/\alpha - \lambda/2 \alpha^3 +\mathcal{O}(\lambda^2)$.

Alternatively, employing the Wick theorem, you find $$\left\langle \phi^2 \right\rangle =\frac{\left\langle e^{-S_{\rm int}(\phi)} \phi^2 \right\rangle_0}{\left\langle e^{-S_{\rm int}(\phi)} \right\rangle_0 } = \frac{\left\langle \phi^2 \right\rangle_0 - \frac{\lambda}{4!} \left\langle \phi^6 \right\rangle_0+ \mathcal{O}(\lambda^2)}{1 - \frac{\lambda}{4!} \left\langle \phi^4\right\rangle_0 + \mathcal{O}(\lambda^2)} = \frac{\frac{1}{\alpha}- \frac{\lambda}{4!} \frac{15}{\alpha^3}+ \mathcal{O}(\lambda^2)}{1- \frac{\lambda}{4!} \frac{3}{\alpha^2}+ \mathcal{O}(\lambda^2)} = \frac{1}{\alpha} - \frac{\lambda}{2 \alpha^3} + \mathcal{O}(\lambda^2),$$ in agreement with the previous result.