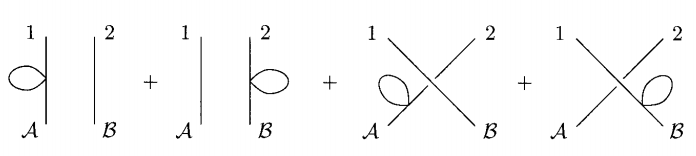On which propagator does the field self-contraction loop go on this Feynman diagram?

Question

This question relates to page 111 in Peskin and Schroeder.

I am trying to do the derivation of the 2-particle to 2-particle Feynman diagrams in $\phi^4$ theory by hand, following Peskin and Schroeder. We use Wick's theorem to obtain the following expression:

$$\langle p_1p_2|\mathcal N\left(-i\frac{\lambda}{4!}\int d^4x\text{ }\phi(x)\phi(x)\phi(x)\phi(x)+\text{contractions}\right)|p_Ap_B\rangle. \tag{4.92}$$

I have obtained the correct diagrams for the case in which all fields are contracted, and now I am trying to obtain the diagrams for the case in which two fields are contracted with themselves and the two remaining fields are contracted on the initial/final states. I have worked through the calculations without too much grief and obtained the following expression:

$$-\frac{i\lambda}{4!}D_F(x-x)\left[ \delta(p_B-p_1)\delta(p_A-p_2)+\delta(p_B-p_2)\delta(p_A-p_1)+\delta(p_A-p_1)\delta(p_B-p_2)+\delta(p_A-p_2)\delta(p_B-p_1) \right] \tag{1}.$$

At this point the Feynman propagator is simply multiplied into the delta functions. However, In Peskin and Schroeder the "loops" from the Feynman propagator are drawn onto the diagrams in the following way:

In words, the loops representing $D_F(x-x)$ are attached to (for example) the $1$-$A$ external leg in the first diagram and the $2$-$B$ external leg in the second diagram, even though in both cases (assuming my equation above is correct) the Feynman propagator is multiplied in the same way into the delta functions. My question is whether this is something I need to be careful about, or would it be equally correct to simply attach the $D_F(x-x)$ loop to an arbitrary propagator?

Answer 1

Your result is correct. As you know, each component of a Feynman diagram combines multiplicatively. Meanwhile, the kind of loop that you have here does not depend on the momentum of the propagator that it attaches to. Loosely speaking, this is because the loop both "absorbs and emits" its own momentum into the vertex. It doesn't affect and is not affected by the momentum in the other propagator, which just "passes through" the vertex. Because of this, all of the four loops in these four diagrams are identical, even though the diagrams themselves are in principle not identical. Since the loops are all identical, you can just factor them out, as you have done. Now you are left with a loop factor in front, and the sum over four diagrams with plain propagators.

Just two comments:

• This kind of loop is particular to $\phi^4$ theory. In a general theory, the 1-loop correction will depend on the momentum of the propagator, and this factorization won't happen. See, for example, Yukawa theory. So don't get used to this kind of factorization.
• It's still important to count all the possible diagrams, because physically they are there. The factorization is just a convenient outcome of this special case. You have two pairs of identical terms in your square braces now, and that factor of 2 is important.