Peskin and Schroeder Eq. 11.103: how does it relate to one-loop diagrams?

Question

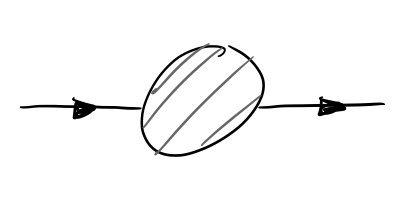
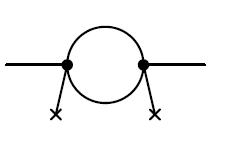
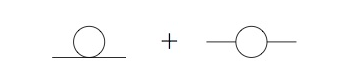I am a bit confused by how Peskin & Schroeder describe the corrections to the two point function of the linear sigma model from the second functional derivative of the effective action. Without going into too much details on p386 (Eq. 11.103) they derive the second functional derivative of the functional determinant and find \begin{align} \frac{i}{2} \frac{\delta ^2}{\delta \phi^l (w )\delta \phi^k (z)} &\log \det [-i \mathcal{D}] =-\lambda ( \delta^{kl}\delta^{ij} + \delta^{jl}\delta^{ik} + \delta^{il}\delta^{jk}) \delta (z-w) \left( \mathcal{D}^{-1}\right) ^{ij} (z,z)\nonumber\\ & +2i \lambda^2 \left[ \phi^k(z) \delta^{ij} + \phi^j (z) \delta^{ik}+ \phi^i(z) \delta^{jk}\right] \nonumber\\ &\times \left( \mathcal{D}^{-1}\right) ^{im}(z,w) \left[ \phi^l(w) \delta^{mn} + \phi^m (w) \delta^{ln}+ \phi^n(w) \delta^{lm}\right] \left( \mathcal{D}^{-1}\right) ^{nj}(w,z). \tag{11.103} \end{align} I have dropped the subscript $_\text{cl}$ for convenience. So far so good. They then say that it is related to the inverse propagator and that we recognise the one-loop diagrams. I have added these diagrams at the end of the question (apologies for the formatting, I am not sure how to do it better).

I really don't understand how these are related. The first line of the equation has no field, so I expect no external line; I would have expected a vacuum bubble. The second and third line have a $\lambda^2$ factor so I expect two vertices, but the first diagram only has one vertex. They also have two $\phi$ fields and two propagators, so again how does the first diagram fit in? Or does the first diagram correspond to the first line of the equation, but then where do the external lines come from?

I am probably missing something simple here, but any help to put me on the right track would be much appreciated.

Answer 1

Indeed, these diagrams are misleading to the beginners. I was asking exactly the same questions (to myself) when I first encountered them on this page.

There are two points need to be explained:

1) the external lines in these figures are NOT the "external lines" in the terminology of a scattering process. These lines actually play the same role of those appearing in a propagator, as shown in the following picture

Apparently, the lines in the above figure do not mean external scattering particles. So we understand the figures in your post are actually propagators (1PI as you know), they will finally appear in the internal parts of a full scattering process.

2) The classical fields are suppressed in the figures. For instance, the $\lambda$ is a 4-vertices interaction, while in the second figure, it looks like a cubic interaction, what is wrong? The reason is that, the authors suppressed the classical fields. If you take it rigorously, you should have a figure like this.