4
$\begingroup$

I have developed a method to calculate the time of sunrise, sun noon and sunset, but it does not take the atmosphere into account. Therefore, in order for my results to be useful in practice, I need to know the time interval between the sunrise with refraction and without refraction.

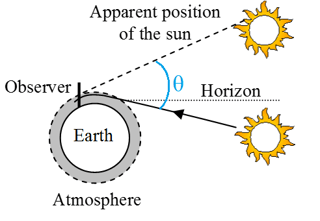
By using the Stellarium software I have determined that it is about 7-8 minutes (such that the sun rises 7-8 minutes earlier due to the presence of the atmosphere), although it varies depending on latitude, because the angle at which the sun rises is different - though I believe the angle between the incoming light and the final, refracted, light would be enough to calculate the time interval, which may be independent of latitude and which is shown as $\theta$ in the image below.

enter image description here

Is there a way to calculate this time interval or the previous angle, or does it need to be experimental?

Improve this question
$\endgroup$
1
  • $\begingroup$ This question is on-topic here, but it would probably be better on Astronomy.SE. Actual sunrise / sunset times can vary from even the best predicted times because of the weather. FWIW, using standard refraction formulae I can calculate sunrise / sunset times that differ by less than a minute from the times given by Horizons. $\endgroup$
    – PM 2Ring
    Commented Jun 7, 2022 at 7:08

1 Answer 1

Reset to default
1
$\begingroup$

The "standard" altitude given in the Astronomical Almanac, is that the center of the Sun should be 0.8333 degrees below the horizon to account for refraction. [For stars it's 0.5667]. Here is an example implementation.

Improve this answer
$\endgroup$
3
  • $\begingroup$ Why is the refraction angle different for sunlight versus starlight? Perhaps a thermal effect? $\endgroup$
    – rob ♦
    Commented Jun 7, 2022 at 3:43
  • $\begingroup$ @rob It's accounting for the mean angular radius of the Sun. You use a similar factor for the Moon, since its angular diameter is similar, although for the Moon you also need to adjust for the effects of parallax. However, the refraction may be substantially larger than the mean, due to temperature & pressure, especially near the horizon, which sometimes creates mirages. "Basic Principles of Marine Navigation" by D. A. Moore (1964) says "Nevertheless, when abnormal refraction is suspected, all observations taken under these conditions should be treated with caution". $\endgroup$
    – PM 2Ring
    Commented Jun 7, 2022 at 6:42
  • $\begingroup$ From siranah.de/html/sail040o.htm Under "normal" conditions - when the Standard Atmosphere is a fair approximation - k is about 1/6 to 1/7. When there is a strong temperature inversion, k can be as large as 1. Values larger that 1 correspond to ducting condition: if the observer is inside the duct, a pseudo-horizon appears above the astronomical horizon, so the dip of this apparent horizon is negative, a remarkable phenomenon that is observed occasionally. $\endgroup$
    – PM 2Ring
    Commented Jun 7, 2022 at 6:49

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.