0
$\begingroup$

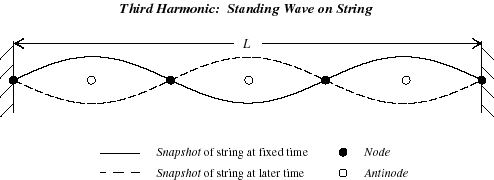
I’m studying standing waves and I got stuck with this question. The answer key says ‘A’ is the correct answer, but I think ‘B’ is the correct answer.

My reasoning. The speed of the wave depends on the medium. As we change the frequency of the wave it changes its form to go into the next harmonic so the wavelength changes.

In this question, it says an adjustment is made -say the density of the particles in the environment has changed- so the speed of the standing wave changes. It shouldn’t change its frequency, am I wrong?

enter image description here

Improve this question
$\endgroup$

2 Answers 2

Reset to default
1
$\begingroup$

The wavelength of standing waves in a pipe would have to depend on the length of the pipe, since we are dividing the length of the pipe between the standing waves. For example, in the third harmonic, we are dividing the length of the pipe, let's say $L$, into three parts for each 'node-node' pair in the wave, thus giving us $\frac{2L}{3}$ as the wavelength. enter image description here

This is waves on a string, but the concept is the same.

So, when the density of the particles in the pipe has been changed to change the speed of sound as you said, you may observe that we're still talking about the first harmonic here, which means exactly two antinodes are present on either end of the pipe, and thus the wavelength remains $2L$ as the length of the pipe has not changed. Finally, the frequency will increase by the virtue of wavelength remaining constant while the velocity of the medium has increased.

Your answer key is therefore correct.

Improve this answer
$\endgroup$
4
  • $\begingroup$ I disagree with the point that the wavelength depends on the length of the pipe. Let’s change the example to the standing wave on a rope by a vibration generator. I can increase the frequency and it changes the wavelength of the standing wave, so the length of the pipe or rope is not the only factor here. It may stay constant but we can still change the wavelength of the standing wave. What am I getting wrong here? $\endgroup$
    – AliceX
    Commented Apr 14, 2022 at 13:38
  • 1
    $\begingroup$ @AliceX You're forgetting that we are talking about standing waves here. I'd suggest you look into how standing waves are formed in the first place, then you'll be able to answer why we can't get standing waves by putting just any frequency in the vibration generator. Standing waves form at integral multiples of a certain frequency. These frequencies(which we call harmonics) are just right to give rise to a situation where waves travelling in one direction interfere with the waves from the opposite direction(these other waves could be reflected off a wall). $\endgroup$
    – Reet Jaiswal
    Commented Apr 15, 2022 at 10:07
  • 1
    $\begingroup$ Thus we get nodes and antinodes, nodes being the points which don't move and antinode being the points with maximum displacement. Since the end attached to the vibration generator is necessarily an antinode, and the end attached to the wall is necessarily a node, we can only use specific frequencies in order to generate standing waves that fit this configuration in a consistent repeating pattern. At any other frequency in between we will simply get a chaos of waves. $\endgroup$
    – Reet Jaiswal
    Commented Apr 15, 2022 at 10:25
  • 1
    $\begingroup$ Therefore, for a given pipe or rope, the wavelength of the $n^{th}$ harmonic will never change, since it completely depends on the length itself. If the velocity of the medium changes, the frequency will alter to adjust to the wavelength, and not the other way round. $\endgroup$
    – Reet Jaiswal
    Commented Apr 15, 2022 at 10:29
1
$\begingroup$

The question asks you to consider the first harmonic before and after the adjustment is made. Wavelength $\lambda$ will be determined by the geometry of the pipe (length and whether ends are closed or open), which does not change. Speed is related to frequency and wavelength via $v = f \lambda$, so frequency $f$ must increase by the same factor as the speed of sound $v$. Thus A is correct.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.