The wavelength of standing waves in a pipe would have to depend on the length of the pipe, since we are dividing the length of the pipe between the standing waves. For example, in the third harmonic, we are dividing the length of the pipe, let's say $L$, into three parts for each 'node-node' pair in the wave, thus giving us $\frac{2L}{3}$ as the wavelength.
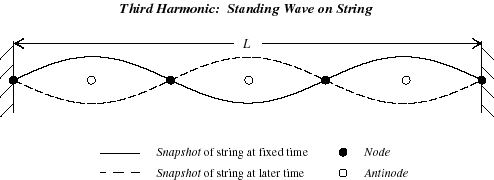
This is waves on a string, but the concept is the same.
So, when the density of the particles in the pipe havehas been changed to change the speed of sound as you said, you may observe that we're still talking about the first harmonic here, which means exactly two antinodes are present on either end of the pipe, and thus the wavelength remains $2L$ as the length of the pipe has not changed. Finally, the frequency will increase by the virtue of wavelength remaining constant while the velocity of the medium has increased.
Your answer key is therefore correct.
