I'll start of with my (mis)understanding:
We have a planet orbiting around another body. The orbiting planet has infinitely many axes passing through it, but we're interested in two, $A_1$ and $A_2$. $A_1$ is the axis that is perpendicular to the planet's orbit, whereas $A_2$ is the one passing through its poles.
The planet is rotating around $A_1$. When $A_2$ is exactly superimposable onto $A_1$, then this is a rotation without precession. If $A_2$ is at an angle from $A_1$ however, we've got precession. Let's say that the point at the tip of $A_2$ is called $a_2$. When there is no nutation, $a_2$ orbits around $A_1$ in some elliptical path.
When there is nutation, then the that orbit is disturbed in a specific way. Let's define an abstract point, $p$, that orbits around $A_1$ identically to how $a_2$ would, if there was no nutation. So, the way I've understood nutation is that it's when $a_2$ orbits around $p$.
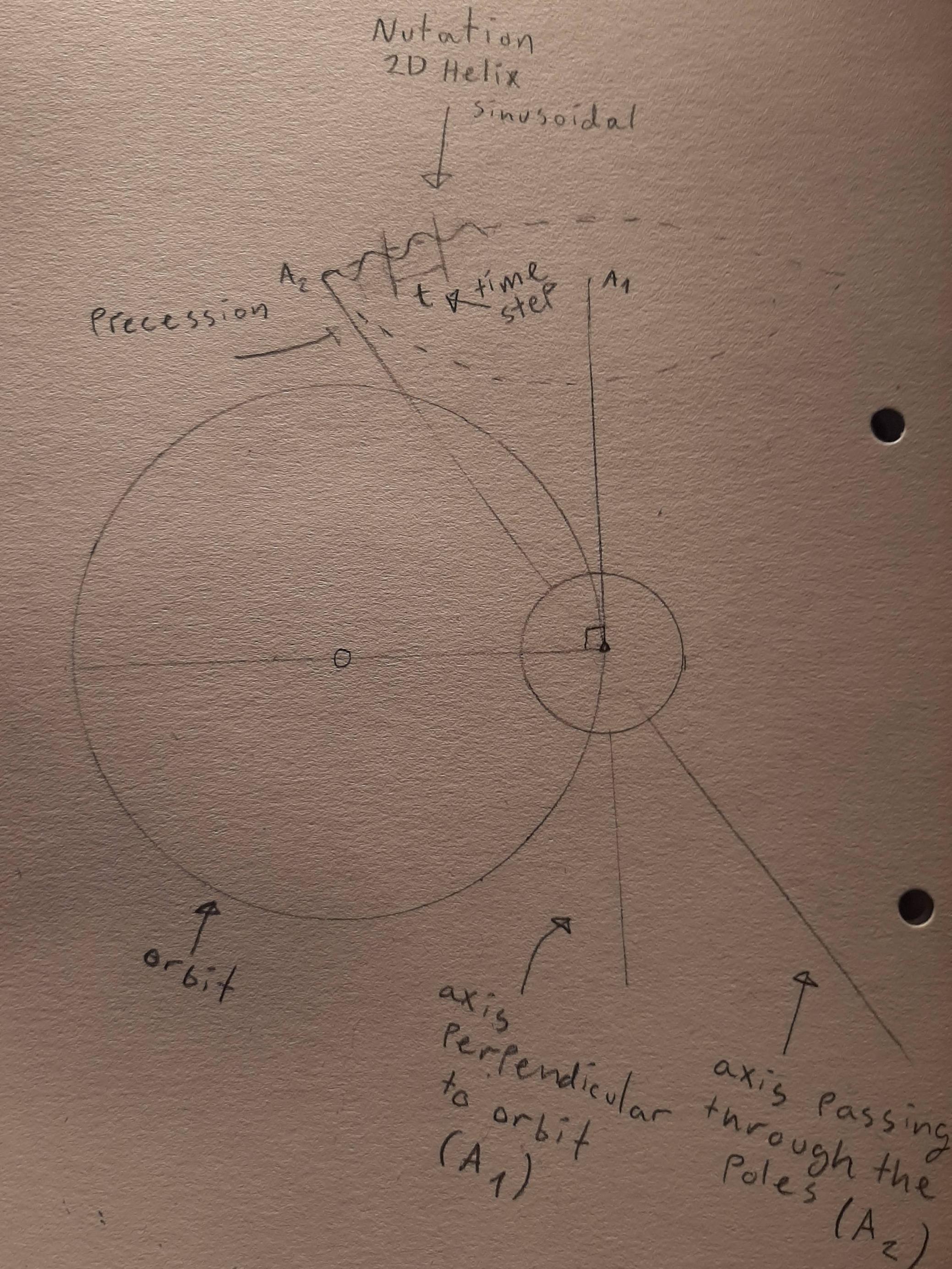
In the lower left, you see the orbit of $a_2$ around $p$ quite clearly. However, that is a contrived scenario where $p$ isn't moving. Thing is, $p$ is in orbit around $A_2$, which means that circle gets stretched out into a $2$D helix. We could make it into a $3$D helix by defining an axis $t$, and define integer values of $t$ as the time taken for $a_2$ to orbit around $p$ once. If you look at the first photo, that time step is demarcated onto the helix.
In this Math.SE post, I defined a function, $_rF_n(t)$, that gives us the coordinates that trace out the movement of $a_2$. It takes $t$ as the input, and outputs a two-dimensional value, corresponding to where $a_2$ exists at time $t$. If one looked at this function, it would be a $3$D helix. However, one could look at that $\Bbb R^3$ space in such a way so as to compress it into two dimensions. Basically, you position the observer's line of sight perpendicular to the $t$-axis (the $x$-axis in this photo).
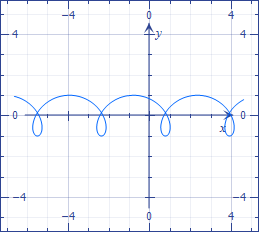
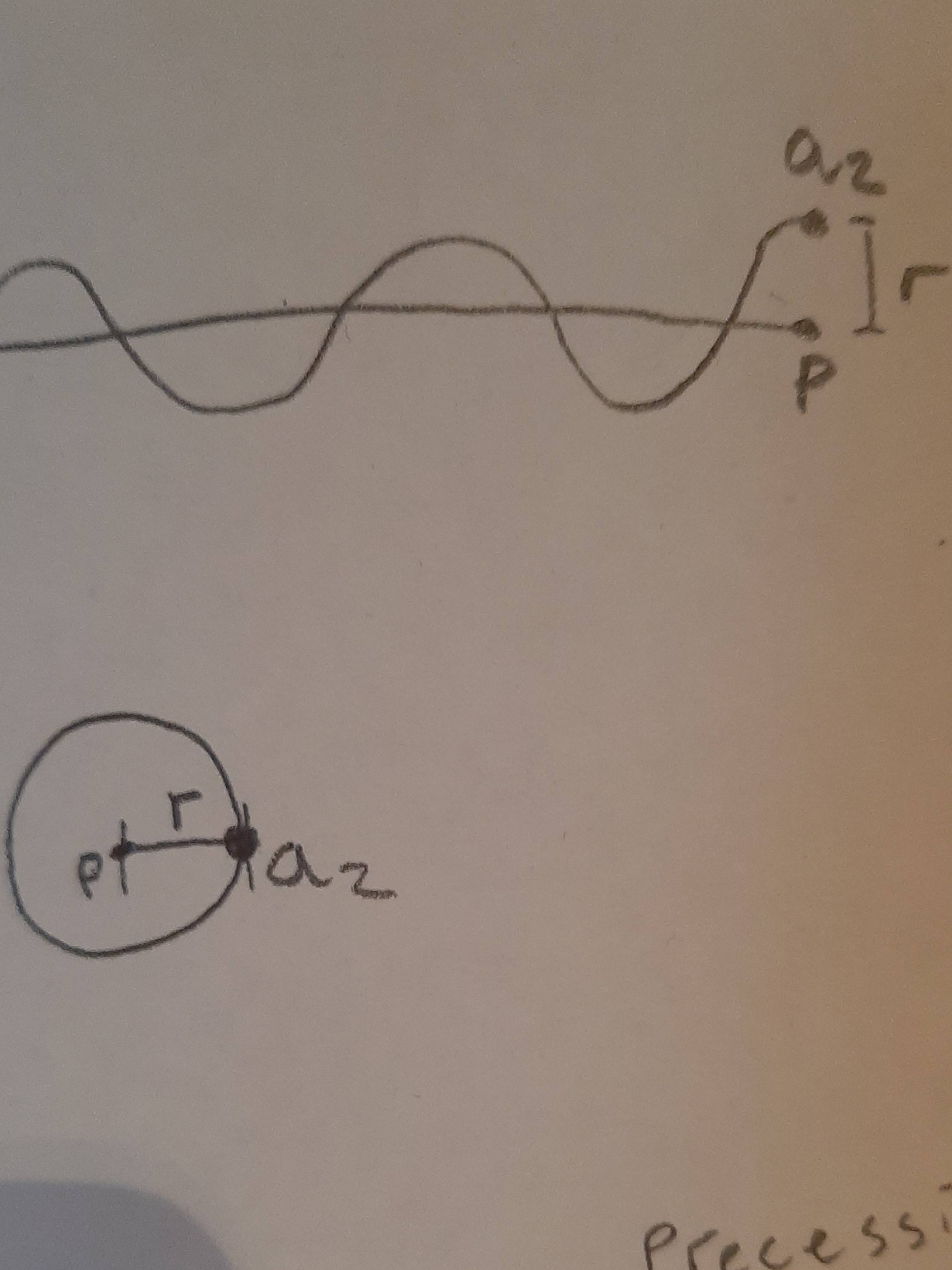
And this get's you the path traced out by a point orbiting around another point that is moving. The above picture was taken from user65203's answer to this Math.SE post. Now, in the second photo of this post, I defined $r$ as the distance between $a_2$ and $p$. Now, if $p$ moves a distance $\ge 2r$ per time step, that means that there will be no looping (by loops, I mean the loops you see in the above $2$D helix). When the velocity of $p$ exceeds $2r$ per time step $t$, you get a sinusoidal wave as the curve traced out by the movement of $a_2$. The reason is that $p$ moves too fast for $a_2$ to loop back on itself, for a lack of more precise wording. In other words, by the time $a_2$ has finished its orbit, $p$ has moved a distance of $2r$, thus meaning it has no chance of crossing over a point is has been before. To illustrate this, let me show the middle point between $n = 0$ and $n = 2r$, $n$ being the distance travelled by $p$ per time step $t$.
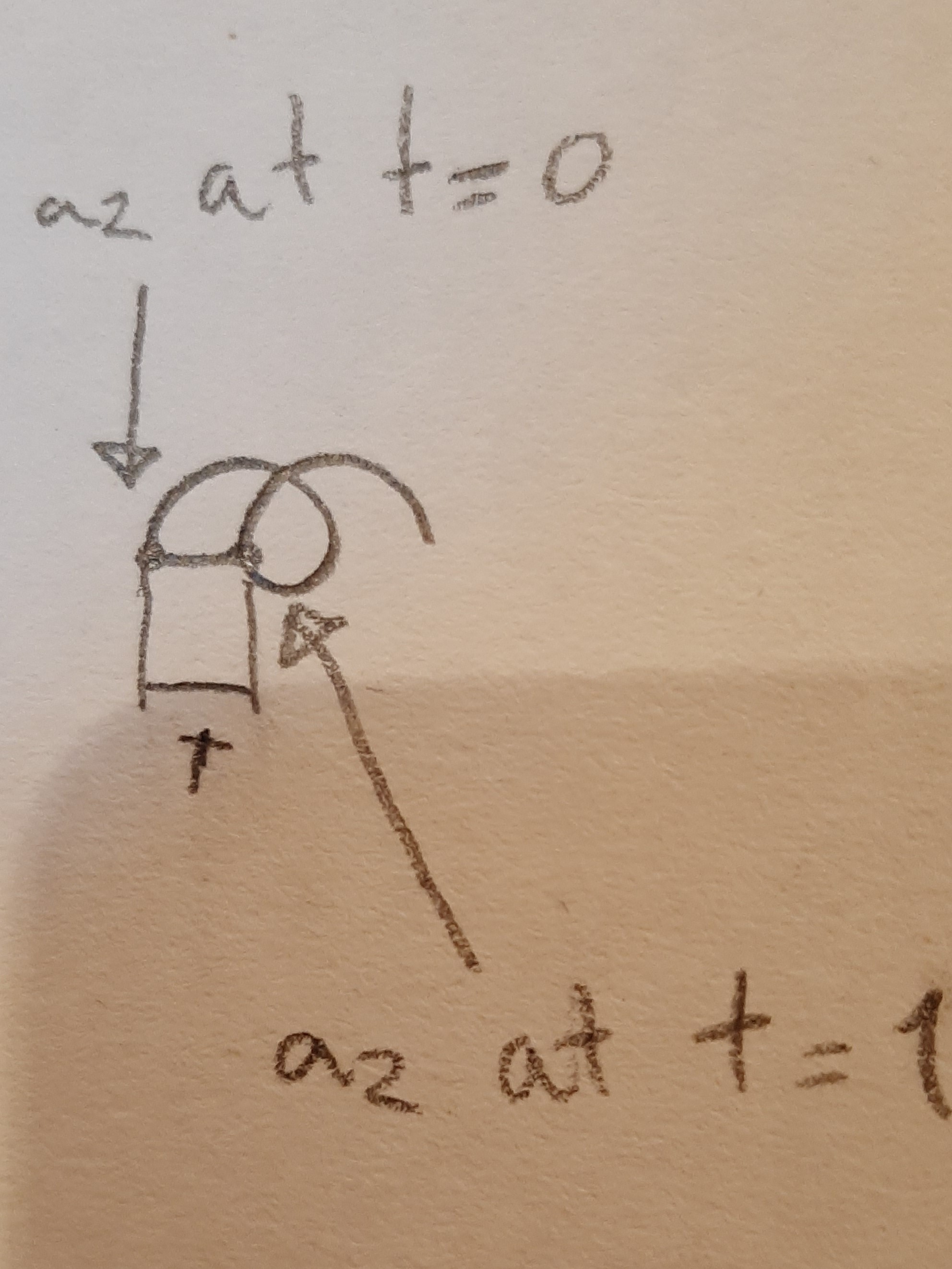
Here, $n = r$.
The question:
The thing is, whenever I look at pictures where nutation is illustrated, the path of $a_2$ never loops; it's sinusoidal (see this Wikipedia article for an example). So, that makes me think that nutation for some reason requires $n \ge 2r$. However, this belief is based on my current (mis)understanding. So, this leaves me with two questions:
- Is my understanding correct?
- If so, why is $n \ge 2r$?