Newtonian contribution to perihelion precession of Mercury

Question

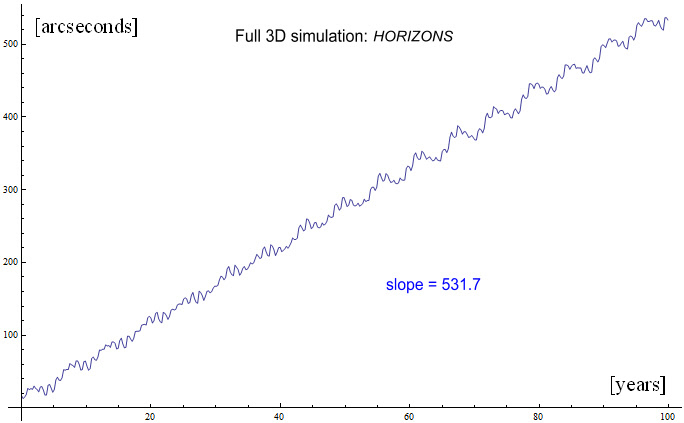
We have seen that the orbits of the planets around the sun can be found, to a good approximation, by only considering a two-body system (a planet and the sun) interacting through a central force. However, all the other planets cause slight variations in the predicted path of an individual planet. Astronomers have been able to calculate the expected precession of Mercury’s apsides (due to the forces of other planets) to be 531 arcseconds per century, and they have observed that the actual precession is 574 arcseconds per century. This leaves a difference of 43" that could not be explained by uncertainties in calculation or measurement and which was noticed as early as 1845.

Source: https://www.tau.ac.il/~morris/03411203/chapter3/mercury_paper.pdf

As we can see from the quote above that the precession of mercury had been calculated to an amazing degree of accuracy well before Einstein's theory of general relativity. To account for the difference between the calculated value and the one predicted by Newton's theory, they came up with mistaken ideas of Vulcan and dust clouds. It was Einstein's theory which gave a final answer. The orbits of all other planets also precess but the value is too small and astronomers in the past didn't notice it for other planets other than Mercury.

Perihelion is the closest point to the sun while the aphelion is the farthest for any orbit.

My question is about the Newtonian precession of 531 arcseconds per century. Suppose, we are looking at the picture shown below from above the solar system. It could be assumed that all the planets lie in same plane. Looking from the top, one would say that precession is taking place counterclockwise as if some force is gradually pulling the entire orbit of planet Mercury in counterclockwise direction.

Source: https://www.astronomicalreturns.com/2020/05/the-mystery-of-mercurys-missing.html

The Newtonian precession of 531 arcseconds per century was the result of gravitational attraction of other planets onto Mercury. The picture below shows the relative sizes of the planets and their distance from the sun. I think that Mercury should be affected most by Venus, Earth, Mars, and Jupiter since these planets are relatively close to Mercury's orbit. Mercury takes almost 88 days to orbit the sun.

Source: https://www.wikihow.com/Draw-the-Solar-System

As I said earlier, it appears as if Mercury's orbit is being pulled in counterclockwise direction which suggests, at least to me, that mostly other planets were in such a configuration that their resultant pull onto Mercury was in the counterclockwise direction. If it was not true then there would be times when Mercury's orbit could precess or slide in clockwise direction as well. The diagram below is a crude attempt to show you how I'm thinking. The orbit in cyan is for Mercury, the orbit in red is for Venus, and so on. The arrows show the pull of shown planets onto Mercury.

Could you please let me know if I'm thinking along the right lines? I'm trying to understand it intuitively.

Answer 1

You are essentially correct to assume that the precession is sometimes backwards as well. The frequently quoted value of 531"/cy is only the long term net average. In reality there is a considerable oscillatory component added to this, as illustrated in the following schematic figure

The oscillation period here is essentially the orbital period of the disturbing planet. The effect is exaggerated here just to demonstrate it. If you do the same calculation with the actual data for all planets, you get the following graph for Mercury's Newtonian perihelion precession

Although the increase of the perihelion angle is now closer to a linear increase, there is still a considerable wobble, and at times it is going backwards for a few years. The two main periods in the oscillation visible here are those of Jupiter and Venus, which have the largest impact on the precession (Jupiter due to its mass and Venus due to its proximity)

For more details see the web page Mercury's Perihelion Advance from which also the above figures were taken

---

EDIT: Let me add a qualitative explanation of what is happening here:

an outer planet in opposition to Mercury (i.e. on the same side of the Sun) will result in a small force pulling it away from the Sun. So if Mercury is moving away from the Sun again after its closest approach (perihelion) the gravitational force of the Sun will effectively be reduced slightly and it takes longer to bring the radial velocity back to zero again. This means Mercury will go slightly further in its orbit until it has reached its furthest point (aphelion) i.e. a positive precession. If however the disturbing planet is on the opposite side of the Sun, its gravitational field will effectively increase the gravitational field of the Sun, so the effect will be the other way around, leading to a negative (backwards) precession. In the latter case the planet is further away though than in opposition, so the effect is smaller, leaving an overall positive (forward) precession on average. The net result can therefore be seen as an oscillatory precession angle (related to the time dependent orbital motion of the disturbing planet) superposed on a linearly increasing precession angle related to the time averaged mass distribution of the planet (which is effectively a circle/ellipse and thus always produces an outward directed force i.e. a positive precession)