Why is $J^P=0$ for all even-even nuclei?

Question

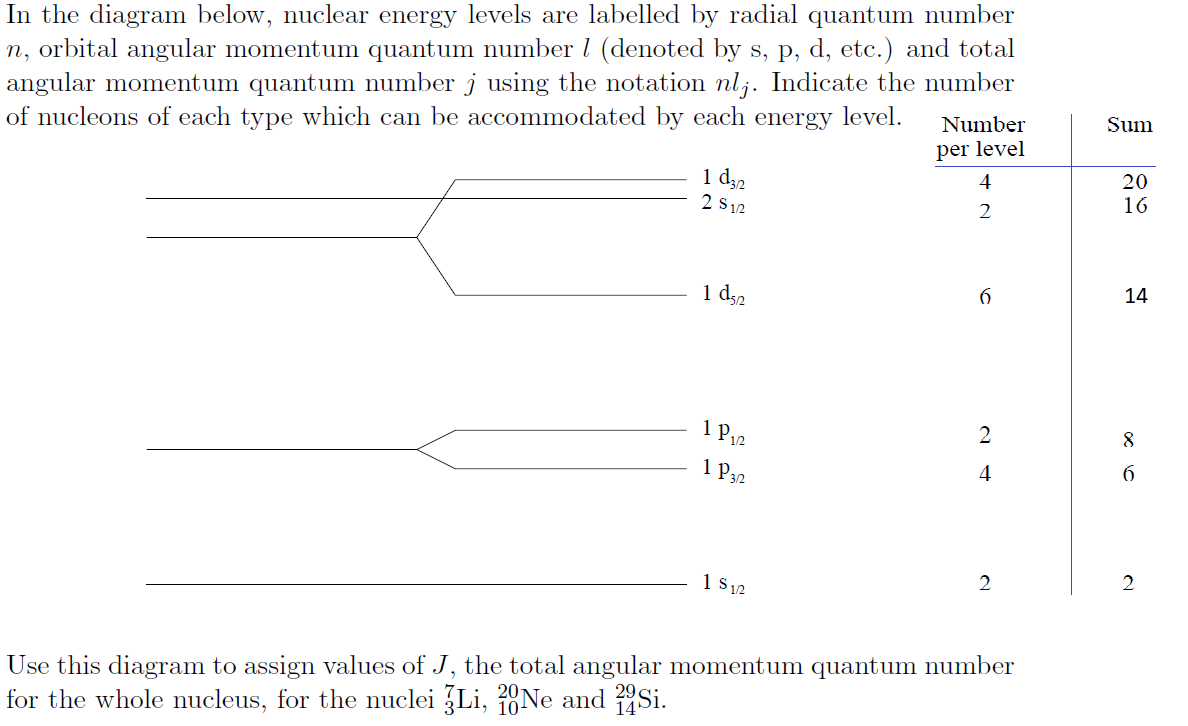
I have the following question, for which I have answered fully, but I am questioning the logic behind finding the value of $J^P$ for ${}^{20}_{10}\mathrm{Ne}$:

N.B, when I wrote "Number per level" in the picture above this is really just the degeneracy, $2j+1$.

Now to answer the question at the bottom for ${}^{7}_{3}\mathrm{Li}$, ($Z=3\,$ & $N=4$) since there is one unpaired proton, it will go in the $1p_{3/2}$ level and since the subscript is the value of $J$, then $$J^{P}=\frac32^{-}$$ where I used a minus sign in the superscript since the parity $P=(-1)^{\ell}$ and $\ell=1$ for a $p$ state.

Onto ${}^{29}_{14}\mathrm{Si}$, ($Z=14\,$ & $N=15$) there is only one unpaired neutron, using the table I added this will go in the $2s_{1/2}$ level and therefore $$J^P={\frac12}^{+}$$ now, with a positive sign since $\ell=0$ for a $s$ state ($P=(-1)^{0}=1$).

I left the ${}^{20}_{10}\mathrm{Ne}$, ($Z=10\,$ & $N=10$) to the end on purpose as although I note that all nucleons are 'paired off', looking at the table the $10$th proton (or neutron), ought to go in the $1d_{5/2}$ level and hence $$J^P={\frac52}^{+}$$ parity is $+1$ here as $\ell=2$.

But this is incorrect and the correct answer is actually $$J^P=0$$ But, from the logic I just showed this does not make sense.

${}^{16}_{\,8}\mathrm{O}$ is another example when $N=Z$ (an even-even nucleus), with $J^P=0$.

Am I just to accept that for some (unknown) reason $J^P=0$ for all even-even nuclei? So in summary; why can't I just use the table (I inserted in the picture above) to determine the value of $J^P$?

Answer 1

I'll answer by asking you some questions:

For $^7_3$Li, why did you ignore the 4 neutrons? Why did you ignore the two $1p_{3/2}$ neutrons? You mentioned that the third proton was "unpaired."

For $^{29}_{14}$Si, why did you ignore the $14$ protons and their contribution to spin and parity? Again, you mentioned there was an "unpaired" neutron.

Maybe you should consider the idea of pairing, in the ground state, to be balancing the angular momentum contribution of nucleons, so that paired nucleons contribute zero, and the parity is even, regardless of the particle state.

The basic rule for ground state nuclei is that pairs of neutrons or pairs of protons occupy the same $\ell,j$ state with opposite $m_j$ values and contribute 0 to the total J.

You should have said "there are no unpaired nucleons for $^{20}_{10}$Ne, so the ground state angular momentum will be zero."