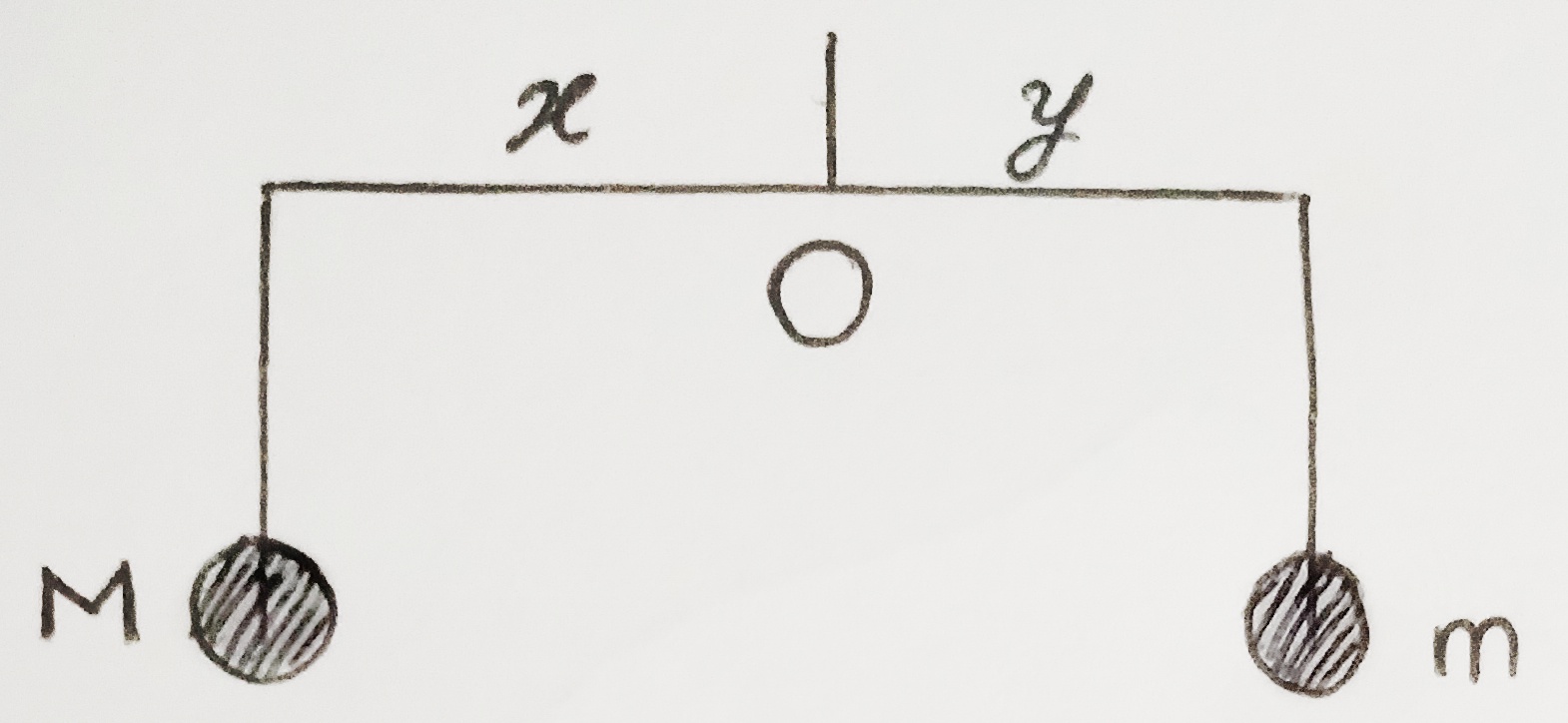Why isn't this rod in neutral equilibrium?

Question

Suppose that the rod shown below in figure(1) is balanced. So the torque about point $O$ is $Mg.x-mg.y=0$.

[figure(1)]

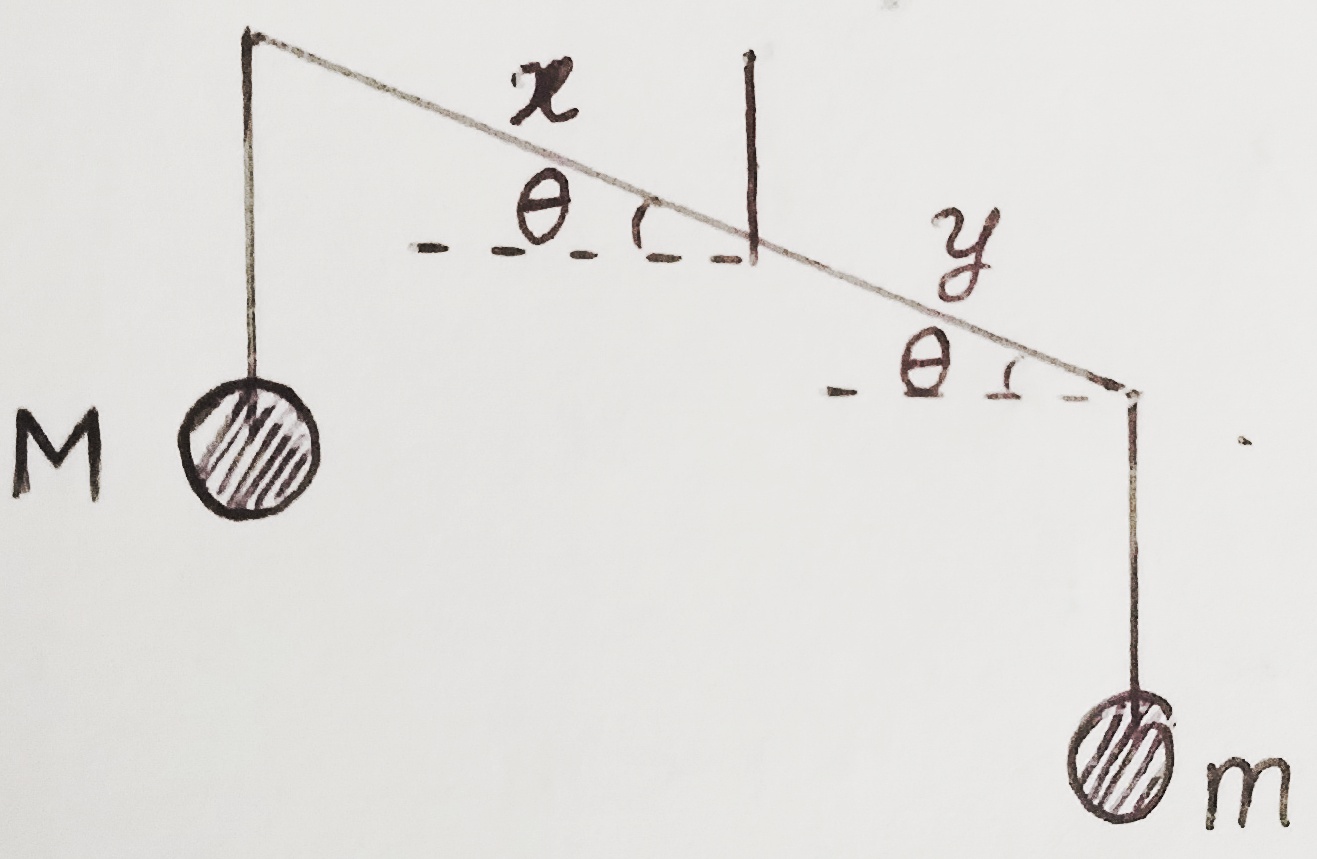
Then imagine than M is lifted a little bit up making an angle of $\theta$ with the horizontal plane(figure(2)).

[figure(2)]

Then let's calculate the torque about point $O$.

Torque about point $O$ $$= Mg.x cos\theta - mg.y cos\theta $$ $$ =cos\theta(Mg.x-mg.y)$$ from the first equation:

$$=cos\theta.(0)$$ $$=0$$ Also we can find the torques about both ends of the rod are equal to zero. So shouldn't it be balanced in its new position?

And when talking about potential energy of each mass, when we lift one side up its P.E. is increased as well as the other one's P.E. is decreased. We can imagine that both of them cancel each other( This is obvious when $M=m$ and $x=y$).*

Thus shouldn't it be in neutral equilibrium?

But as we experience in day-to-day life balances always find a horizontal plane to be balanced. So what is wrong with my previous explanation?

Edit: The example of a beam scale may be irrelevant here. But I tried to balance such a rod and I couldn't keep it in neutral equilibrium. Is that because both masses are trying to keep their gravitational potential energy at a low level? Though this contrast again with above paragraph marked as *. Does it imply that these two masses do not act as a system?

Answer 1

To elaborate on R.W. Bird's excellent and correct answer: if the masses are not suspended precisely in a horizontal line with the suspension point of the bar, then the horizontal position is a stable equilibrium configuration rather than a neutral equilibrium configuration. To see this, let's first draw a "thick" rod in horizontal equilibrium:

The cross here marks the pivot point. As you (correctly) showed, the condition that this be an equilibrium configuration implies that $M g x = m g y$.

Now let's tilt the beam a bit:

The lever arm for the left-hand force is $x \cos \theta + d \sin \theta$, while the lever arm for the right-hand force is $y \cos \theta - d \sin \theta$. Thus, the total torque exerted about the pivot point (with clockwise rotations taken to be positive) is $$ \tau = -M g (x \cos \theta + d \sin \theta) + m g (y \cos \theta - d \sin \theta) = -(M+m) g d \sin \theta $$ So for any positive value of $d$, a positive displacement in $\theta$ leads to a negative torque, which restores it towards $\theta = 0$. Similarly, a negative displacement in $\theta$ leads to a positive torque, which also restores the equilibrium. Thus, the horizontal configuration is not a neutral equilibrium unless $d = 0$, i.e., the suspension points of the masses are perfectly horizontally aligned with the pivot point.

Note that the above also implies that if $d < 0$, the horizontal configuration is an unstable equilibrium. This would correspond to the case where the mass suspension points are (for whatever reason) above the pivot point.

Answer 2

With a balance beam scale, the point of support is shifted slightly up (think of the beam as being a little bent). Then the effective horizontal becomes a position of stable equilibrium (if the loads are equal).

Answer 3

Your calculations are correct, the rod will be in equilibrium regardless of the angle. This assumes that all the joints are pin joints, so that the joints do not transmit any torques, and that the vertical rods stay vertical.

A horizontal plane may be more aesthetically pleasing, but as anyone who has tried to hang a picture can tell you, objects can be in equilibrium without being horizontal.

Answer 4

Your calculation is correct. This is because the center of gravity of your system is always under the axis of rotation and don't move laterally when you rotate the system. The same goes for a wheel that turns around an axis that passes through the center of gravity.