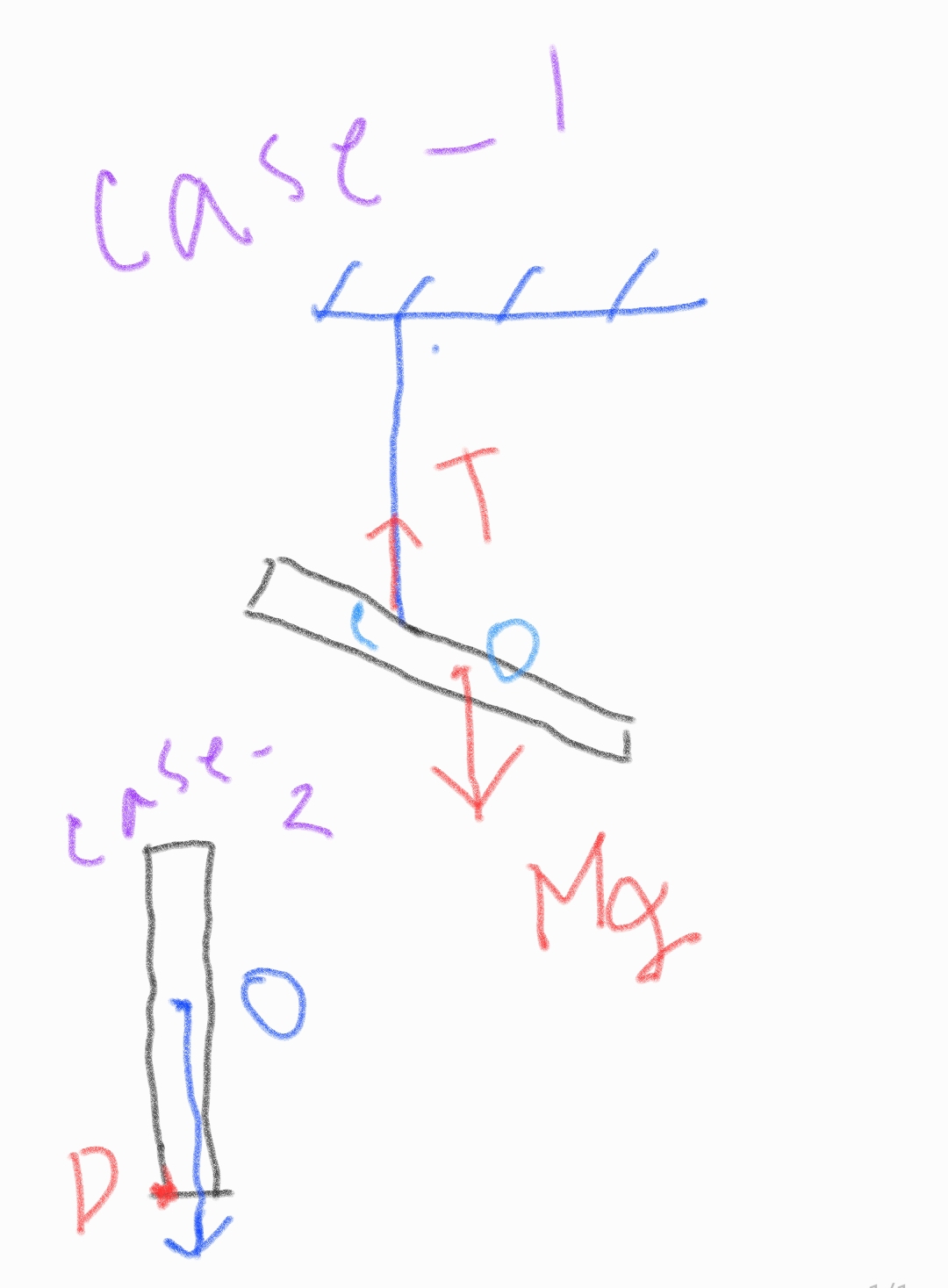
I have two silly queries:
In case $1$,a rod $AB$ is attached to a string at point $C$. Here the weight is acting at the center of mass $O$. My question is: * Is it even possible to keep a rod hanging like this*? If it were possible,then the rod is in rotational equilibrium,but as we can see the line of action of the weight is not going through $C$. So,there should be net torque with respect to $C$. In light of this logic,how can a rod keep hanging in this position?
In case $2$,we imagine a cylinder whose cross sectional area is large enough standing on ground. Now we all know the center of mass $O$ is at the center of the cylinder. As the body is in rotational equilibrium, the net torque with respect to any point should be $0$. But here, the line of action of weight that is acting at $O$ does not pass through $D$. So,there should be a net torque about $D$. But,still we don't see the cylinder falling down with respect to $D$ even if there is a net torque. Why does this happen?
I will be grateful for clearing my doubts.