Theoretical explanation for why torque is dependent on the lever arm? [duplicate]

Question

We know that the formula of torque is $\tau=Fl$, where $l$ is the lever arm of the force $F$. I am wondering why is torque dependent on the lever arm? I suspect that it's initially concluded based on experimental data? If so, I am also wondering if there's a theoretical explanation that addresses from the first principle why the value of torque depends on the length of the lever arm?

Answer 1

This is a good learning moment, because you are asking a type of question that will come up a lot in your studies of physics, where you need to know what kind of answer will make sense.

In general terms, the question you are asking is, "Why is $X$ defined the way it is?" In this specific case, $X$ is the torque, but this comment is more general. This kind of question can't be answered by appealing to an experiment. It is not the case that we discovered a "torque-meter" in the lab and then we need to work out what it is measuring. We decided that torque is an interesting quantity, for some reason, and then built measuring devices to measure torque because we decided it was interesting.

So, the kind of answer you want to this type of question, is: "what special property or properties does torque have, that makes it an interesting quantity to define?" There are an infinite number of combinations: $F l, Fl^2, Fl^3, ...$, why is $Fl$ so special?

This is actually quite a deep question and I am only going to scratch the surface. The motivation is to think about the rotation of a rigid body around a given axis. If we have a symmetric object like a cylinder, and the object is rotating about an axis of symmetry (like the center of the cylinder), then the angular acceleration of the body is related to the torque via \begin{equation} \tau = I \alpha \end{equation} where $\tau=Fl$ is the torque and $I$ is the moment of inertia. This equation is interesting because it is very similar to Newton's law $F=ma$, and as a result a lot of the intuition you have built from studying forces, also applies to torques. For example: just like how an object at rest has no net forces applied, an object that is not accelerating in its rotation has no net torques applied. As one application fo this -- a top spinning on a frictionless table with its axis completely vertical has no torques applied. This is quite interesting and not obvious because there are certainly forces acting on individual atoms making up the top -- after all each individual piece of the top is accelerating (since it is moving in a circle), but somehow we have been able to meaningfully combine all the pieces of the top into a single object whose angular acceleration is zero. I won't go through a derivation of this law, but it follows from Newton's laws and the assumption of a rigid body, and the torque naturally falls out of the derivation. This definition turns out to be incredibly useful, and can be used to solve many complicated problems related to rotating bodies, that would be very difficult to solve directly in terms of forces.

Answer 2

I'd have said that this is the definition of torque. The theorems relating torque to the rate of change of angular momentum follow from $F=ma$ and this definition.

Torque is defined this way so that the resulting theorems are simple and useful.

Answer 3

The relevance of a property related to multiplying the force by the lever arm comes from the experience with one of the oldest tools of mankind: the lever.

Answer 4

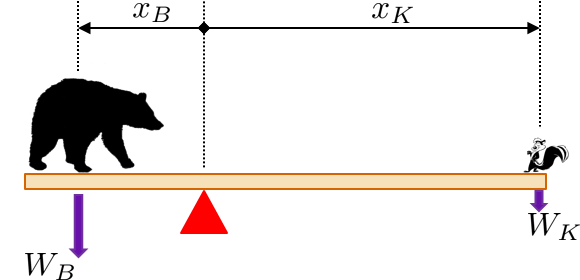
In this answer to a similar question I approached this with statics, by introducing the concept of "balance".

To quantify the idea of "balance" you need a description of the weight of each object as well as how far away does this weight applies relative to the fulcrum (red triangle).

You equate the moments

$$ \text{(moment of bear)}=\text{(moment of skunk)} $$ $$ x_B\; W_B = x_K\; W_K $$

_{where xB and xK are the distances (of the bear and the skunk respectively), and WB and WK the weights (of the bear and the skunk respectively).}

But to really answer this question, on a bit more fundamental level, I want to introduce the concept of line of action of a force.

Torque is just a special case of a moment. Specifically the moment of a force. In the diagram below you see the same force (vector) $F$ acting along some line in space that is offset from the coordinate system we use.

The moment of the force as seen felt by an observer at the coordinate origin does not change when you slide the force vector along its line of action. The effect is the same. This effect has the quality of twisting (light pink curved arrow) and the further away the force is, and the stronger the force is the stronger this twisting.

Mathematically this twisting is described by the moment vector (darker pink arrow) and it is defined as the cross product between the position vector $\boldsymbol{r}_P$ and the force vector $\boldsymbol{F}$

$$ \boldsymbol{M} = \boldsymbol{r}_P \times \boldsymbol{F}$$

The cross product is a special multiplication operation that filters out any components parallel between the two vectors. The result in magnitudes is

$$ \| \boldsymbol{M} \| = d \; \|\boldsymbol{F}\| $$

where $d$ is the perpendicular distance to the line of action of the force. The quantity $d$ is purely geometrical in nature.

The moment of a force is a quantity that describes how far away a force is acting from the coordinate origin, as in $d = \| \boldsymbol{M} \| / \| \boldsymbol{F} \|$

Summary

• We use the perpendicular distance for torque (moment of force) because force vectors can be moved freely along their line of action.
• We use the cross product the evaluate moments exactly because they ignore parallel components and only consider the perpendicular distances.

You can go a level deeper by reading this answer and understand that the above applies not only to the moment of forces $M = r \times F$, but also the angular momentum (moment of momentum) $L = r \times p$ and even velocity (moment of rotation) $v = r \times \omega$.

All three situations above are examples of situations where an action is performed along a line in space that is offset from the origin. This line might be called the line of action, or the percussion axis, or the rotation axis, but the underlying geometry of this situation is the same.