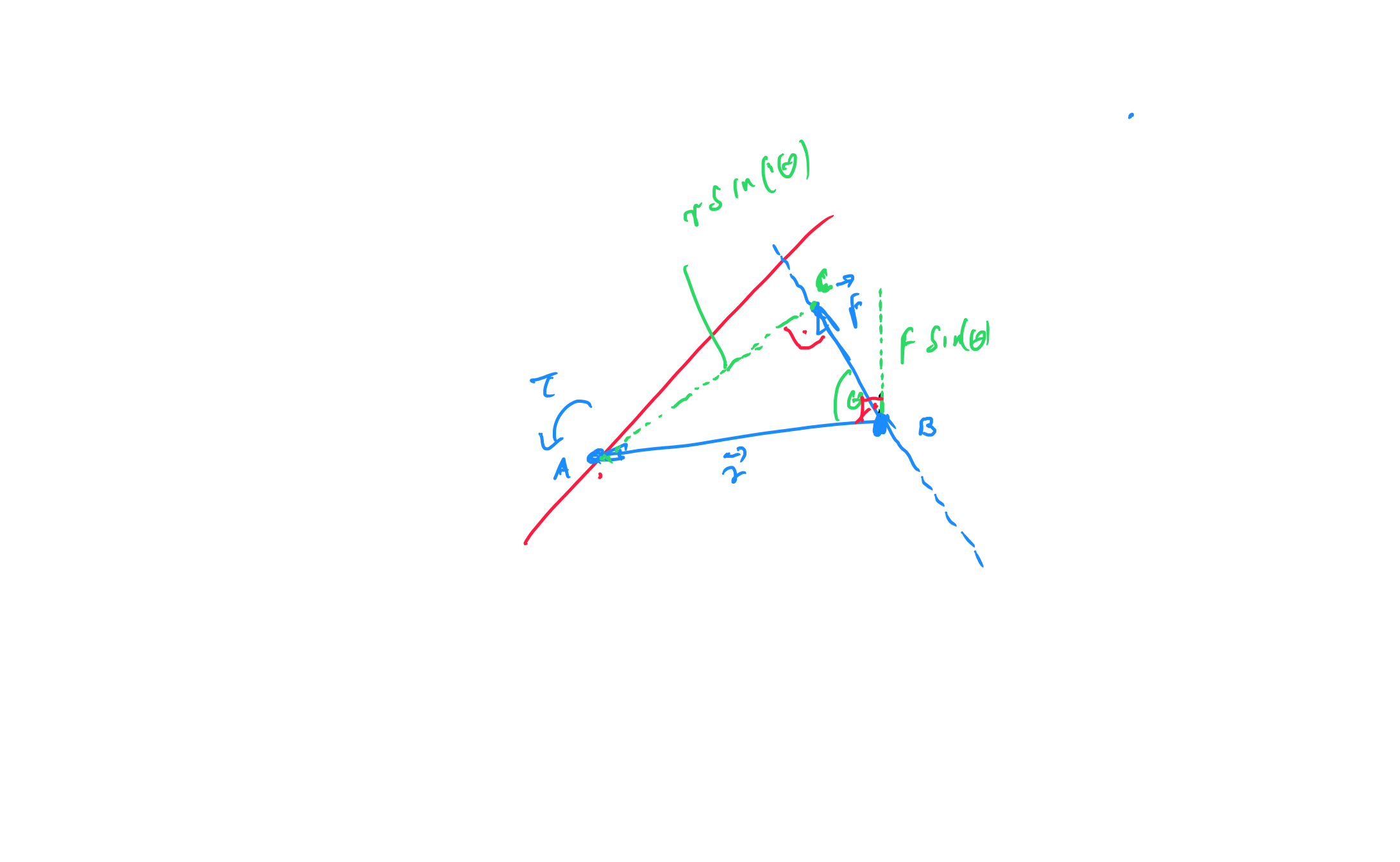
Why do we use the lever arm concept?

Question

Why do we use lever arm? Torque is $\tau = \vec{r} \times \vec{F}$ or $\tau = rF\sin(\theta)$, but why when explaining what we mean do we choose to imagine a straight line to where the force would be perpendicular (essentially taking the "component" of $\vec{r}$, which is the distance straight from the rotation axis) instead of just finding the perpendicular part of the force and leaving r as the distance from the axis to the point of application of the force? Is there a practical situation in which the lever arm concept leads to something the component of the force concept does not?

I teach intro courses (calc and algebra based). Every student I have ever worked with has found breaking up the force easier to understand, because they are quite used to doing that and what it means by the time we get to torque. Many of them find the idea of taking the "component" of an object and "applying the force in the middle of space" to be very confusing, and they are already having to get use to being careful about where they put the force on their diagrams suddenly.

The two descriptions are mathematically equivalent, so why do we insist on teaching using the more complicated concept? In particular, I see this more in Algebra based textbooks such as Cutnell.

Answer 1

For me, the concept of line of action of a force is important to introduce first. Then you can talk about the perpendicular distance of this line to the measuring point (origin). Now the concept of moment arm is introduced in terms of geometry only.

You can then indicate similarity with the "moment arms" of other important lines in physics, the rotation axis, and the percussion axis. Just like torque is evaluated by $\vec{r}\times \vec{F}$, velocity is $\vec{r} \times {\omega}$ and angular momentum $\vec{r}\times \vec{p}$, all being "moment of" something.

Finally, you can show that although numerically equivalent $(r \sin \theta) F$ and $r (F \sin \theta)$ the interpretation differs. The first one shows that the force (and its magnitude as a whole) are important, and only the perpendicular distance counts. It is ok to dilute the location information and not ok to dilute the force.

The reason for this asymmetric treatment for the cross product $\times$ is physics/geometry as any point along the line counts the same, and you can slide a force along its line of action without consequences. The force stays the same, but the location can change as long as the perpendicular distance remains the same.

---

References:

• Answer on the nature of torque posted here.

Answer 2

We want to obtain the torque $~\tau~$about point A .If you look at the figure :

Case I

find at point B the component of the force $\vec{F}$ that is perpendicular to $\vec{r}$ which is $F\,\sin(\theta)$ thus

$$\tau_A=F\,\sin(\theta)\,r$$

Case II

find at point C the component of the force $\vec{r}$ that is perpendicular to $\vec{F}$ which is $r\,\sin(\theta)$ thus

$$\tau_A=r\,\sin(\theta)\,F$$

I agree with you that case I is more "intuitive" to obtain the torque $\tau_A$ then case II.