When you touch two spheres, you can consider them as one system, in other words, one big conductor. Now, if a conductor has different potentials on either side, then current (charges) flows through it from higher potential to lower potential.
This happens as a system always wants to be in the lowest energy state, hence it will try and minimize potential energy by redistribution of charges.
If we talk about net electric field, then it can be zero at only one point between the spheres, it is non zero everywhere else.
Assuming distance between the spheres as d, we get:
$\frac{kq{1}q{2}}{r^{2}}=\frac{kq{1}q{2}}{\left(d-r\right)^{2}}$
where r is the distance from one sphere, upon solving we find only 1 value of r for which the net field is zero.
Since net field is zero at only one place, the charges can still move under the influence of non zero electric field elsewhere.
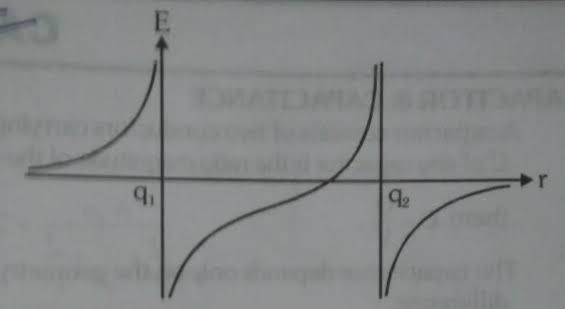
Consider the equation $E=\frac{dV}{dr}$ written in different format:
$V=\int_{ }^{ }E\cdot dr$
now, V is the integral of E•dr, hence it is the area under the curve of electric field vs distance. In such a situation E can be negative and positive, depending on direction, which means that the net area under the curve can be zero. This doesn't imply that E is 0 everywhere, it just means the positive area cancels with negative area