0
$\begingroup$

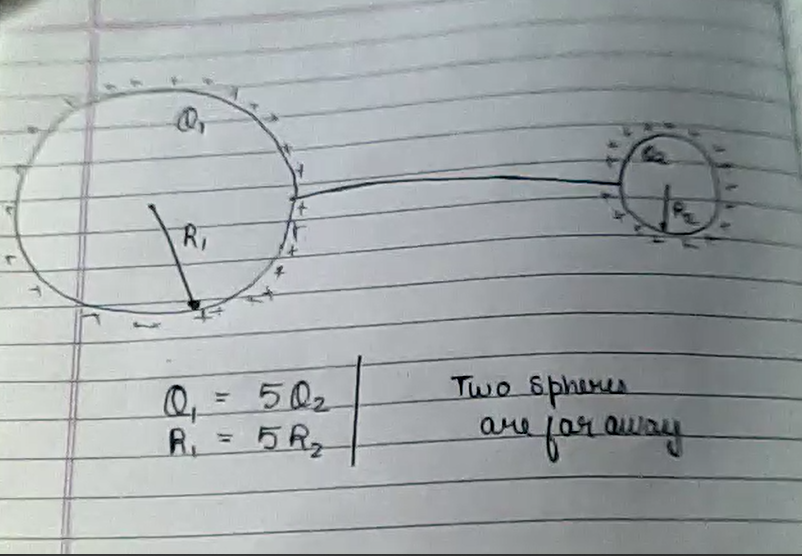
Two conducting solid spheres are kept apart, one with charge Q1 and radius R1 and the other with charge Q2 and radius R2.

Q1=5Q2 and R1=5R2

When these spheres are connected by a conducting wire, the potential at both ends of the wire is the same.

enter image description here

I understand that the electrons will arrange themselves in such a way that the electric field inside the conductor will be zero.

However, electrons closer to the spheres would be attracted to them in this arrangement.

The question is why, despite being attracted to positive charges on the sphere, they (electron nearer to the surface of spheres) did not leave the wire and enter (flow through) the conductor (sphere), given that spheres are far apart

What's keeping it from happening?

A thoughtful response would be greatly appreciated.

Improve this question
$\endgroup$
4
  • $\begingroup$ You should not assume the wire is electrically neutral. $\endgroup$
    – The Photon
    Commented May 19, 2022 at 13:37
  • $\begingroup$ It's hard to figure out what you are asking. When there's no electric potential, there's no current flow. That doesn't mean there's no free charge in the wire, just that nothing is moving. $\endgroup$
    – Carl Witthoft
    Commented May 19, 2022 at 13:41
  • $\begingroup$ The electric field inside the conducting wire is zero due to electron rearrangement BUT the potential on each individual sphere is not zero, the difference is zero, and the NET current is zero, so the electrons near the sphere must be attracted to it, but they did not migrate to the sphere, why? that is the issue $\endgroup$
    – TPL
    Commented May 19, 2022 at 14:26
  • $\begingroup$ To add onto other answers, the electrons will migrate to the edges of the wire. In this transient state "current" flows. However quickly, the field from electrons cancels out the original field in the wire $\endgroup$
    – jensen paull
    Commented May 19, 2022 at 15:47

2 Answers 2

Reset to default
2
$\begingroup$

The electric field inside the conducting wire is zero due to electron rearrangement BUT the potential on each individual sphere is not zero, the difference is zero, and the NET current is zero, so the electrons near the sphere must be attracted to it, but they did not migrate to the sphere, why?

You should not assume the wire is electrically neutral. It is not. If it were, you wouldn't have an equipotential over the whole conductive region. You'd just have exactly the same potential distribution you had before you connected the wire.

So the electrons did migrate, when you first connected the wire, from the wire onto the two spheres. Now that the system has come to equilibrium, there's no longer a potential difference along the wire to drive the electrons to move further.

Improve this answer
$\endgroup$
4
  • $\begingroup$ So, did the sphere's charges change? $\endgroup$
    – TPL
    Commented May 19, 2022 at 18:05
  • $\begingroup$ @TonyPhysicslover, yes, but (presumablyl) by an insignificant amount. $\endgroup$
    – The Photon
    Commented May 19, 2022 at 18:11
  • $\begingroup$ Your response was exactly what I was searching for, however could you tell me if there is any supporting material/documentation? $\endgroup$
    – TPL
    Commented May 19, 2022 at 18:19
  • 1
    $\begingroup$ @Tony, you can calculate the potential distribution before the wire is connected using Guass's law and superposition. You can see there is no equipotential line between the two spheres. You know that when the wire is connected there must be an equipotential line between the two spheres. The only way for this to happen is for the wire to have some charge. With a field solver you could work out the details of how much charge is required on each element of the wire. $\endgroup$
    – The Photon
    Commented May 19, 2022 at 18:56
-4
$\begingroup$

If there is no potential difference, there is no electric field, so there is no force to the charges. tej electric field between four two spares os zero at any point far away or near the sphere -

Improve this answer
$\endgroup$
1
  • 1
    $\begingroup$ Wrong, a PD of zero across the wire doesn't mean there is zero field on all points in the wire. $\endgroup$
    – jensen paull
    Commented May 19, 2022 at 15:48

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.