Centripetal and centrifugal acceleration in an athletics raceway (for example)

Question

I consider this question and the answer of the user @Bob D in this link: Centripetal and Centrifugal acceleration/force. When an athlete runs round a curve in the atletic track he will be subject to centripetal acceleration and centrifugal acceleration, and I also believe that the motion will be circular and non-uniform.

I know that,

$$\mathbf{a}_{\text{cp}}=\frac{v^2}r, \quad \mathbf{a}_{\text{tan}}=\alpha r$$ where $\alpha$ it is the angular acceleration. Easily the total acceleration is:

$${a}=\sqrt{a^2_{\text{cp}}+a^2_{\text{tan}}}$$

Why (with the formulas) the centrifugal acceleration must coincide exactly with centripetal acceleration? Isn't centrifugal acceleration a tangential acceleration hence $\mathbf{a}_{\text{tan}}=\alpha r$?

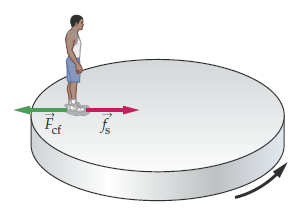
Addendum: I add any image for clarifications by the comments.

Comment for the picture: An observer on a rotating platform rotation feels a force that pushes it outward and, to remain stationary, it must exploit the friction with the floor. Since the weight force and the normal reaction of the floor cancel each other out, the only real force on the observer is the static friction force $\mathbf{f}_s$, which acts as centripetal force. We must then suppose that in the reference system of the platform another force appears, the centrifugal force $\mathbf{F}_{\text{cf}}$, which has the same intensity and the same direction as the centripetal centripetal force, but opposite direction.

Answer 1

Why (with the formulas) the centrifugal acceleration must coincide exactly with centripetal acceleration?

In an inertial frame, the centripetal force is simply the component of the net force directed to the center of rotation.

Consider the classic string with a mass attached at the end. Assume no gravity, such that the only force acting on the mass is the tension $T$. Now if the mass is to undergo circular motion in a perfect circle of fixed radius $r$, you know that the net force directed inwards (i.e. centripetal force) is $$F_C = \dfrac{mv^2}r$$

Now imagine this object rotating and you cut the string. I made an animation here: https://www.desmos.com/calculator/s8roerbvub. At first, the object is flying radially outwards. This is simply due to the object's inertia -- it continues moving with its given velocity.

Imagine you're in a car. You take a sharp left. Approximate the turn as perfectly circular. Even though you end up turning left, your body feels as though it "wants" to go right, outwards. After all, if you're leaned against the door, you will feel the door exert a force on you. The force would be the normal force, providing the necessary centripetal force to keep going in that circle (also friction, but we can ignore that for this purpose). The conclusion is that $F_C = N$

Now, let's pick a non inertial frame of reference where you're not accelerating. That would be right inside your car. Since you're not accelerating, the net force must be zero. But the door is pushing on you with some force $N$ to the left. But you're not accelerating in that frame, therefore there must be some other force to balance it out, pushing you to the right. That "other force" is the fictitious centrifugal force. By Newton's second law, you get that $F_{fictitious} = N$, but you know that $N=F_C$, which means your fictitious centrifugal force is equal in magnitude (but opposite in direction) to the centripetal force. Same goes for acceleration.

Answer 2

Another way to look at it is thinking of the centrifugal force as a real force, but acting on the ground, not on the runner. It is part of the horizontal component of the force that the runner does on the track. The ground reacts with a centripetal force on the runner, since there is enough friction between shoes and track.

The other horizontal part is the tangential force. For the foot behind the body, that force on the ground is backward and the reaction of the ground accelerates the runner. For the foot ahead the body, the force on the ground is forward, and its reaction decelerates the athlete. So it can make the curve keeping the modulus of velocity constant.

Answer 3

If you are viewing the system from an inertial (non-accelerating) frame of reference, then there is no centrifugal force. The centrifugal force has nothing to do with the motion of the system and everything to do with the reference frame. If you are viewing the system from a rotating reference frame, then you will have to conclude one of two things (not both):

1. Newton's second law is broken: accelerations are being affected by something other than a force
2. Newtons third law is broken: forces are present that do not arise from interactions.

The centrifugal force is the result of the latter option: it is a pseudo-force that has no "equal but opposite reaction".

Furthermore, contrary to other answers, it need not be equal to the centripetal force. For starters, there doesn't even need to be a centripetal force to have a centrifugal force because, as I said before, the centrifugal force only depends on the frame of reference. But even in the case of uniform circular motion, the centrifugal force only equals the centripetal force in the case where the frame rotates with the object undergoing circular motion.

And finally, the tangential component of the force is not the centrifugal force; it is a real component just like the centripetal force is.