1
$\begingroup$

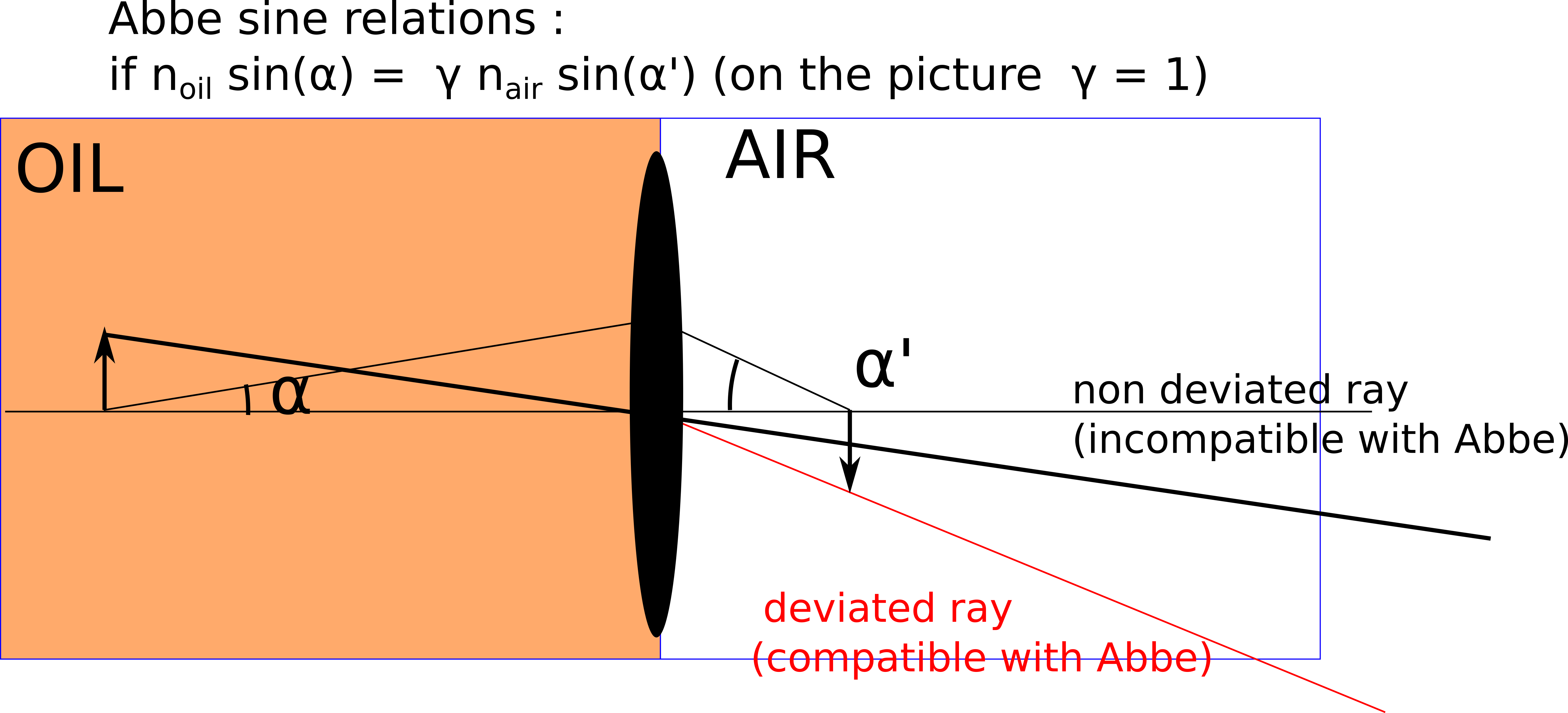
I can not find out the right conjugation relation for an oil immersion objective (oil in the object space, air in the image space). If I send an incident plane wave in air with angle $\alpha_{air}$ with regard to the optical axis, its angle in oil $\alpha_{oil}$ will be smaller because of refraction. Where will focus the plane wave in the rear focal plane of an oil immersion objective with focal $f$ ? At the coordinate $f \tan(\alpha_{air})$ or $f \tan(\alpha_{oil})$ ? The question is illustrated in the image below. 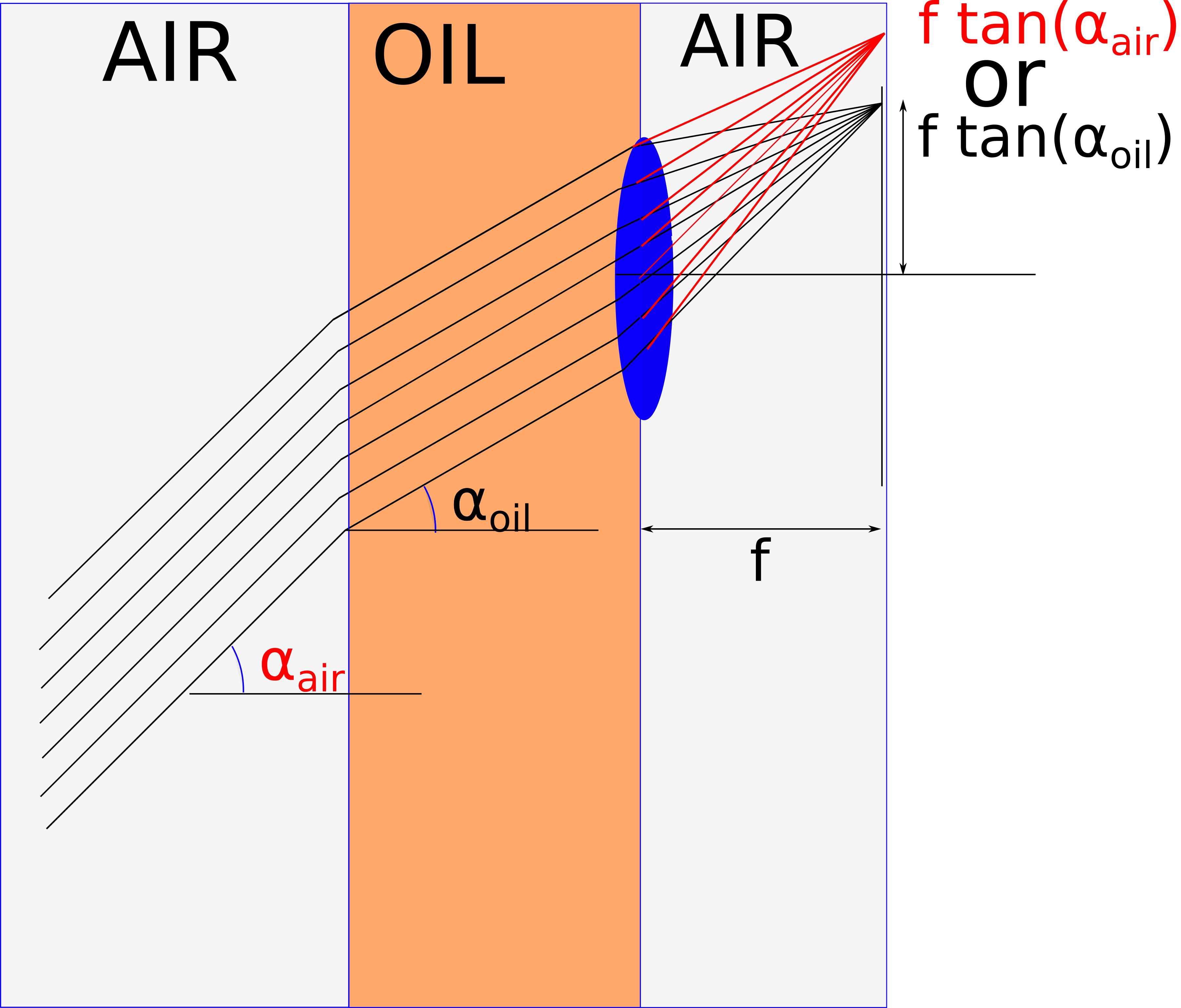OIL/AIR/ANGLES

Edit : The first intuition is that the ray passing through the lens is not deviated. However, this is incompatible with Abbe sine relation, as shown in the picture below. Abbe sine relation should be verified by an aplanetic objective (that is not at a thin lens). enter image description here

Improve this question
$\endgroup$
2
  • $\begingroup$ Is there a mistake between $\alpha_{\mathrm{air}}$ and $\alpha_{\mathrm{oil}}$ in your drawing? $\endgroup$
    – QuantumApple
    Commented Nov 3, 2020 at 21:35
  • $\begingroup$ Yes, sorry, I just corrected it. $\endgroup$
    – magtweezers
    Commented Nov 4, 2020 at 12:05

1 Answer 1

Reset to default
2
$\begingroup$

I think that the former ($\tan(\alpha_{\mathrm{air}})$) is correct. The ray going through the center of the lens shouldn't be deviated by the lens, and if you can neglect the lens, then the outgoing angle is indeed $\alpha_{\mathrm{air}}$ (the same as the incident angle in air) because of refraction.

Improve this answer
$\endgroup$
2
  • $\begingroup$ I corrected the angle. That is indeed what we would expect for an infinitely thin lens, but is inconsistent with Abbe relations. $\endgroup$
    – magtweezers
    Commented Nov 4, 2020 at 12:12
  • 1
    $\begingroup$ Now I see that my answer was not very clear. What I meant is that the beam passing through the optical center should not be deviated by the lens, but it will be deviated by the oil/air interface, accordingly to Abbe relations (for $\gamma =1$). This results in an angle $\alpha_{\mathrm{air}}$ (which is the same as the incident angle in air before the ray enters the oil). $\endgroup$
    – QuantumApple
    Commented Nov 4, 2020 at 15:35

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.