Is the well known textbook-formula for net-thrust oversimplified or do I have a misinterpretation?

Question

In order to understand how Jet-Engines works I came across the "famous" formula for net-thrust:

https://en.wikipedia.org/wiki/Turbojet#Net_thrust

$F_{thrust} = Q_{out} \cdot v_{out} - Q_{in}\cdot v_{in}$

From well known physical law about momentum this sound quite reasonable for me.

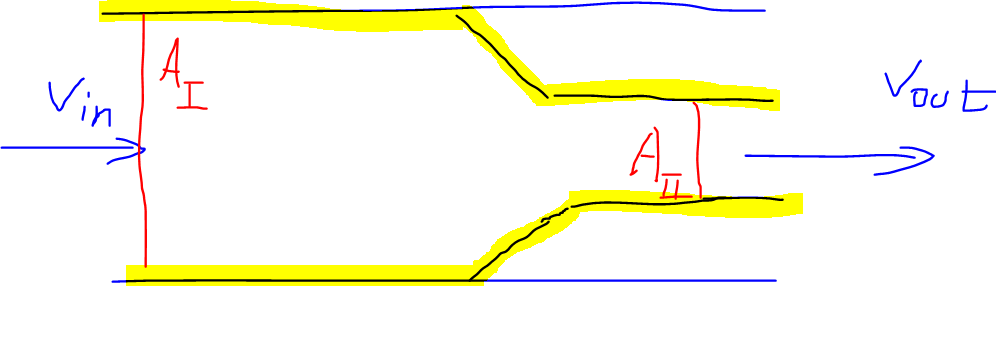
But now assume an empty cylindrical chamber with nozzle at the end, mounted statically in a wind channel:

Regardless of any details, just by continuity equation we must have $Q_{out} = Q_{in}$ and $v_{out} \gt v_{in}$

Nevertheless, this construction would not produce a net propulsion in left direction. It it were true, we just had to put this "empty" construction on a plane...

I was thinking over that quite a time and become already a little crazy. Where is my mistake? Or is the formula above not the whole truth?

Anyway, I'm not sure if

$v_{in} = v_{air}$

although it sounds reasonably for me.

But even when I take the formula

$F_{thrust} = Q_{out} \cdot v_{out} - Q_{in}\cdot v_{air}$

a left thrust would be produced, whenever

$v_{out} \ge v_{air}$

Is this the key-factor where I'm wrong? Do I have to take change of static pressures due to Bernoulli's law into account? But those are not included in the formula I linked to.

Answer 1

For the situation you sketched, the kinetic energy of the incoming air builds up pressure in the large part of the tube, and this forces an equal quantity of air out through the smaller tube with a larger velocity but with a lower pressure. The net force on the tubes is from the horizontal component of the pressure (in the forward direction)on the slanted section. This is consistent with the behavior of a “wind sock” that you might see at a small airport. For a jet engine you might run this backwards with extra energy from fuel. (Most jet engines have a more complex geometry and an internal turbine.)