5
$\begingroup$

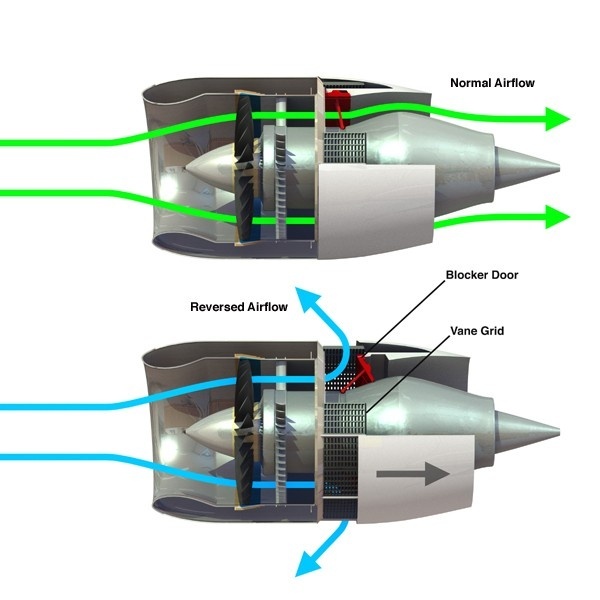
Thrust reversers are commonly used on aeroplane jet engines, to generate backwards thrust. They work by directing the hot, high speed exhaust forwards instead of backwards. At a basic level, the air has a net momentum gain forwards because the incoming air is slow (and dense), but the outgoing air is fast (and less dense), so the engine has a net momentum gain backwards. This makes sense to me (and I hope it's right).

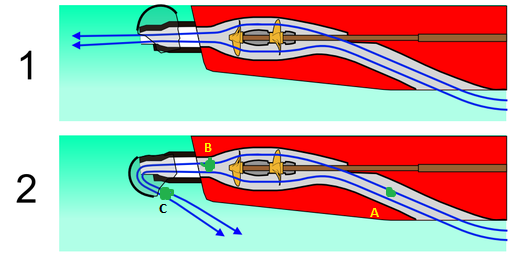
enter image description here

Boats also apparently have the same system, however I don't understand the physics. Since water is basically in-compressible, the water coming in cant be less dense than the water going out. This must mean that the water coming in is travelling at the same speed as the water going out, so there is no net momentum change. Am I being dumb? It seems like you could just connect a pipe directly from the exhaust to the intake and it would be the same. Is the main slowdown from something different, like the drag inside the intake or something?

enter image description here

edit: is momentum the wrong thing to be thinking about here? maybe its to do with the fact that at the inlet, the water is coming from all directions on the surface of the hull, but at the outlet it's all going in one direction?

Improve this question
$\endgroup$
6
  • $\begingroup$ I think the inertial is already causing the boat to decelerate, then there is the drag force from the resistance of the water and now the captain just need to apply additional force like using the reverser to decelerate further still. $\endgroup$
    – user6760
    Commented Nov 3, 2017 at 6:41
  • $\begingroup$ right ok, but what i'm asking is how, exactly, doe the reverser provide this thrust? Where is the force coming from, in terms of the momentum of the water? $\endgroup$
    – BeB00
    Commented Nov 3, 2017 at 6:53
  • $\begingroup$ under normal operation the force is in x direction, when using reverser there is force in perpendicular (y) direction as well as opposing (x) direction I'm using free body diagram hope it helps ;D $\endgroup$
    – user6760
    Commented Nov 3, 2017 at 7:04
  • $\begingroup$ That is 0 help. There is a force acting backwards on the thrust reverser, but there is also a force on the propeller blades acting forwards. Why are these forces different? $\endgroup$
    – BeB00
    Commented Nov 3, 2017 at 7:05
  • $\begingroup$ General tip: Let's not have posts look like revision histories. $\endgroup$
    – Qmechanic ♦
    Commented Nov 3, 2017 at 7:52

3 Answers 3

Reset to default
0
$\begingroup$

The change in speed of both the wind and water is due to the props in the engines, which you can see as the ‘fan blades’ in your linked diagrams. They push the fluid through the system (rather than just having it flow straight through an empty pipe), causing the outgoing fluid to have more velocity than the incoming fluid.

In the case of the thrust reverser, they simply angle the exhaust direction such that the fluid is being ejected in the opposite direction, reversing the direction in which the generated force is being directed.

How Propellers Work

Think of the propellers as rotating wings. We can apply the same principles to the propeller as we do the wing of an airplane, which tell us that there will be an area of lower pressure in the exhaust direction. We can then apply Bernoulli’s Principle to show that the velocity of the outflowing water will be larger than that of the inflowing water.

In order for this to be sustainable, the outflowing water must push out of the exhaust pipe into the surrounding area with some force. Newton’s Third Law will then tell us that this ejection will cause the vessel to receive a force acting opposite to the exhaust direction.

TL:DR

Due to the pressure change across the propeller, the velocity of the fluid will not be the same at all points in the process (it will become faster as the fluid passes through the propeller).

The size of the nozzle at the end of the process is smaller than the water intake, which allows for the change in momentum of the water exiting the system.

Improve this answer
$\endgroup$
5
  • $\begingroup$ I understand how propulsion works, I'm looking for a more mathematical answer here, in terms of momentum. Since the water is incompressible, isn't it moving at the same speed at points A, B and C in the image (assuming the pipes are all the same size)? In that case, I don't get how the net momentum change of the water isnt 0, or even to the left (making the ship move to the right)? $\endgroup$
    – BeB00
    Commented Nov 3, 2017 at 6:20
  • $\begingroup$ If we assume that the inlet pipe and outlet pipe are the same size, how can the water be flowing out faster than it's flowing in? Where is this extra water mass coming from? $\endgroup$
    – BeB00
    Commented Nov 3, 2017 at 7:06
  • $\begingroup$ A way of seeing that this isn't answering the question is to consider ramjets: they don't have fans or propellors so can't possibly work like this. Yet they do work. I think it's also clear that they would not work for water. So the propulsion mechanisms for (traditional, not turbofan) jet engines and these water things is very different. $\endgroup$
    – user107153
    Commented Nov 3, 2017 at 8:05
  • $\begingroup$ Also, regardless of what is and isn't happening at the propeller, what we really care about is the inlet/outlet $\endgroup$
    – BeB00
    Commented Nov 3, 2017 at 8:21
  • $\begingroup$ I think the key thing here (that I missed) is that, since the pipes at the outlet will be smaller, the exhaust can have a high velocity. This means that even though the same amount of mass is coming out as is going in, each bit of that mass has more momentum, because it's travelling faster (p=mv), so the overall momentum of the exhaust is higher. This is only possible due to the difference in size. $\endgroup$
    – BeB00
    Commented Nov 3, 2017 at 23:13
0
$\begingroup$

A key thing you are missing here is that you do not need any obvious density differential to create a pressure differential. Liquids are of course compressible but it takes a lot more energy to decrease their volume by a given amount. That way, a tiny amount of compression stores a lot of energy in a liquid, whereas you would need to compress a gas by a considerable amount for the same energy. Since I am not very familiar with the formal theory of fluid dynamics, you would have to wait for a professional to answer this formally. I'm sure it hides somewhere in the Navier-Stokes equations. But as a start, look up compressibility, which is a measurable property of a fluid. To put it in simple terms:

  • Pressure is not the same as density or compressibility
  • Liquids can be compressed, you just need more pressure per given volume change

Just for fun: If water would physically be incompressible, you would have a backwards momentum of the water particles that flow into the inlet and would net a backwards momentum on the boat just by redirecting the incoming flow. A way to think about it might be that the inlet "selects" for particles that have backwards momentum with respect to the boat, because it opens to the front. But if you then consider the outlet(which would basically do the same thing), the explanation breaks down again - so yes, i think you really need the pressure differential to explain it.

But what I now wonder is: Is there a way to explain the forward thrust without invoking pressure changes, just with pure Newtonian Physics?

Improve this answer
$\endgroup$
9
  • $\begingroup$ I realise liquids can be compressed, but I highly doubt that that's the dominant mechanism here. The only reason the compression matters is because it allows the outgoing air to have a lot more momentum than the incoming air. I doubt the 0.01% increase in water density is going to make a difference in the momentum of the outgoing water. $\endgroup$
    – BeB00
    Commented Nov 3, 2017 at 21:33
  • $\begingroup$ Also, that stuff about momentum seems incorrect, because I suspect that this system would still work even if the water were entirely still with respect to the boat, i.e. all the water molecules had 0 momentum. $\endgroup$
    – BeB00
    Commented Nov 3, 2017 at 21:38
  • $\begingroup$ Yes, I see your point. But as I tried to explain, the amount of compression going on says nothing about the amount of energy being transferred to the medium. The only thing that matters is how much of a pressure differential you can enforce, and that is completely independent of the volume change. $\endgroup$
    – lthz
    Commented Nov 3, 2017 at 21:38
  • $\begingroup$ I agree that energy is clearly being transferred to the water, but I'm specifically interested in the momentum, and how the boat transfers forward momentum to the water $\endgroup$
    – BeB00
    Commented Nov 3, 2017 at 21:39
  • $\begingroup$ Yes of course, the momentum circle argument is incorrect in practice, but that was my point. When you believe the fluid to be entirely incompressible, you would end up with this cycle I believe. $\endgroup$
    – lthz
    Commented Nov 3, 2017 at 21:42
0
$\begingroup$

I realize now that I was being a bit dumb. All of this stuff that we went into in chat is actually not relevant.

There are only two places where the momentum change of things is relevant: At the propeller and at the thrust reverser.

You can ignore everything about the pressure and compressibility of water and just think of it as a particle system.

If you imagine that the propeller takes a non-moving particle of mass a and throws it backwards at speed -b, the propeller adds the momentum ab=C to the boat, and a(-b)=-C to the particle.

When the particle hits the thrust reverser, its speed changes from -b to +b, so its momentum changes from -C to +C, subtracting 2C from the boat momentum, giving the boat overall a momentum of -C, decreasing it's speed.

This works regardless of pipe size changes and pressure changes etc, and regardless of whether the water is initially still or moving.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.