Inertial Torque Exerted By Engine on Crankshaft

Question

In Shigley(5th Edition), in Chapter 14. Dynamics of Reciprocating Engine ,Section 14.7 Inertia Forces, the inertia torque exerted by the engine on the crankshaft is given as $$ \mathbf{T}_{21}^{\prime \prime}=-\left(-m_{B} A_{B} \tan \phi\right) x \hat{\mathbf{k}} $$

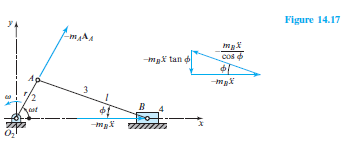
Background : In this section they have assumed equivalent masses $m_A$ and $m_B$ for the crank and connecting rod (link 3) at the crank Pin A and wrist pin B respectively. (with $m_A$ rotating mass at A ($m_{A}=m_{2} \frac{r_{G}}{r}$ + $m_{3} \frac{l_{B}}{l}$) where $r_G$ is the CG of Crank from $O_2$ and $l_B$ is the CG of connecting rod from B and $m_B$ reciprocating mass at B, ($m_{B}=m_{3} \frac{l_{A}}{l}$ + $m_{4}$) , $l_A$ is the CG of connecting rod from A) . From the formulas for inertia force and torques or equivalently Frame Force and Frame Moment(Sec 12.7 Shaking Forces and Moments) as : $$ \begin{array}{l} \mathbf{F}_{s}=\sum\left(-m_{j} \mathbf{A}_{G_{j}}\right) \\ \mathbf{M}_{s}=\sum\left[\mathbf{R}_{G_{j}} \times\left(-m_{j} \mathbf{A}_{G_{j}}\right)\right]+\sum\left(-I_{G_{j}} \alpha_{j}\right) \end{array} $$ we can calculate the inertia forces : \begin{array}{l} -m_{A} \mathbf{A}_{A}=m_{A} r \omega^{2}(\cos \omega \hat{t} \mathbf{i}+\sin \omega t \hat{\mathbf{j}}) \\ -m_{B} \mathbf{A}_{B}=m_{B} r \omega^{2}\left(\cos \omega t+\frac{r}{l} \cos 2 \omega t\right) \hat{\mathbf{i}} \end{array} As per my understanding we incorporate Inertia Forces in dynamic systems to reduce the system to static system using d'Alembert Principle.

My doubt is although we have reduced the system to its equivalent mass system and the respective inertia forces for both of them pass through $O_2$

1. How is the inertia force generating a torque about $O_2$ ? (like as mentioned in the text, it says $-m_A\mathbf{A}_A$ can be neglected because it does not have any moment arm about $O_2$ , so why isn't $-m_B\mathbf{\ddot{X}}$ also ignored along the same line of reasoning?
2. The inertia force due to $m_B$ is resolved into components and only one of the component ($-m_B\mathbf{\ddot{X}}\:tan \phi$ ) is used to calculate the torque. Won't the net torque if we use both the component be zero, as the force $-m_B\mathbf{\ddot{X}}$ has zero moment arm in the first place and hence the net torque of its components about $O_2$ will also be zero.

Answer 1

Upon reading further I came to the conclusion. The inertial force acting $-m_B\mathbf{\ddot{X}}$ on B can 'transmit' the force(acting along the connecting rod $F_{43}$ ) through the connecting rod, and the rod being mass-less will transfer the forces.We have $F_{34}=\frac{-m_B\mathbf{\ddot{X}}}{cos\phi}$ using force balance at point $B$ . Torque at $O_2$ will be $r_\perp*F_{43}$ and $r_\perp=x*sin\phi$. Using these two equations we get $\mathbf{T}_{21}^{\prime \prime}=-\left(-m_{B} A_{B} \tan \phi\right) x \hat{\mathbf{k}}$