Confusion regarding angular momentum of a tilted rod

Question

I have a question regarding angular momentum and torque in the following example. This is not a homework problem, I just believe understanding some parts of this particular problem would help me understand the two concepts better.

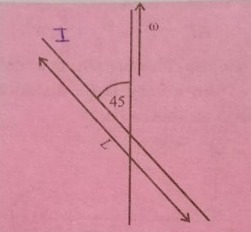
I have a rod of length $l$ oriented at an angle $\phi$ about an axis that passes through its center of mass. The rod is rotating about this axis, and I'm supposed to find the magnitude of the angular momentum and the torque.

In this example, the given angle is $\pi/4$, but I'm looking for a general solution.

Using the inertia tensor, I can easily find the moment of inertia of this rod, in the direction of the axis. let us have the $x$ axis along the rod, and the $y$ axis perpendicular to it. Let us have the axis of rotation along some direction $\vec{a}$. We can see, that $\vec{a}=a\cos\phi\hat{i}+a\sin\phi\hat{j}$.

If $I$ denotes the inertia tensor, and $I_a$ represents the moment of inertia about this axis $\vec{a}$, then I can easily write :

$$I_a=( cos\phi, \sin\phi) I ( cos\phi, \sin\phi)^T $$

In the case of our rod, the moment of inertia along the length ($x$ axis) is $0$. So, we can just say :

$I_a=I_y \sin^2\phi=\frac{1}{12}Ml^2\sin^2\phi$

So, I think I've managed to find the moment of inertia about the $a$ axis.

Now I'm confused regarding how should I calculate the angular momentum in this case.

On one hand, I have $$\vec{L}=I\vec{\omega}$$

So, I can simply multiply $\omega\hat{a}$ to the moment of inertia along $\hat{a}$.

Using this, I get $$\vec{L}=\frac{1}{12}Ml^2\omega \sin^2\phi\hat{a}$$

However, I could also have done the following :

$$L=I_x\omega_x \hat{i}+I_y\omega_y\hat{j}$$ Here $I_x=0$ and $\omega_y=\omega\hat{a}.\hat{j}=\omega\sin\phi$

In this case, I get: $$\vec{L}=I_y\omega_y=\frac{1}{12}Ml^2\omega\sin\phi\hat{j}$$

I'm inclined to believe that the second one is correct, but I have no idea why the first method seems to be wrong here. Any explanation would be highly appreciated.

Answer 1

Your projection of the inertial moment into the rotational axis, $\hat a$, is not correct.

Starting with your inertial moment in your $\hat i$ (along the rod) and $\hat j$ (perpendicular to the rod) coordinate. $$ \bf{I} = \begin{bmatrix} 0 & 0 \\ 0 & \frac{1}{12}Ml^2 \end{bmatrix} $$

The transform to the $\hat a$ and $\hat b$ (perpendicular to $\hat a$) by a rotation matrix $\bf R$: \begin{align} \bf I_a & = \bf R^T \bf I \bf R \\ &= \begin{bmatrix} \cos \phi & \sin\phi \\ -\sin\phi & \cos\phi \end{bmatrix} \begin{bmatrix} 0 & 0 \\ 0 & \frac{1}{12}Ml^2 \end{bmatrix} \begin{bmatrix} \cos \phi & -\sin\phi \\ \sin\phi & \cos\phi \end{bmatrix} \\ &= \frac{1}{12}M l^2 \begin{bmatrix} \sin^2 \phi & \sin\phi\cos\phi \\ \sin\phi\cos\phi & \cos^2\phi \end{bmatrix} \end{align}

Then angular momentum in $\hat a$ and $\hat b$ coordinate become \begin{align} \vec L_a & = \bf I_a \, \vec \omega_a \\ &= \frac{1}{12}M l^2 \begin{bmatrix} \sin^2 \phi & \sin\phi\cos\phi \\ \sin\phi\cos\phi & \cos^2\phi \end{bmatrix} \begin{bmatrix} \omega \\ 0 \end{bmatrix}\\ &=\frac{1}{12}M l^2 \, \omega \sin\phi \left\{ \sin\phi \,\hat a + \cos\phi \,\hat b \right\} \end{align}

You may also check that $\vec L_a = {\bf R^T} \vec L $.