EMF induced due to moving rod in magnetic field

Question

When a conducting rod moves in a uniform magnetic field as shown.

By Lorentz force it is easy to explain that EMF induced is BvL and upper end is positive and lower end is negative.

But in books, this concept is explained by Faraday's law of electromagnetic induction as the area swapped by conductor is changing and EMF is induced. But why we take area swapped into account?

I think that magnetic flux through conductor remains constant as B is constant. I am not able to justify this concept using Faraday's Law (by area swapped). Why area swapped method is used? Please help.

Answer 1

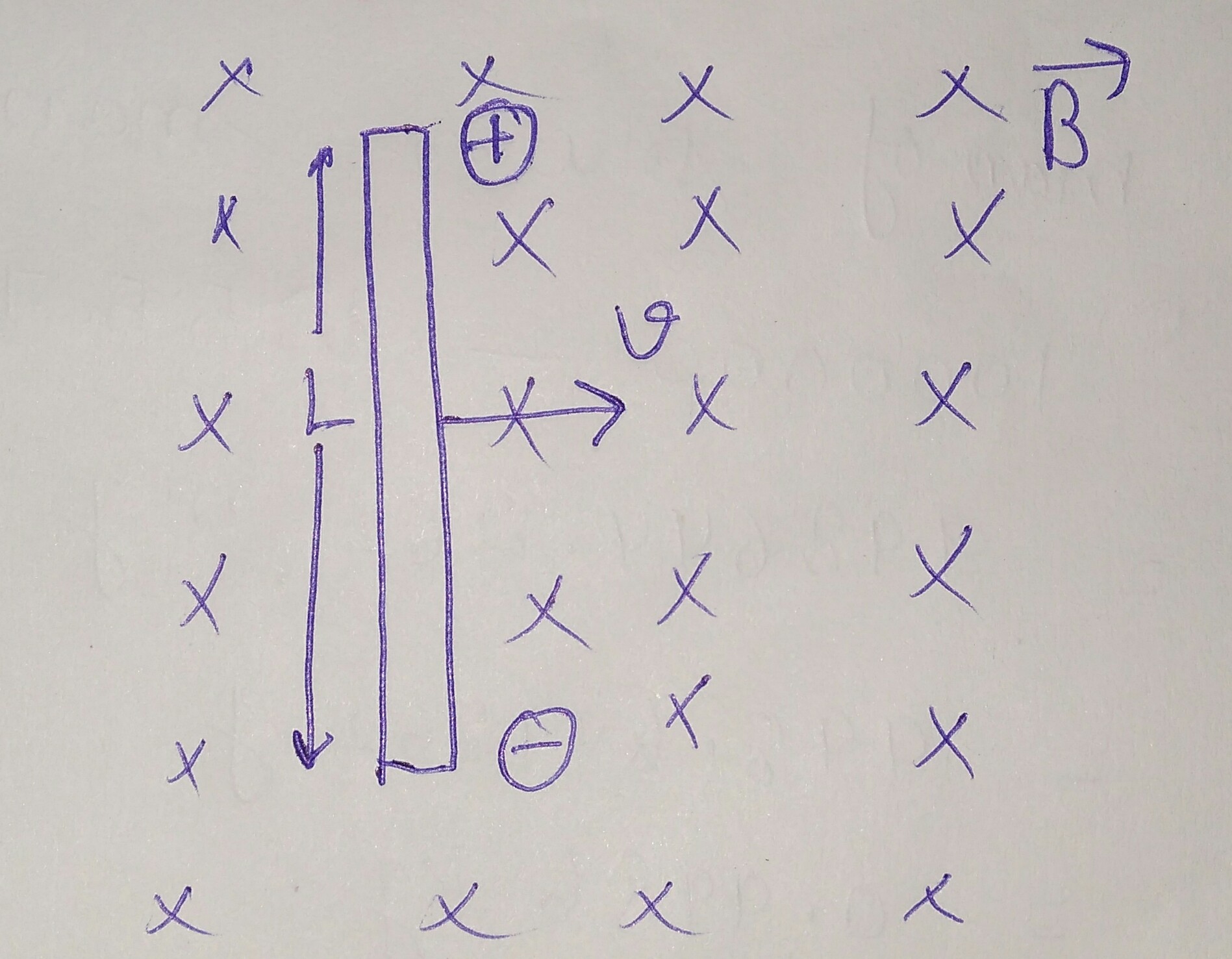
I am proving that the area-sweeping technique gives the same result as the Lorentz force method. Using a battery across the two rods in parallel doesn't change the idea as we shall see.

Magnetic Flux $\phi=\int_A \mathbf{B}.d\mathbf{A}$

Faraday's law of electromagnetic induction transforms as follows: \begin{align*} \text{EMF }\varepsilon&=-\frac{d\phi}{dt}\\ \varepsilon&=-\frac{d}{dt}\left(\int_A \mathbf{B}.d\mathbf{A}\right)\\ \varepsilon&=-\mathbf{B}.\frac{d}{dt}\left(\int_A d\mathbf{A}\right)&(\because \mathbf{B}\text{ is uniform})\\ \varepsilon&=-\mathbf{B}.\frac{d\mathbf{A}}{dt}&(\because \mathbf{A}\text{ is unidirectional})\tag{1}\\ \varepsilon&=-\mathbf{B}.\frac{d(\mathbf{l}\times\mathbf{L})}{dt}\\ \varepsilon&=-\mathbf{B}.\left(\frac{d\mathbf{l}}{dt}\times\mathbf{L}\right)&(\because \mathbf{L}\text{ is constant})\\ \varepsilon&=-\mathbf{B}.\left(\mathbf{v}\times\mathbf{L}\right)&(\because \mathbf{v}dt=d\mathbf{l})\\ \varepsilon&=-\mathbf{L}.\left(\mathbf{B}\times\mathbf{v}\right)&(\because \mathbf{B}.(\mathbf{C}\times\mathbf{A})=\mathbf{A}.(\mathbf{B}\times\mathbf{C}))\\ \varepsilon&=\left(\mathbf{v}\times\mathbf{B}\right).\mathbf{L}&(\because \mathbf{A}\times\mathbf{B}=-\mathbf{B}\times\mathbf{A})\tag{2} \end{align*} In the figure, $\mathbf{F}_{\text{Lorentz}}=q(\mathbf{E}+\mathbf{v}\times\mathbf{B})=q(\mathbf{v}\times\mathbf{B})$

Work along the moving rod $=q(\mathbf{v}\times\mathbf{B}).\mathbf{L}\Rightarrow \varepsilon = (\mathbf{v}\times\mathbf{B}).\mathbf{L}\tag{3}$

So, the area-sweeping technique $(1)$ produces $(2)$ for the shown configuration. 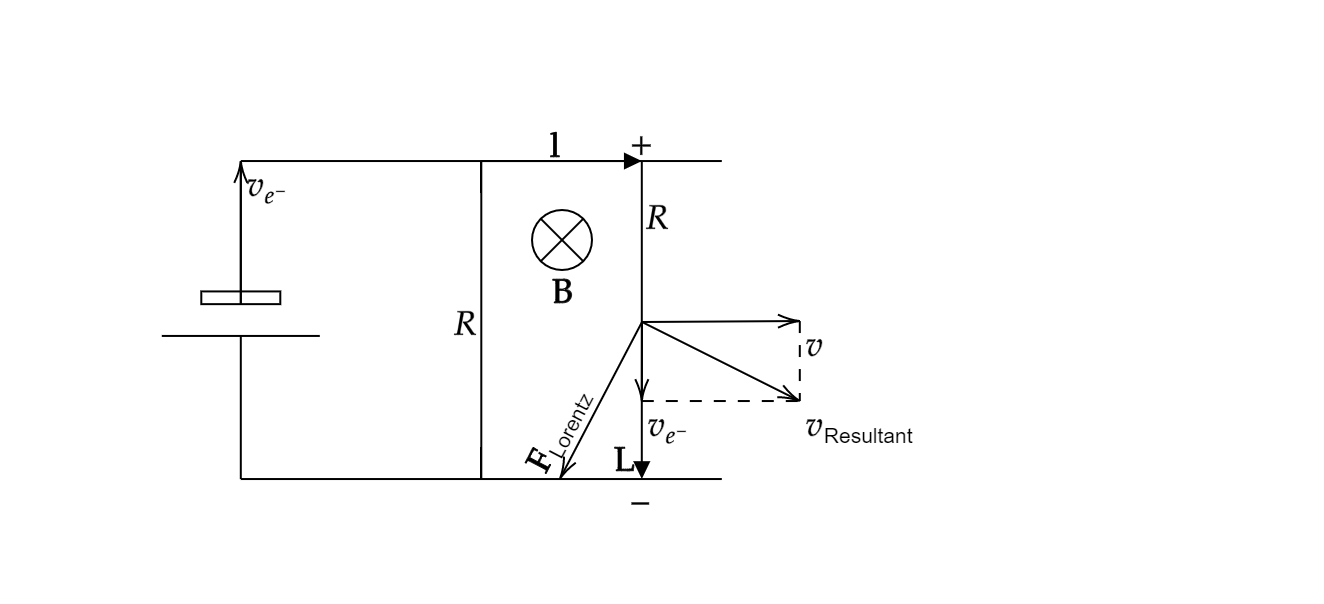The trick also works for a single rod without a circuit even though there is no real area changing that changes the flux in turn inducing an EMF. $\mathbf{F}_{\text{Lorentz}}$ is along the rod in this latter case. Regardless, the EMF produced is the same due to taking the dot product of $\mathbf{F}_{\text{Lorentz}}\equiv q(\mathbf{v}\times\mathbf{B})$ with $\mathbf{L}$ in $(3)$. The difference is only that there is force required to move the rod towards the right in the former case because $v_{e^-}$ gives a component of Lorentz force on the rod towards the left.

Answer 2

"I think that magnetic flux through conductor remains constant as B is constant."

It's not the flux "through the conductor" that matters. It's the flux through the area swept out by the conductor. Imagine that the straight conductor (length $\ell$) is lying on a table, and that there is a uniform magnetic field acting downwards. (Actually there is : the vertical component of the Earth's field.) You then move the conductor across the table at speed v in a direction at right angles to itself. In time $\Delta t$ it sweeps out an area $\ell v \Delta t$

The flux through the swept out area is $$\Delta \Phi = (\ell v \Delta t)B$$

So according to Faraday's law, the induced emf is $$\mathscr E=\frac {\Delta \Phi}{\Delta t}=\frac {(\ell v \Delta t)B}{\Delta t}=B\ell v$$ So we have recovered the result that you obtained from the magnetic Lorentz force. In my opinion the magnetic Lorentz force is more fundamental than Faraday's law when the emf is due to movement of conductors. However Faraday's law has the merit of spanning two types of electromagnetic induction: this one and the type due to changing flux through a stationary circuit, which depends on the electric field part of the Lorentz force.