1
$\begingroup$

Suppose i have a region with uniform magnetic field which is changing with time. For finding EMF induced in a conductor, we use $\frac{d\phi}{dt}$, where $\phi=B.A$ (as $B$ is uniform). $$\implies EMF = A\frac{dB}{dt}$$ after reading some books and searching on internet, i concluded that if we have to find EMF through a closed circuit, then A is the area enclosed by closed circuit, as we need to find magnetic flux through it.

But what if we need to find EMF induced through an isolated conductor such as a rod.

NOTE: The rod is at rest initial conditions

So far what i have got-

if we try to find out the reason for EMF induced, there is an induced circular(non-conservative) electric field in region of changing magnetic field. So it accelerates electrons in conductor. Magnetic force acts on these moving charges (electrons) which induces the EMF. But how to relate it with magnetic flux?

Improve this question
$\endgroup$
5
  • 2
    $\begingroup$ You could use Faraday's law in its local form. Find the induced electric field Eind in the geometric area occupied by the rod. If the conducting rod is static, the net electric field Etot inside it must be zero, so surface charge will develop in such a way as to compensate the induced field inside. The EMF you are looking for is the path integral of Eind from one end of the rod to the other. And it has been killed by the Colombian field of the charge. But an answer will depend on your ability to manage vector calculus. $\endgroup$
    – Peltio
    Commented Oct 6, 2022 at 0:45
  • $\begingroup$ @Peltio "You could use Faraday's law in its local form. Find the induced electric field $\mathbf E_\text{ind}$ in the geometric area occupied by the rod." I'd like to know how you'd do this using $\mathbf {\nabla} \times \mathbf E=-\frac{d\mathbf B}{dt}$ ! $\endgroup$
    – Philip Wood
    Commented Oct 6, 2022 at 8:02
  • $\begingroup$ @PhilipWood well, it's a PDE that tells you that the electric field curls around the decrement in the magnetic field. Of course we need boundary conditions and iirc at least another Maxwell's equation. For linearly increasing B the solution is a symmetric tangentially directed E whose magnitude increases like r inside the disk and decreases like 1/r outside of it. Once we have this field, we can see what it will do to the conducting bar: it will displace charges on its surface in order to make the resultant field zero. When inside the disk, the path integral of E is zero in the bar and !=0 out $\endgroup$
    – Peltio
    Commented Oct 6, 2022 at 23:14
  • $\begingroup$ To the OP, I don't have time now to write an answer, but to answer your edit, when you talk about flux you need to specify an area, so that's the area you will apply Faraday's law in integral form. --- to finish my comment above: when the bar is outside the disk, the path integral of E will be zero both inside and outside the bar (as long as the path outside does not go around the disk) $\endgroup$
    – Peltio
    Commented Oct 6, 2022 at 23:19
  • $\begingroup$ @Peltio Agree with your analysis. Maybe the situation isn't as intractable as I'd thought. With increasing $B$ the top end of the rod should acquire a positive charge. $\endgroup$
    – Philip Wood
    Commented Oct 7, 2022 at 7:57

1 Answer 1

Reset to default
0
$\begingroup$

As soon as we connect a voltmeter to measure the voltage we form a loop. The voltage that we read will be for the loop as a whole and the voltmeter will read positively or negatively according to whether it is placed to the left or right of the rod.

But, you might ask, suppose that we connect nothing to the rod: will one end of the rod acquire positive charge and the other negative (as do the terminals of a battery)? Yes indeed. We can calculate the resulting potential difference in this way...

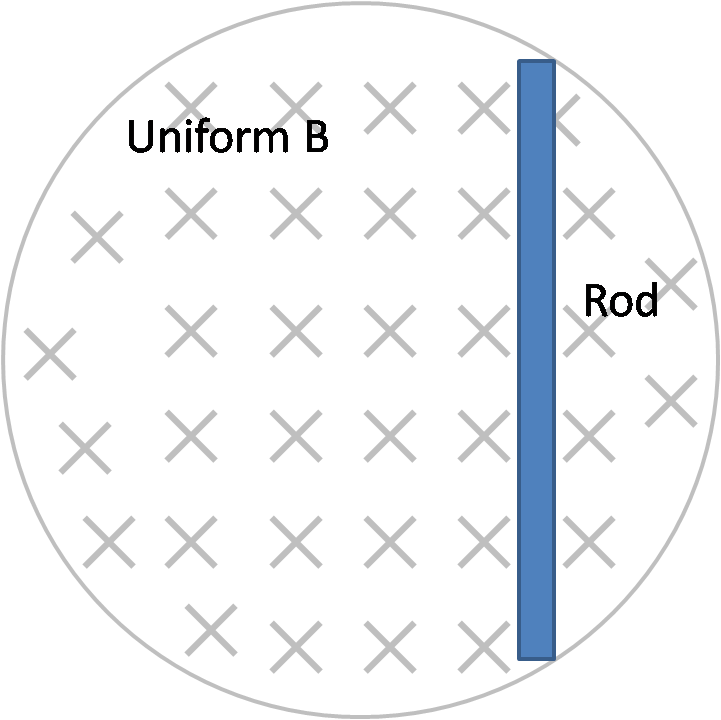
Call the centre of the circular patch (in which there is a magnetic field changing at a rate $\dot B$), 'O'. Call the ends of the rod 'P' and 'Q', and its midpoint, 'M'. Write PQ$=l$, OM$=h$. Then the emf induced around triangle OPQ will be: $$\mathscr E = \text{area of triangle OPQ}\times \dot B= \tfrac 12 lh\dot B.$$ However, there is no emf in either OP or QO, the radial sides of the triangle. Such emfs and their associated electric fields would, by symmetry, have to be radially outwards for all radii or radially inwards for all radii, and that is ruled out by Gauss's law, as there is no 'central' charge.

So the emf we have calculated will be induced along PQ. The emf will cause charges to move in the rod until the pd due to the charges is equal in magnitude to the emf (so that there is no net electric field in the rod). So... $$\text{pd between ends of rod} =\tfrac 12 lh\dot B.$$

Note: treating the rod as part of the triangle OPQ was done just for convenience of calculation. It is not essential to choose the closed loop OPQ, nor indeed any closed loop. A more physically direct method is to use the result (obtainable from $\vec \nabla \times \vec E=-\frac{d\vec B}{dt}$, Stokes's theorem and symmetry) that at distance $r$ from O the electric field is tangential to a circle centred on O and of magnitude $E=\tfrac 12 r\dot B$. It is easy to show that the component of this field parallel to PQ is $E_\text{PQ}=\tfrac 12 h\dot B$ all along PQ, giving the same value for the emf induced in PQ as calculated earlier!

Improve this answer
$\endgroup$
3
  • $\begingroup$ Thanks to the comments of @Peltio, I have completely revised this answer. Any mistakes or infelicities in the answer are entirely my responsibility. $\endgroup$
    – Philip Wood
    Commented Oct 19, 2022 at 14:27
  • $\begingroup$ Given that the E-field and the net force per unit charge are both zero within the conductor, is it even meaningful to say that there is a non-zero EMF? Is such a non-zero EMF useful in any way, given that if you were to try to connect the rod to a circuit, the EMF would be completely different and depend on the loop that is formed? $\endgroup$
    – Puk
    Commented Oct 23, 2022 at 0:13
  • $\begingroup$ @Puk Your first sentence: I think that saying there is a non-zero emf IS meaningful, because (a) the emf is calculable (different methods giving the same answer) and (b) the ends of the rod acquire opposite charges as a result. Your second sentence: I agree with you that calculating the emf might be considered not to be useful; my first paragraph made the point about the loop-dependency and should have served as a health-warning. $\endgroup$
    – Philip Wood
    Commented Oct 23, 2022 at 8:17

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.