0
$\begingroup$

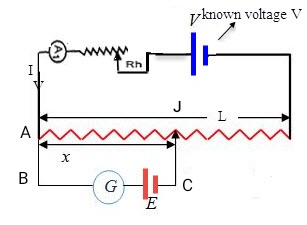
In the following circuit involving a potentiometer;

enter image description here

Assume $V$ to be the voltage produced by the cell in the primary circuit across the length $AJ$ of the potentiometer wire, and $E$ to be that produced by the cell of the secondary circuit.

In the case of $V=E$, it's known that there is zero current through the secondary circuit. The current would obviously follow the path of the potential drop and not the rise, but in this limiting case, why is there current flow through the potentiometer wire instead of the secondary circuit, when the potential drops are similar in both?

Improve this question
$\endgroup$
6
  • 1
    $\begingroup$ How did you conclude that "the net potential difference between A and J is zero"? $\endgroup$
    – SarGe
    Commented Jun 11, 2020 at 16:29
  • $\begingroup$ Isn't the potential calculated by the scalar sum? $\endgroup$
    – harry
    Commented Jun 11, 2020 at 16:30
  • 1
    $\begingroup$ As you mentioned in the question, potential difference across AJ is V=E and not 0. $\endgroup$
    – SarGe
    Commented Jun 11, 2020 at 16:34
  • $\begingroup$ Oh, right. So current flows through the path that has the potential drop, and not the rise, right? $\endgroup$
    – harry
    Commented Jun 11, 2020 at 16:38
  • 1
    $\begingroup$ Yes, you got it. $\endgroup$
    – SarGe
    Commented Jun 11, 2020 at 16:39

1 Answer 1

Reset to default
1
$\begingroup$

enter image description here

As potential difference between points $A$ and $B$as well as $J$ and $C$ is zero, there is no current in the secondary circuit. However, there is current in the primary circuit due to the primary (known) cell.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.