Net acceleration in rotational motion is $ \sqrt{a_t^2+a_c^2}$ ($a_t$ and $a_c$ are tangential and centripetal acceleration respectively). Why isn't angular acceleration also considered?
1
-
$\begingroup$ Angular acceleration around which axis? $\endgroup$– GiorgioP-DoomsdayClockIsAt-90Commented Dec 8, 2019 at 5:26
Add a comment
|
3 Answers
$\begingroup$
$\endgroup$
3
That's because angular acceleration is not the same as linear acceleration, angular acceleration is the rate of change of angular velocity, and acceleration is the ate of hange of linear velocity. The tangential acceleration is however related to the angular acceleration as $$a_t=r\alpha$$
-
$\begingroup$ But isnt angular acceleration also a component of the net acceleration acting on a rotating body? $\endgroup$ Commented Dec 8, 2019 at 4:57
-
$\begingroup$ They don’t have the same dimensions, so one can’t be a component of the other. $\endgroup$– G. SmithCommented Dec 8, 2019 at 5:13
-
$\begingroup$ @ShreyaRajaram, angular acceleration is not a type of acceleration that you think it is. It is quite different, think of it as an analogue of acceleration, like angle is an analogue to distance. $\endgroup$– SK DashCommented Dec 8, 2019 at 5:17
$\begingroup$
$\endgroup$
Angular acceleration and acceleration are different physical quantities. Angular acceleration is just another way to express tangential component of acceleration. Angular acceleration is included as tangential component in net acceleration.
$\begingroup$
$\endgroup$
When you have some acceleration vector $\mathbf a$ you can always write it in the form of component vectors in perpendicular directions. Now in 2 dimensional motion there are two components (say) $ \hat {\mathbf i}$ and $ \hat {\mathbf j}$ . In non uniform circular motion the acceleration vector can be broken into (out of many possible combinations) two components one tangential and the other centripetal.
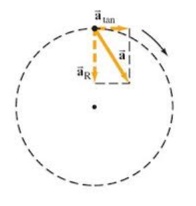
So when finding the magnitude of the original vector there is no argument as to why tangential and centripetal ones (they are defacto what they are). Also angular acceleration doesn't have the same dimensions as the linear ones.