When you have some acceleration vector $\mathbf a$ you can always write it in the form of component vectors in perpendicular directions. Now in 2 dimensional motion there are two components (say) $ \hat i$$ \hat {\mathbf i}$ and $ \hat j$$ \hat {\mathbf j}$ . In non uniform circular motion the acceleration vector can be broken into (out of many possible combinations) two components one tangential and the other centripetal.
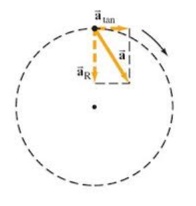
So when finding the magnitude of the original vector there is no argument as to why tangential and centripetal ones (they are defacto what they are). Also angular acceleration doesn't have the same dimensions as the linear ones.
