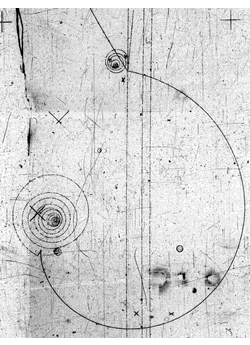
The bubble-track phenomenon is not in conflict with the projection postulate, as long as we use the projection postulate appropriately. Applying the projection postulate directly to the particle's position observable $\hat X$ (the one defined by $\hat X\psi(x)=x\psi(x)$) is not appropriate. Real measurements have finite resolution, and applying the projection postulate directly to $\hat X$ amounts to assuming that the measurement has infinite resolution.
To naturally account for the finite resolution of the real measurement, we can use a model in which the molecules comprising the bubble chamber (and atmosphere, etc) are included as part of the quantum system, along with their interaction with the quantum electromagnetic field. In this model, the formation of bubbles, the reflection of light by the bubbles, the dissipation of heat, and so on, are all described as quantum phenomena at the microscopic level. Doing the calculations explicitly would be too difficult, but based on experience with less-daunting models, we know what will happen: the particle's position will become practically irreversibly entangled with the rest of the system, including with the light that reflected from the bubbles. Then, instead of applying the projection postulate to an observable ${\hat X}$ associated directly with the particle's position, we can apply it to an observable ${\hat M}$ associated with the reflected light, such as an observable corresponding to a two-dimensional array of photon-counters, which has a discrete set of eigenspaces.
Let $|\psi\rangle$ denote the state after a bubble has formed and scattered some light, but before applying the projection postulate. We can write this state as a sum of eigenstates $|\psi_m\rangle$ of the observable ${\hat M}$:
$$
|\psi\rangle=\sum_m|\psi_m\rangle,
$$
When applied to the observable ${\hat M}$, the projection postulate says that after the formation of a bubble and the reflection of light, we might as well replace the state of the whole system (the particle, the bubbles, the light, the air) with one of the eigenstates $|\psi_m\rangle$. As usual, the relative frequencies of these various possible outcomes are given by Born's rule
$$
\frac{\langle\psi_m|\psi_m\rangle}{\langle\psi|\psi\rangle}.
$$
Thanks to the entanglement that developed between the light and the particle's position in the original state $|\psi\rangle$, each of the eigenstates $|\psi_m\rangle$ is a state in which the particle's position is concentrated in a small region determined by the resolution of the bubble-chamber system, as described in Ryan Thorngren's answer. The important point is that the particle's position is concentrated only in a small region, not at a point. This finite resolution comes naturally when we extend the model to include the physical processes involved in the measurement.
To see how this finite resolution can fix the problem described in the OP, suppose that the bubble-chamber system resolves the particle's position to $\sim 1$ micrometer. This means that in each of the eigenstates $|\psi_m\rangle$, the particle's position is concentrated in $\sim 1$-micrometer-wide neighborhood of some point $\mathbf{x}_0$, with momentum concentrated in a neighborhood of $\mathbf{p}_0$. Let $\Delta x$ and $\Delta p$ denote the widths of these neighborhoods. We must have $\Delta x\,\Delta p\gtrsim\hbar$, but if $\Delta x\sim 1$ micrometer, then $\Delta p$ can still be as small as
$$
\Delta p\sim \frac{\hbar}{\Delta x}
\sim 10^{-28}\frac{\text{ kg}\cdot\text{m}}{\text{s}}.
$$
That's small enough to allow for the formation of a long bubble-track.
The key is that real measurements have finite resolution, and we can account for this naturally by applying the projection postulate to an observable that is farther "downstream" in the cascade of effects caused by the passage of a particle through the bubble chamber, such as an observable associated with the light reflected from the bubbles.
By the way, this is how so-called "weak measurements" can be treated in quantum theory using only the usual projection postulate.