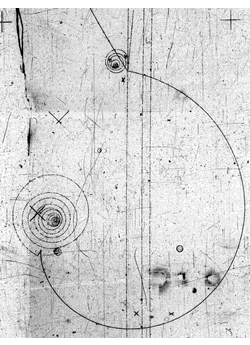
In the basic quantum mechanics lectures, we learn that we can measure any observable. That means mathematically, all Hermitian operators correspond to a physically measurable quantity. In strong contrast, it seems that experimentally, we only ever have access to the position of a quantum system. To measure its other properties, one has to find a way to entangle the other property with the position and then measure the position. A few examples:
The spin of an electron
To measure the spin of an electron, we send it into a Stern-Gerlach apparatus and let the inhomogeneous magnetic field change its trajectory depending on its spin projection. Then we detect its position on a CCD chip. $$\left( \alpha \left| \uparrow \right\rangle + \beta \left| \downarrow \right\rangle \right) \otimes \left| x_{in} \right\rangle \to \alpha \left| \uparrow, x_{out, \uparrow} \right\rangle + \beta \left| \downarrow, x_{out, \downarrow} \right\rangle$$
The energy of a photon
To measure the energy/momentum/wavelength of a photon you send it into a grating or prisma and see where it comes out. Here, the energy gets entangled with the position, like $$\left( \int s(\nu) \left| \nu \right\rangle d\nu\right) \otimes \left| x_{in} \right\rangle \to \int s(\nu) \left| \nu, x_{out, \nu} \right\rangle d\nu.$$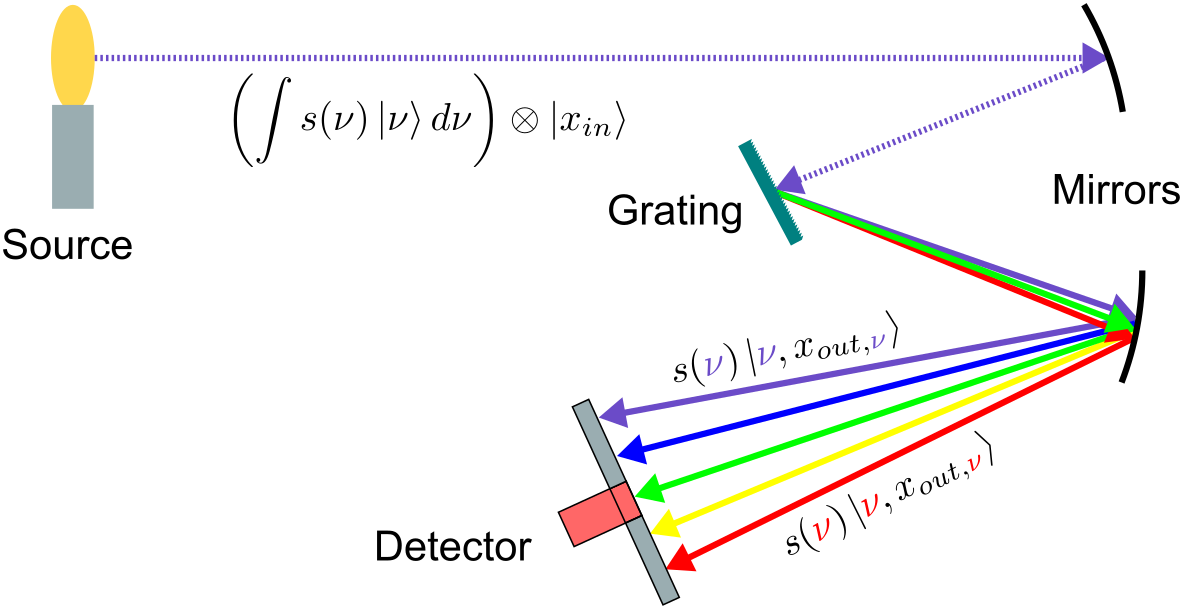
The momentum of a charged particle
Similar to a photon spectrometer, we can spatially separate different momenta through the Lorentz force. A particle with charge $q$ and a momentum $p$ is deflected with a radius $R = \frac{p}{qB}$ when it enters a homogeneous magnetic field $B$. Again, we create entanglement between the momentum and the position of the particle, then measure its position. $$\left( \int s(p) \left| p \right\rangle dp\right) \otimes \left| x_{in} \right\rangle \to \int s(p) \left| p, x_{out, p} \right\rangle dp$$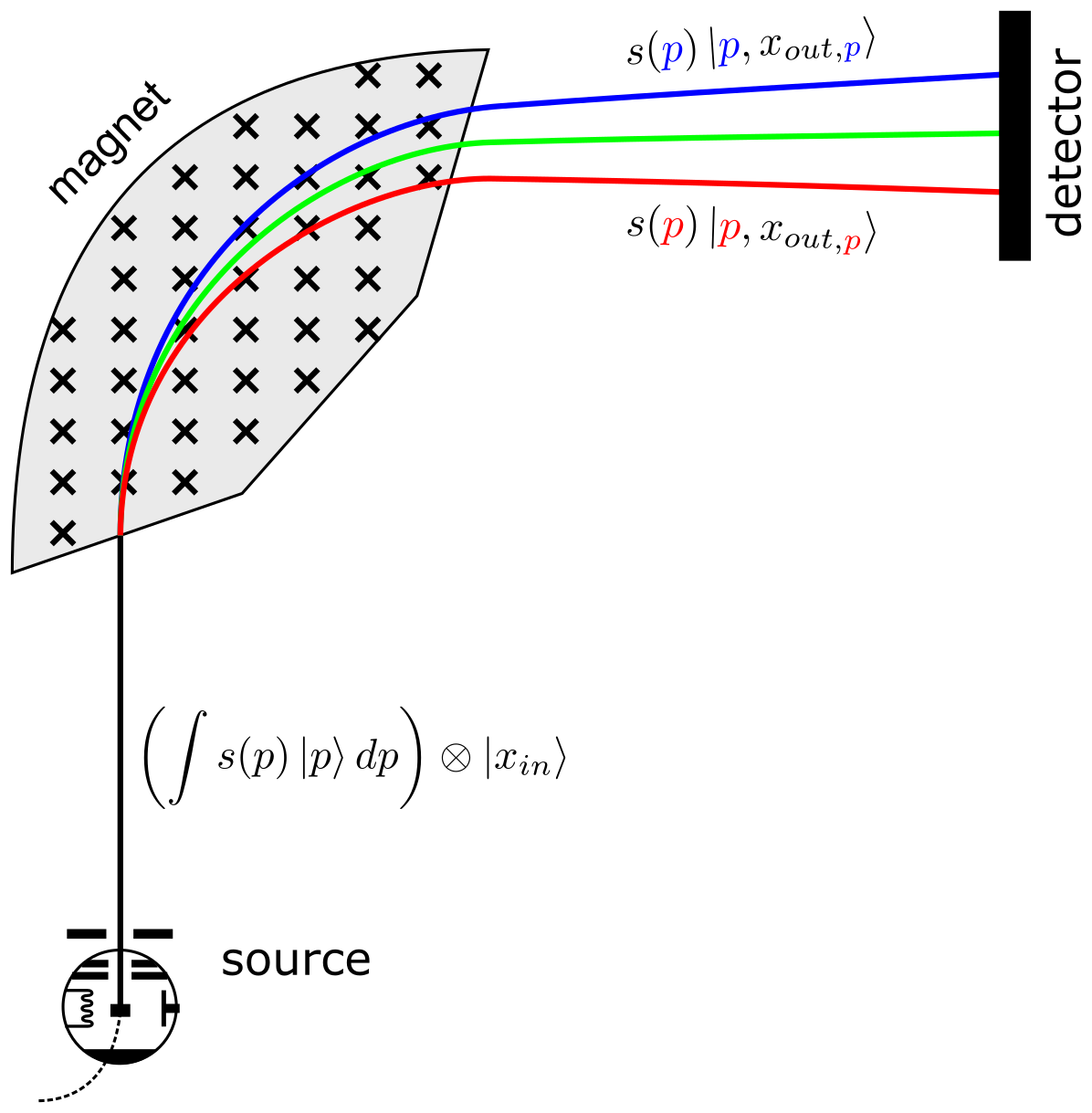
If we think about what a measurement means, it becomes clear: A measurement is an interaction of the particle with a larger system of many degrees of freedom. For the interaction to happen, the particle must spatially be close to the detector. Or in other words – interaction takes place in position space.
This all seems like it doesn't make any difference for how we use quantum mechanics, because after all we can always infer the desired property from the position measurement. But it does make a big difference when it comes to formulating a physical theory based on observations. If one tries to come up with a novel theory, which should be consistent with all measurements ever done, it only has to explain how we end up measuring particles at particular places. All the other stuff, like energy, spin, momentum, etc. are just useful concepts, but not necessarily part of the physical reality.
Is this reduction to position measurements in conflict with the statement in the introduction that any Hermitian operator corresponds to a physical measurement?
Does this concept have a name? I didn't know what to search for. The closest thing I found is Plato's allegory of the cave.
Is there a way to discriminate between a quantum mechanics formulation which allows only position measurements and one which also allows to directly measure other observables? As pointed out in Mark Mitchison's answer on Is every quantum measurement reducible to measurements of position and time?, the validity of Bohm's pilot wave approach to quantum mechanics relies on the assumption that indeed position measurements are sufficient to completely describe quantum mechanics. This could help identifying differences – if Bohm's theory makes different predictions, it could be because it doesn't allow all theoretically possible measurements.