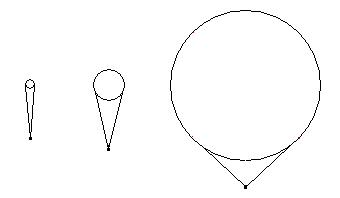
1) Why can't a balloon float into space?
A balloon rises because it is filled with a gas that is less dense than the air surrounding the balloon. Roughly speaking, the atmosphere gets less dense the higher up you go, so the highest altitude your balloon can reach is simply the altitude where the density is the same as whatever you filled your balloon with... sort of.
The above is true if most of the mass of the balloon is in the gas filling it (rather than the balloon envelope, or anything you might want to carry with the balloon). But if what matters is the density of the balloon-filling, then the ideal balloon would be filled with nothing (vacuum). This balloon could, naively speaking, take us up to an altitude where vacuum exists, or in other words, to exactly the edge of space. The trouble is, the vacuum needs to be wrapped in the envelope of the balloon. To contain a vacuum without collapsing, the envelope has to be pretty sturdy and, with known materials, this means it has to be pretty massive. This extra mass means the balloon's maximum altitude is lower - wherever the buoyant force provided by the balloon is balanced by the gravitational force of the Earth pulling down on the envelope. So an ideal balloon with a rigid massless envelope and filled with vacuum can carry us to space, but practical considerations and real materials means that the limit for balloon flight is inside the atmosphere.
2) What is this 26,000mph measured relative to?
The speed is measured along a circle drawn around the Earth. To make this easier to visualize, let's pretend that the Earth doesn't rotate, that it is a perfectly smooth sphere and that it has no atmosphere. Suppose we drop a ball. It falls, because gravity pulls it toward the center of the Earth. If instead we throw the ball, it still falls toward the center of the Earth, but its sideways velocity carries it some distance away from us before it hits the ground. The faster we throw the ball, the further away it lands. If we threw the ball fast enough, we could miss the Earth entirely - the ball would fall around the Earth. It would always accelerate toward the center of the Earth because of gravity, and for the right speed this gives a circular orbit around the Earth (faster leads to the ball flying away from the Earth, slower and it falls to the ground). The exact speed required depends on the distance from the center of the Earth - low Earth orbit, for instance, needs a velocity somewhere in the neighborhood of 15,000mph. The shape of the orbit doesn't necessarily need to be circular; it could also be elliptical.
3) Why doesn't a balloon need to move so fast to rise?
Hopefully it's clear by now that the balloon and the orbiting ball are "held up" by entirely different mechanisms. The balloon uses buoyancy, while the ball is in some sense supported by its own motion.
4) Can we get high enough, "stop" and watch the Earth leave then come back in a year?
The short answer is no. First, can we get high enough to be able to stop (i.e. have 0 velocity relative to the Earth) and never fall back to Earth? If the Earth were the only thing in the Universe, then this would be impossible because no matter how far you go, you can't escape gravity, its range is infinite. It might take a while, but gravity would eventually pull you back. The only way to get around this inevitable consequence is if there is something else out there pulling on you harder. For instance, in the Solar System, there is a place between the Sun and the Earth where the gravitational attraction from each is equally strong. Get a bit closer to the Sun from here, and you'll never fall back to Earth - you'll fall into the Sun instead. The same idea applies in the other direction - depending where you go, you might end up headed for another planet, another star or another galaxy entirely. The details of what exactly happens can be really complicated because the Sun, Earth, other planets and stars are all moving relative to each other and interacting via gravity as well. Could we stop and watch the Earth come back in a year? I think this question makes more sense if we try and stop relative to the Sun (to stop relative to the Earth would mean putting ourselves on the same orbit as the Earth around the Sun, so we would just follow our planet around). If we did this, we could indeed watch the Earth go around the Sun for a year. But it wouldn't be easy, because we'd have to provide a constant thrust away from the Sun to avoid falling into it. The only other answer to this question I can think of would be to leave the Earth, traveling in the opposite direction along its orbit at the same speed, and meet up with it in 6 months on the other side of the Sun. This would require relatively little effort because once you get into a (stable) orbit, you don't need to provide any extra effort to stay on it.
A comment on one of the other answers brought up equilibrium points between planets/other bodies. It's true that there is a point between, for instance, the Sun and the Earth where the attraction from each cancels the other. The trouble is that if you move a little bit away from this point, you start to feel a pull and accelerate away from the equilibrium (it's an "unstable point"). And moving a little away from this point is inevitable; sitting between the Earth and the Sun for instance, Jupiter still exerts a significant force and will nudge an object off the equilibrium point fairly quickly. Still, putting an object at an equilibrium point and keeping it there is a LOT less work than putting it anywhere else. I'll point out one last complication about equilibrium points: because the Earth is orbiting the Sun, the equilibrium point between the two is ALSO orbiting the Sun, with exactly the same orbital period. Like it or not, to maintain a stable position in space, you either have to do a lot of work, or orbit something. If you're interested, the fact that the Sun-Earth system is rotating leads to 4 other equilibrium points other than the one mentioned above (some of which are very non-intuitive); they're called the Lagrange points. These are great places to put satellites. Things get pretty weird around some of these points; it turns out you can actually orbit them (yes, an orbit around nothing) in a quasi-stable way. Anyway, there's lots of information out there if you want to learn more.