Is it true that debris can stay in orbit at the same height as space stations, while having a very large relative speed to the space stations?
Most LEO objects orbit in the same direction, namely the direction the Earth rotates. This is done to take advantage of the initial speed supplied by the Earth's rotation. So the main velocity difference would likely be due to different inclinations (i.e., not in the same plane) or elliptical vs. circular orbits.
Let's make it simple and assume that two objects, E and I, both have circular orbits at the same altitude, thus they have the same orbital speed. However, let us assume object I has an inclined orbit at some angle $\alpha$ to the equatorial plane and that they orbit in the same azimuthal direction.
Now the when we move into the frame of, say, the equatorial orbiting object the inclined object will appear to be coming from ahead of you. If the inclined orbit is from south-to-north(north-to-south), then object I would appear to be coming at you from the south(north) and from ahead of you. This is just from the vector subtraction, where:
$$
\mathbf{V}_{I} - \mathbf{V}_{E} = -\mathbf{V}_{rel} \tag{1}
$$
or
$$
\mathbf{V}_{E} = \mathbf{V}_{I} + \mathbf{V}_{rel} \tag{2}
$$
where $\lvert \mathbf{V}_{E} \rvert = \lvert \mathbf{V}_{I} \rvert$ we have defined:
$$
\begin{align}
\mathbf{V}_{E} & \sim V_{E} \ \hat{\mathbf{x}} \tag{3a} \\
\mathbf{V}_{I} & \sim V_{I} \left( \cos{\alpha} \ \hat{\mathbf{x}} + \sin{\alpha} \ \hat{\mathbf{y}} \right) \tag{3b}
\end{align}
$$
Note: I really should be doing this in spherical trigonometry, but let's only concern ourselves with the immediate region of interaction so we can take the limit as the radius of curvature goes to infinity.
As you can see, $\mathbf{V}_{rel}$ is given by:
$$
\mathbf{V}_{rel} = V_{E} \left[ \left( 1 - \cos{\alpha} \right) \hat{\mathbf{x}} - \sin{\alpha} \hat{\mathbf{y}} \right] \tag{4}
$$
which shows that $\lim_{\alpha \rightarrow 0} \mathbf{V}_{rel} = 0$, as expected.
The maximum of $\lvert \mathbf{V}_{rel} \rvert$ occurs in the limit as $\alpha \rightarrow \pi/2$, which is $\sim \sqrt{2} \ V_{E}$.
The typical orbital speed of a LEO object is ~7-8 km/s (or ~25,200-28,800 kph = ~15,700-17,900 mph), so the maximum impact speed is going to be ~10-11 km/s (or ~35,600-40,700 kph = ~22,100-25,300 mph).
So the short answer to your question is yes.
If the objects were orbiting in opposite directions, then the maximum of $\lvert \mathbf{V}_{rel} \rvert$ would increase, of course. Regardless, unless $\alpha \ll 1$, $\lvert \mathbf{V}_{rel} \rvert$ is going to be large for all intents and purposes.
Is it possible for the intact station to deorbit, simply by getting a little bit hit (it couldn't have been much since it was still mostly intact)?
I am going to guess no because of my response to the previous part. A space station has a great deal of linear and angular momentum. To exert enough force and torque to change those would require high impact speeds. Since most of the space station's outer hull is very thin, the end result would not be the entire object being "shoved" to a different altitude. Rather, the impacting object would probably just tear through the station and/or ablate on impact.
More Important Note
In the movie, the characters are able to see the objects moving towards them. If we assume that the objects are moving at, say, $\alpha \sim \pi/6$ to the space station's plane, then $\lvert \mathbf{V}_{rel} \rvert \sim \sqrt{2 - \sqrt{3}} V_{E}$. If we assume the same values as above for LEO orbits, then $\lvert \mathbf{V}_{rel} \rvert$ ~3.6-4.1 km/s (or ~13,000-14,900 kph = ~8,100-9,200 mph).
These values correspond to Mach ~ 10-12 at sea level. For comparison, the muzzle velocity of a bullet from a high powered rifle varies from Mach ~ 1.8-3.6, depending on the caliber and model.
I am inclined to think that were this situation to really occur, a human would not be able to see the incident objects unless they were very large because of the lighting conditions and the speed at which the objects were moving.
A more accurate portrayal would have shown parts of the space station just disappearing and/or exploding. There would not have been the nice, slow moving objects flying by that destroyed everything.
Update
When we look at the full range of muzzle velocities from a list of cartridges, we find handguns/pistols range from ~304–515 m/s and rifles range from ~600–1392 m/s. I plotted the relative speed between two orbiting objects as a function of inclination (i.e., Equation 4 above) shown in the following image.
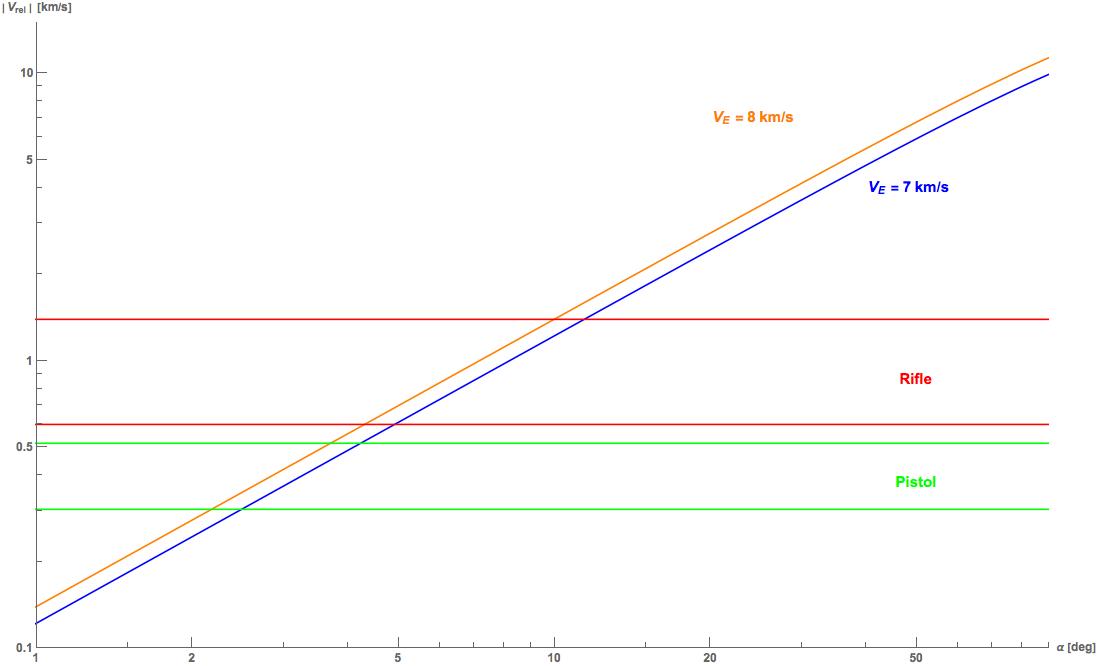
As shown, after even only ~3-4 degrees the speed differences exceed most handgun round muzzle speeds and after ~11 degrees nearly all civilian rifle rounds.

