87
$\begingroup$

My professor asked an interesting question at the end of the last class, but I can't figure out the answer. The question is this (recalled from memory):

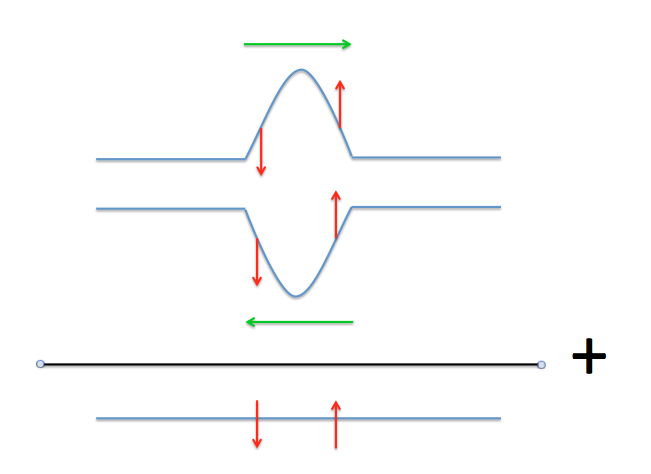
There are two travelling wave pulses moving in opposite directions along a rope with equal and opposite amplitudes. Then when the two wave pulses meet they destructively interfere and for that instant the rope is flat. Why do the waves continue after that point?

Here's a picture I found that illustrates the scenario

enter image description here

I know it's got to have something to do with the conservation laws, but I haven't been able to reason it out. So from what I understand waves propogate because the front of the wave is pulling the part of the rope in front of it upward and the back of the wave is pulling downward and the net effect is a pulse that propogates forward in the rope (is that right?). But then, to me, that means that if the rope is ever flat then nothing is pulling on anything else so the wave shouldn't start up again.

From a conservation perspective, I guess there's excess energy in the system and that's what keeps the waves moving, but then where's that extra energy when the waves cancel out? Is it just converted to some sort of potential energy?

This question is really vexing! :\

Improve this question
$\endgroup$
1

4 Answers 4

Reset to default
122
$\begingroup$

What you cannot see by drawing the picture is the velocity of the individual points of the string. Even if the string is flat at the moment of "cancellation", the string is still moving in that instant. It doesn't stop moving just because it looked flat for one instant. Your "extra" or "hidden" energy here is plain old kinetic energy.

Mathematically, the reason is that the wave-equation is second-order, hence requires both the momentary position of the string as well as the momentary velocity of each point on it to yield a unique solution.

Improve this answer
$\endgroup$
2
  • 1
    $\begingroup$ what about EM waves ? $\endgroup$
    – Manu de Hanoi
    Commented Apr 17, 2021 at 17:10
  • $\begingroup$ Pretty much the same deal. There are several ways to rearrange Maxwell's equations into wave equations that reveals the propagation of EM waves. These equations are 2nd order differential equations just like the 1D case except in 3D space. $\endgroup$
    – PersonWithName
    Commented Oct 8, 2021 at 7:00
70
$\begingroup$

ACuriousMind's excellent description was missing a picture. Here it is:

enter image description here

This clearly shows that for the wave moving to the right, the front is moving up and the rear is moving down. For the opposite wave traveling to the left, the front (now on the left) is moving down and the rear is moving up.

Summing them, you get a straight line with significant velocity.

Improve this answer
$\endgroup$
0
41
$\begingroup$

Just to complement the other excellent answers, here's an animation showing what two wave pulses with opposite amplitude passing through each other actually look like:

Animation of two opposite wave pulses colliding

You can clearly see that, at the instant when the string is momentarily flat, it's not stationary but rather moving quite rapidly, and thus will not stay flat for long.

(Obviously, the animation depicts an idealized string with perfectly linear wave propagation and zero dispersion, but the same qualitative behavior can indeed be observed in the real world e.g. in a flexible spring.)

Improve this answer
$\endgroup$
5
  • 2
    $\begingroup$ Fun further consideration: the point in the middle of the string never moves, so its displacement and velocity are both zero. Instead the pulse going right reappears from the velocity "left behind" by the pulse that went leftwards and got cancelled. $\endgroup$
    – jpa
    Commented Apr 4, 2016 at 7:05
  • $\begingroup$ @jpa It does have an angular velocity and acceleration. $\endgroup$
    – curiousdannii
    Commented Apr 4, 2016 at 11:21
  • 2
    $\begingroup$ @curiousdannii: jpa does have a point; the dynamics on either side of the midpoint would look exactly the same even if you fastened the midpoint to an immobile wall. (In fact, this is how one can show that a wave hitting a fixed boundary will produce an inverted reflection.) So we effectively have two equivalent descriptions of the same motion; one in which the waves pass through each other and combine linearly, and one in which they never interact except through the midpoint, which never moves. $\endgroup$
    – Ilmari Karonen
    Commented Apr 4, 2016 at 12:09
  • $\begingroup$ @IlmariKaronen If the center point was truly fastened in both degrees of freedom it would look exactly the same, as long as both waves had the same speed. But if they had different speeds then although they would still cancel each other out, they would not look like they had both reflected. $\endgroup$
    – curiousdannii
    Commented Apr 4, 2016 at 12:11
  • $\begingroup$ @curiousdannii I don't think angular velocity matters for "idealized" springs, though it will of course occur in real ones. Also, all waves in the same spring always have the same propagation speed. $\endgroup$
    – jpa
    Commented Apr 4, 2016 at 14:53
0
$\begingroup$

Tensions and forces are still in existance in the rope fibres and recreate the waves. if you firmly and solidly confined these forces at such a node, the waves could not pass through the mode but would reflect off either side of the node (now a fixed boundary) and a phase change would be observed. Just guessing could well be wrong?

Improve this answer
$\endgroup$

Not the answer you're looking for? Browse other questions tagged or ask your own question.