Why do we see virtually inverted image on concave side of spoon?

Question

As we know that a virtual image is always erect then why do we see a virtual inverted image in the concave side of a spoon.

Answer 1

The problem is that the image you obtain from a concave mirror you obtain a real image, and not a virtual one.

---

To understand why the real image coming from the reflection in a spoon is inverted, you have to consider the ideal case of a concave mirror, in particular (for the sake of simplicity) a spherical one.

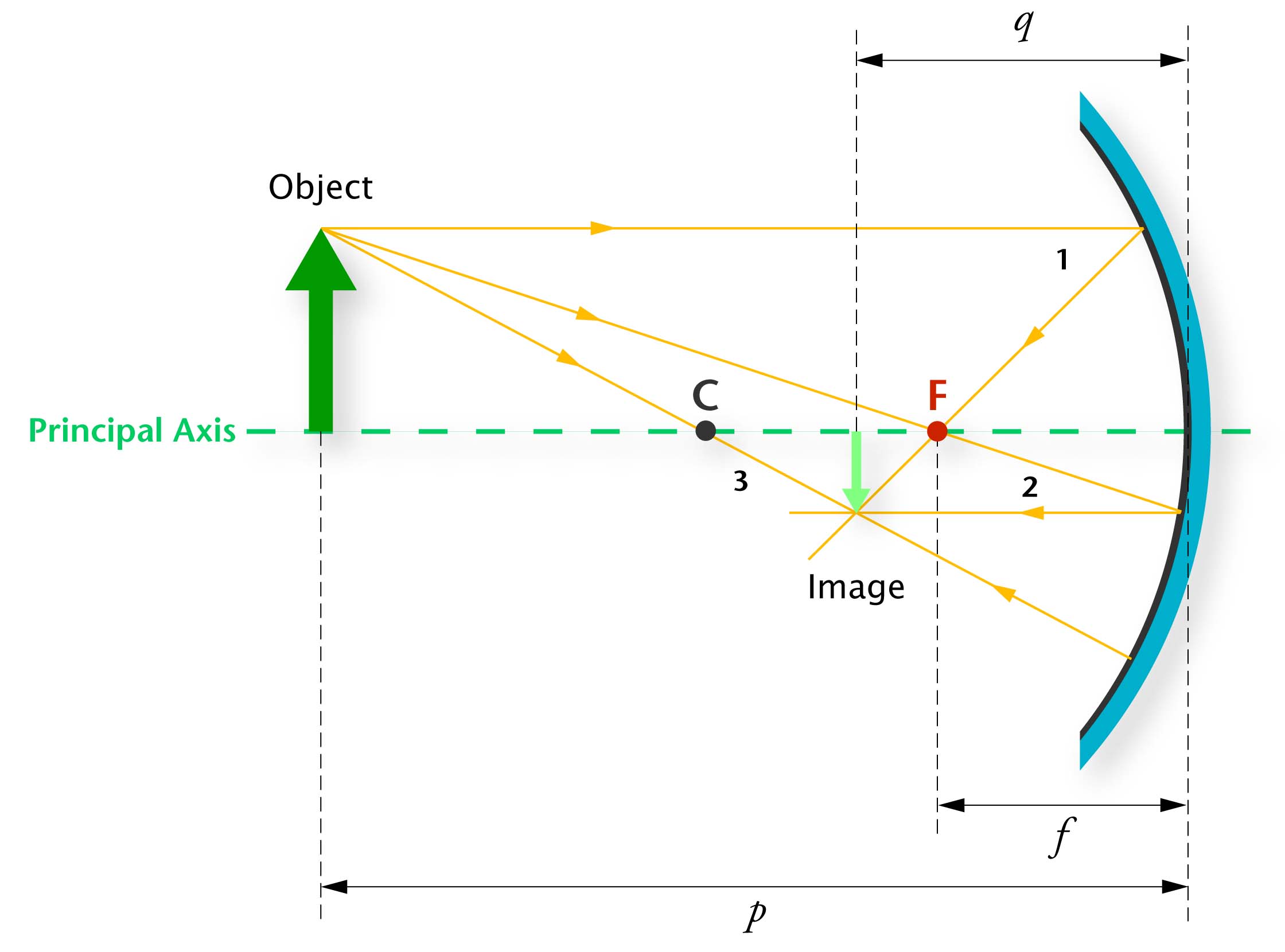
Suppose that the object you are observing, for example yourself, is the big green arrow. You can draw three different rays starting from the tip of the arrow:

• the ray passing through the center $C$ of the mirror;
• the ray passing through the focus $F$ of the mirror;
• the ray impinging on the mirror surface parallely to the optical axis.

Starting from the ray passing through the center $C$, you can see that it will imping perpendicularly on the surface of the mirror, thus being reflected along the same ray.

The ray passing through the focus $F$ will be reflected, by definition, parallely to the optical axis.

The ray impinging parallely to the optical axis will be reflected in the direction passing through the focal point $F$.

You obtain, therefore, an image that is exactly the small light green tip in figure. The important point, that addresses your question, is that this image is not a virtual image: it is a real image, since it is placed in the plane of convergence of the rays, thus it can be inverted. On the contrary, virtual images, that are formed when the rays outgoing from an optical device always diverge, will always be erected.

The last point to be addressed is how this image can be seen. The rays, after having converged again on the tip of the small light green arrow, diverges and they move toward the observer. It will be, therefore, the eyes of the observer that will take these diverging rays, will focus them on the retina and will show to the observer the image of the little arrow. In this specific case, the face of the observer is the big green arrow (even though in principle it is much farther from the center C than what is represented in this figure), the little arrow is the inverted image of yourself that you see in the spoon

Answer 2

It is as in any concave mirror. The radius of curvature of a spoon is so short that your face will always be further away than the center of curvature, but one can see the same thing with a shaving mirror if one goes a bit further away.

The classical ray diagrams do not explain very well what one sees. It is more enlightening to take one's own eye as the starting point for ray tracing (end points of the rays). As for example in this drawing in Conceptual Physics by Hewitt which explains the enlargement of a shaving mirror:

Now, if the eye moves further away, to the center of curvature, it will see itself filling the whole mirror. The rays back and forth are all at normal incidence.

Beyond that point, the angles will be reversed, and one will see an inverted image: the chin above one's eye, the hat below. See also this video with a large mirror: https://www.youtube.com/watch?v=K_p5UoD6ljg Pay attention to how the camera sees itself. (There are more interesting selfie videos with that mirror at the Exploratorium.)

Answer 3

Concave mirror produces real inverted image on a screen if you see erect behind the concave surface it is a virtual image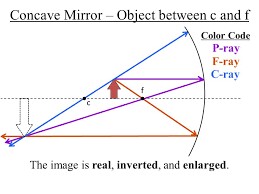]1