1
$\begingroup$

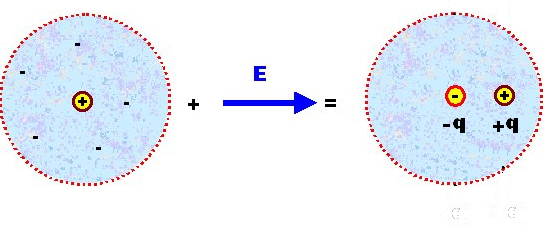
Will the electric field of an induced dipole in an insulator match the electric field inducing it but in the opposite direction? I have 2 counter theories:

Let's say I place an insulator (and let's make this one a plate as well, just because I want electric fields to be constant to simplify things) above a charged plate, and I want the electric field on the other side of the insulator plate. Would it be 0 because the electric field from the insulator has perfectly matched that of the charged plate?

  1. To me, it seems like it would, otherwise I don't understand why if there is a net force on the electrons in the insulator, they wouldn't keep on shifting until the electric fields did indeed match and cancel each other out.

  2. On the other hand, I really feel like for most materials it doesn't, since electric fields DO permeate insulators. But if the electric fields aren't canceling out, why don't the electrons inside the insulator keep on shifting until they DO cancel out?

  3. What about in a salt water solution for example. This is a conductor, so the electric field WILL eventually reach 0. However, what is the big difference in an insulator, if according to everywhere I've read, in an insulator dipoles are still created and the atoms "stretch", it's just the negative charges can't separate? If there is an applied electric field, won't the atoms keep on "stretching" until the field cancels itself out anyways?

Thank you!

Improve this question
$\endgroup$
8
  • $\begingroup$ The applied electric field is just not strong enough to separate electrons from its nucleus to the point where the now induced field would cancel out the applied field completely. Under normal conditions insulators cancel some of the applied field inside. Apply a strong enough field you will get a zero field inside. $\endgroup$
    – Global
    Commented Aug 14, 2018 at 11:34
  • $\begingroup$ But I don't understand. If it's not canceled out inside, then isn't is still strong enough to keep on creating the dipoles until the field DOES become 0? It's like (for an oversimplified example) I hang a block from a rope (the electrons being the block) and suspend it. If the rope (attraction to the atomic nucleus) is holding the block up against gravity, I would say the net force on that block is 0. If I have a bunch of blocks, the entire force on all of the blocks would also be 0. @Global $\endgroup$
    – joshuaronis
    Commented Aug 14, 2018 at 11:54
  • $\begingroup$ Actually, the applied E field keeps on stretching atoms till the applied force and the now induced force cancel. It just happens instantaneously, maybe of the order of $10^{-8} secs$. When you apply a very strong field to an insulator where the field is zero inside, the insulator becomes a conductor. $\endgroup$
    – Global
    Commented Aug 14, 2018 at 12:04
  • $\begingroup$ The conductors and the insulators, BOTH, experience the same type of force due to an applied external field i.e. like an insulator, a conductor do face a force on it. The expressions might be different but they are the same type of force. Does that make any sense or not? $\endgroup$
    – Global
    Commented Aug 14, 2018 at 13:47
  • $\begingroup$ Yea, I know its the same type of force, what I don't understand its why the insulator doesn't balance out the electric field inside of it once the molecules polarize $\endgroup$
    – joshuaronis
    Commented Aug 14, 2018 at 15:14

1 Answer 1

Reset to default
2
$\begingroup$

In an insulator (dielectric) there are no (very few) mobile charge carriers and so the external electric field distorts the electron shells around the nuclei so that the atom acts like a dipole.
All the electrons are still bound to nuclei but the "centre" of the electron cloud is no longer coincident with the position of the nucleus.

enter image description here

The induced dipoles are lined up and produce an induced electric field in opposition to the inducing electric field. The relative permittivity of the medium is a measure of how well the induced field opposes the external inducing field.
So the higher the permittivity the smaller is the electric field inside the insulator.

Under the influence of an external electric field the mobile charge carriers in a conductor rearrange themselves (the mobile charge carriers actually move within the lattice) so that the induced electric field that they produce is equal in magnitude and opposite in direction to the external inducing field with the result that there is no electric field inside the conductor - relative permittivity is infinite.

Improve this answer
$\endgroup$
3
  • $\begingroup$ But why don't these induced dipoles cancel out the electric field? It seems to me that they would, otherwise, the electron cloud would keep on shifting until some electrons become separated from the atom and mobile the entire thing becomes a conductor (as Global said in the comments to my question) , or until the electric field simply cancels out so that the cloud isn't experiencing any net forces on it anymore. Or maybe it is that the electric field DOES cancel out at a local level (in each atom, so as to not rip the cloud off), but not globally, in the entire insulator? @Global and Farcher $\endgroup$
    – joshuaronis
    Commented Aug 14, 2018 at 13:18
  • $\begingroup$ I have just found this answer which might help? physics.stackexchange.com/questions/329800/… $\endgroup$
    – Farcher
    Commented Aug 14, 2018 at 13:23
  • $\begingroup$ Thanks, Farcher, but that answer just kind of says it is, doesn't really explain why :( $\endgroup$
    – joshuaronis
    Commented Aug 14, 2018 at 13:33

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.