3
$\begingroup$

enter image description here

My teacher said that after switch is shifted (after very long time), $\phi_i = \phi_f$ $\implies i_oL = i3L \implies i = \dfrac{i_o}{3} $ where $i_o$ is $\dfrac{\varepsilon}{R}$

So the initial current in the circuit after switch is shifted is $\dfrac{i_o}{3}$

But, I really didn't understand why the flux should be conserved in this case i.e. why $\phi_i = \phi_f$. I would like to know about this concept and the reasons involved.

$\phi_i$ and $\phi_f$ are the total flux in both inductors immediately before and immediately after the switch is shifted?

Improve this question
$\endgroup$
9
  • $\begingroup$ @sammygerbil Sorry, by "new circuit", I meant that current can now flow through 2L as well. $\endgroup$
    – Archer
    Commented Jul 28, 2018 at 8:16
  • $\begingroup$ Your hand-drawn diagram in the chatroom is clearer. You have not said what $\phi_i, \phi_f$ are. I think you mean that these are the total flux in both inductors immediately before and immediately after the switch is closed? $\endgroup$
    – sammy gerbil
    Commented Jul 28, 2018 at 8:29
  • $\begingroup$ Yes @sammygerbil thats what I mean $\endgroup$
    – Archer
    Commented Jul 28, 2018 at 8:31
  • $\begingroup$ Sorry @sammygerbil I had posted the wrong diagram. $\endgroup$
    – Archer
    Commented Jul 28, 2018 at 8:36
  • $\begingroup$ It was a good diagram, except for the switch. If you changed the switch to look like this diagram, the other diagram would be ok. $\endgroup$
    – sammy gerbil
    Commented Jul 28, 2018 at 8:57

1 Answer 1

Reset to default
4
$\begingroup$

A better arrangement would be as follows the reason being that at no time will there be an open circuit as the switch position is changing?

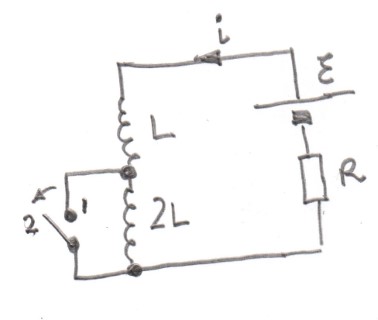
enter image description here

With the switch in position 1 a current flows though the switch but no current flow through inductor $2L$ as it is short circuited by the switch.

The right loop consisting of the two inductors, the cell and the resistor has a magnetic flux passing through it $\Phi_{\rm i} = L \,i_{\rm i}$

When the switch is moved to position 2 the magnetic flux linked with that loop cannot change instantaneously and so the right loop adjusts itself by having the having the same magnetic flux linked with it but now that magnetic flux is contributed by both inductors with $\Phi_{\rm f} = L \,i_{\rm f}+ 2L \,i_{\rm f}$.

You may wonder as to the origin of the magnetic flux linked with the circuit.
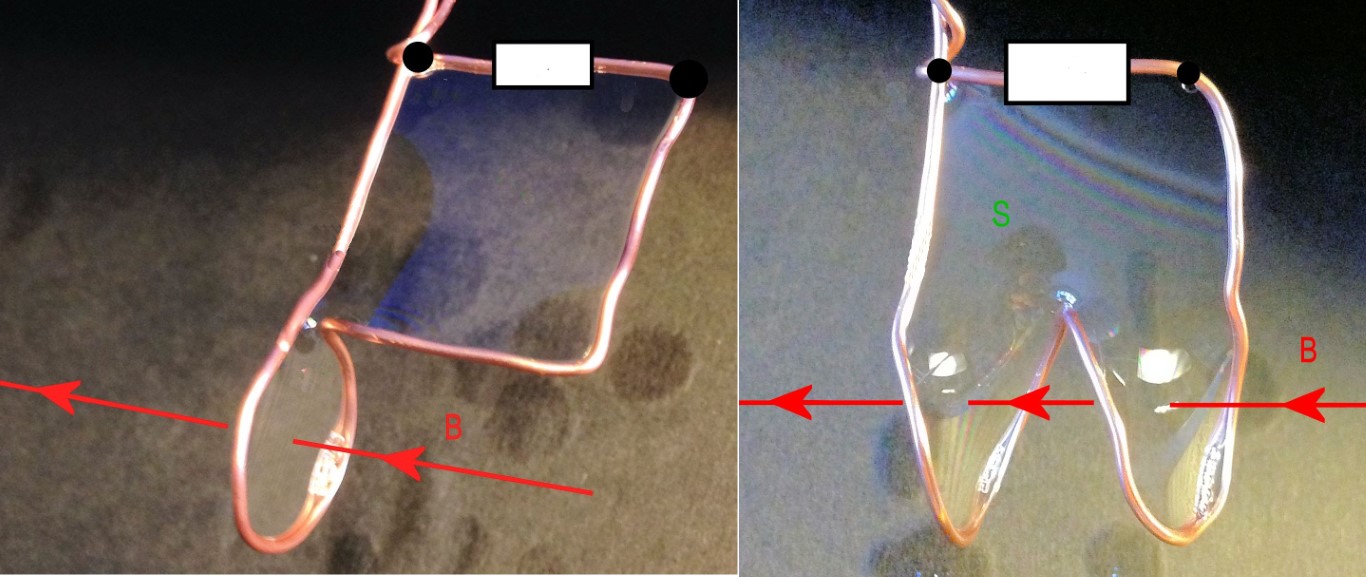
Some time ago I was trying to explain Faraday's law and why two turns produced double the flux linkage than one turn.
Being unable to draw a satisfactory sketch of the area through which the magnetic field was passing I hit on the idea of using a soap film to represent the area.

enter image description here

There is an equivalent circuit for capacitors which perhaps is intially easier to analyse?

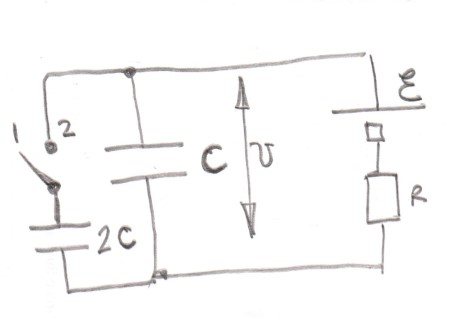
enter image description here

In this case it would be charge which is conserved and on moving the switch to position 2 the initial voltage across the two capacitors would drop to $\frac v 3$.

Note that in both case energy is not conserved

Improve this answer
$\endgroup$
3
  • 1
    $\begingroup$ I think this does not actually explain why the magnetic flux is conserved, whereas the stored magnetic energy is not conserved. $\endgroup$
    – sammy gerbil
    Commented Jul 28, 2018 at 13:11
  • 1
    $\begingroup$ @sammygerbil Magnetic flux cannot change instantaneously as this would induce an infinite emf in opposition to the change in magnetic flux? $\endgroup$
    – Farcher
    Commented Jul 28, 2018 at 16:22
  • 1
    $\begingroup$ Doesn't the instantaneous change of inductance of the circuit induce large (infinite?) potential differences of opposite polarity across the two inductors? What is the difference? (In both cases "instantaneous" is a relative term : the change happens with finite duration, however small, so the rate of change - and induced emf - is not actually infinite.) $\endgroup$
    – sammy gerbil
    Commented Jul 28, 2018 at 16:35

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.