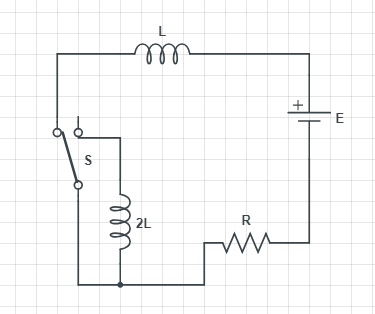
My teacher said that after switch is shifted (after very long time), $\phi_i = \phi_f$ $\implies i_oL = i3L \implies i = \dfrac{i_o}{3} $ where $i_o$ is $\dfrac{\varepsilon}{R}$
So the initial current in the circuit after switch is shifted is $\dfrac{i_o}{3}$
But, I really didn't understand why the flux should be conserved in this case i.e. why $\phi_i = \phi_f$. I would like to know about this concept and the reasons involved.
$\phi_i$ and $\phi_f$ are the total flux in both inductors immediately before and immediately after the switch is shifted?